nLab principal ideal domain

Definition

A ring $R$ is a principal ideal domain if

1. it is an integral domain (hence in particular a commutative ring)

2. every ideal in $R$ is a principal ideal.

Often pid is used as an abbreviation of “principal ideal domain”.

A pid can also be characterized as an integral domain wich possesses a Dedekind-Hasse norm, making very clear the fact that it is a generalization of an Euclidean domain.

Examples

• any field

• the ring $\mathbb{Z}$ of integers

• a polynomial ring with coefficients in a field (in fact, for any commutative ring $R$, the ring $R[x]$ is a pid if and only if $R$ is a field)

• a discrete valuation ring (for example, a ring of formal power series over a field)

• any Euclidean domain

• in the ring of entire holomorphic functions on $\mathbb{C}$ every finitely generated ideal is principal (Helmer 40), but the ring is only a Bézout domain.

That both the integers and the polynomial rings $\mathbb{F}_q[x]$ over finite fields are principal integral domains with finite group of units is one aspect of the close similarity between the two that is the topic of the function field analogy. That also the holomorphic functions on the complex plane form a Bézout domain may then be viewed as part of the further similarity that relates the previous two to topics such as geometric Langlands duality. See at function field analogy – table for more on this.

Properties

Lemma

In a pid $R$, an element $x$ is irreducible iff $(x)$ is a maximal ideal.

Proof

Suppose $x$ is irreducible and $a \notin (x)$. Then the ideal generated by $a, x$ is principal, say $(b)$. Then $x = b y$ and since $x$ is irreducible, one of $b, y$ is a unit; if $y$ is a unit then $(x) = (b) = (a, x)$ and thus $a \in (x)$, contradiction. So then $b$ must be a unit, i.e., $(b)$ is the improper ideal. Thus $(x)$ has no proper extension: $(x)$ is maximal.

For any ring $R$, if $(x)$ is maximal and $x = a b$ for a non-unit $a$, then the inclusion $(x) \subseteq (a)$ is an equality by maximality, so $a = x v$ for some $v$. Then $x = x v b$. In an integral domain we conclude $1 = v b$; thus $b$ is a unit.

Proposition

A pid is a Noetherian ring.

Proof

The union of an ascending chain of ideals $I_1 \subseteq I_2 \subseteq \ldots$ is an ideal $I$; if $I = (x)$, then $x$ belongs to one of the $I_n$, whereupon $I = I_n$.

Proposition

A PID is a unique factorization domain.

Proof

Working in classical logic, Proposition says that the reverse inclusion relation on the set of nonzero ideals is well-founded. Let $A$ be the subset of ideals $(a)$ that are products of finitely many (maybe zero!) maximal principal ideals. For any proper ideal $(x) \neq (0)$, if every $(t)$ properly containing $(x)$ can be factored into maximals, then so can $(x)$. (Spelling this out: either $(x)$ is maximal/irreducible, or factors as $(s)(t)$ where both $s$ and $t$ are non-units; $(s)$ and $(t)$ factor into maximals by hypothesis, and therefore so does $(x)$.) Thus $A$ is an inductive set, so by well-foundedness it contains every ideal $(x) \neq (0)$, i.e., $x$ can be factored into irreducibles.

For uniqueness of the factorization, we first remark that if $p$ is irreducible and $p|a b$, then $p|a$ or $p|b$. (For $R/(p)$ is a field and thus a fortiori an integral domain, so that if $a b \equiv 0 \; mod p$, then $a \equiv 0 \; mod p$ or $b \equiv 0 \; mod p$.) Thus if $p_1 p_2 \ldots p_m = q_1 q_2 \ldots q_n$ are two factorizations into irreducibles of the same element, then $p_1$ divides one of the irreducibles $q_i$, in which case $(p_1) = (q_i)$ and each is a unit times the other, meaning we can cancel $p_1$ on both sides and argue by induction.

Structure theory of modules

Free and projective modules

Theorem

If $R$ is a pid, then any submodule $M$ of a free module $F$ over $R$ is also free. (For the converse statement, see here.)

Proof

By freeness of $F$, there exists an isomorphism $F \cong \sum_{j \in J} R_j$, a coproduct of copies $R_j$ of $R$ (as a module over the ring $R$) indexed over a set $J$, which we assume well-ordered using the axiom of choice. Define submodules of $F$:

$$F_{\leq j} \coloneqq \sum_{i \leq j} R_i, \qquad F_{\lt j} \coloneqq \sum_{i \lt j} R_i\,.$$

Any element of $M \cap F_{\leq j}$ can be written uniquely as $(x, r)$ where $x \in F_{\lt j}$ and $r \in R_j$. Define a homomorphism

$$p_j \colon M \cap F_{\leq j} \to R$$

by $p_j((x, r)) = r$. The kernel of $p_j$ is $M \cap F_{\lt j}$, and we have an exact sequence

$$0 \to M \cap F_{\lt j} \to M \cap F_{\leq j} \to \im p_j \to 0$$

where $\im p_j$ is a submodule (i.e., an ideal) of $R$, hence generated by a single element $r_j$. Let $K \subseteq J$ consist of those $j$ such that $r_j \neq 0$, and for $k \in K$, choose $m_k$ such that $p_k(m_k) = r_k$. We claim that $\{m_k: k \in K\}$ forms a basis for $M$.

First we prove linear independence of $\{m_k\}$. Suppose $\sum_{i=1}^{n} a_i m_{k_i} = 0$, with $k_1 \lt k_2 \lt \ldots \lt k_n$. Applying $p_{k_n}$, we get $a_n p_{k_n}(m_{k_n}) = a_n r_{k_n} = 0$. Since $r_{k_n} \neq 0$, we have $a_n = 0$ (since we are working over a domain). The assertion now follows by induction.

Now we prove that the $m_k$ generate $M$. Assume otherwise, and let $j \in J$ be the least $j$ such that some $m \in M \cap F_{\leq j}$ cannot be written as a linear combination of the $m_k$, for $k \in K$. If $j \notin K$, then $m \in M \cap F_{\lt j}$, so that $m \in M \cap F_{\leq i}$ for some $i \lt j$, but this contradicts minimality of $j$. Therefore $j \in K$. Now, we have $p_j(m) = r \cdot r_j$ for some $r$; put $m' = m - r m_j$. Clearly

$$p_j(m') = p_j(m) - r \cdot p_j(m_j) = 0$$

and so $m' \in M \cap F_{\lt j}$. Thus $m' \in M \cap F_{\leq i}$ for some $i \lt j$. At the same time, $m'$ cannot be written as a linear combination of the $m_k$; again, this contradicts minimality of $j$. Thus the $m_k$ generate $M$, as claimed.

Since the integers $\mathbb{Z}$ form a pid, and abelian groups are the same as $\mathbb{Z}$-modules, we have

Corollary

(Dedekind) A subgroup of a free abelian group is also free abelian.

Remark

The analog of this statement for possibly non-abelian groups is the Nielsen-Schreier theorem.

Also, since projective modules are retracts of free modules, we have

Corollary

Projective modules over a pid are free. In particular, submodules of projective modules are projective.

Normal forms

Proposition

(matrices over principal ideal domains equivalent to Smith normal form)

For $R$ a commutative ring which is a principal ideal domain, every matrix $A \in Mat_{n \times m}(R)$ with entries in $R$ is matrix equivalent to a diagonal matrix filled up with zeros:

There exist invertible matrices $P \in Mat_{n \times n}(R)$ and $Q \in Mat_{m \times m}(R)$ such that the product matrix $P A Q$ is a diagonal matrix filled up with zeros:

$$P A Q \;=\;$$

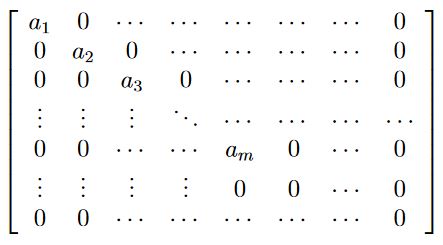
such that, moreover, each $a_i$ divides $a_{i+1}$.

For matrices with coefficients in the pid of integers see also

• Hermite normal form.

Torsion-free modules

Proposition

A finitely generated torsionfree module $M$ over a pid $R$ is free.

Proof

Let $S$ be a finite set of generators of $M$, and let $T \subseteq S$ be a maximal subset of linearly independent elements. (Unless $M = 0$, then $T$ has at least one element, because $M$ is torsionfree.) We claim that $M$ can be embedded as a submodule of the free module $F$ generated by $T$ (which in turn is the span of $T$ as a submodule $F \subseteq M$). By Theorem , it follows that $M$ is free.

Let $x_1, \ldots, x_n$ be the elements of $T$. It follows from maximality of $T$ that for any $m \in S - T$, there is a linear relation

$$r_m m + r_1 x_1 + \ldots + r_n x_n = 0$$

with $r_m \neq 0$. For each $m$ in the complement $S - T$, pick such an $r_m$, and form $r = \prod_{m \in S - T} r_m$. Then the image of the scalar multiplication $\lambda_r \colon M \to M$ factors through $F \subseteq M$, and $M \to \lambda_r(M)$ is monic because $M$ is torsionfree. This completes the proof.

Proposition

Let $R$ be a pid. Then an $R$-module $M$ is torsionfree if and only if it is flat.

Proof

Suppose $M$ is flat. Let $K$ be the field of fractions of $R$; since $R$ is a domain, we have a monic $R$-module map $R \to K$. By flatness, we have an induced monomorphism $M \cong R \otimes_R M \to K \otimes_R M$. For any nonzero $r \in R$, the naturality square

$$\array{ M & \to & K \otimes_R M \\ \mathllap{\lambda_r} \downarrow & & \downarrow \mathrlap{1 \otimes \lambda_r} \\ M & \to & K \otimes_R M }$$

commutes, and since the map $1 \otimes \lambda_r$ is multiplication by a non-zero scalar on a vector space, it follows that this map and therefore also $\lambda_r$ is monic, i.e., $M$ is torsionfree.

In the other direction, suppose $M$ is torsionfree. Any module is the filtered colimit over the system of finitely generated submodules and inclusions between them; in this case all the finitely generated submodules of $M$ are torsion-free and hence free, by Proposition . Thus $M$ is a filtered colimit of free modules; it is therefore flat by a standard result proved here.

Structure theory of finitely generated modules

structure theorem for finitely generated modules over a principal ideal domain

In constructive mathematics

In constructive mathematics, many important rings may fail to be principal ideal domains in the naïve sense; the notion of Bézout domain, in which only the finitely generated ideals are required to be principal, is better behaved.

For instance, the ring of integers is a principal ideal domain if and only if the law of excluded middle holds: In one direction, the usual proofs rely on being able to decide whether any particular integer belongs to the ideal or not. For the converse, let $\varphi$ be an arbitrary proposition. Consider the ideal $\{ x \in \mathbb{Z} | (x = 0) \vee \varphi \}$. By assumption, it is generated by some number $n$. Since the integers are discrete, it holds that $n = 0$ or $n \neq 0$. In the first case $\neg\varphi$ holds, in the second $\varphi$.

However, this ideal cannot be proved to be finitely generated either. If an ideal is generated by $n_1, \ldots, n_k$, then we may form their gcd one step at a time, which we can do algorithmically. Therefore, $\mathbb{Z}$ remains a Bézout domain.

On the other hand, we could try to modify the concept of principal ideal domain to recover a concept that is identical to the usual one in classical mathematics but also includes $\mathbb{Z}$. For instance, we could demand that every decidable ideal is principal, or (potentially more strongly) that any ideal generated by a decidable subset is principal. While these seem to work at first, they are too weak to prove that every PID is a Bézout domain, so we should try to think of something better.

Some authors have tried to define a principal ideal domain as a Noetherian Bézout domain, but it is unknown if this still coincides in constructive mathematics with the definition of principal ideal domain above.

See also

• unique factorization domain

• principal ideal domain

References

• O. Helmer, Divisibility properties of integral functions, Duke Math. J. 6 (1940), 345-356.

• Wikipedia, Principal ideal domain

• Eric Wofsey, Principal Ideal Domains, (written for Mathcamp 2009) pdf

• Henri Lombardi, Claude Quitté (2010): Commutative algebra: Constructive methods (Finite projective modules) Translated by Tania K. Roblo, Springer (2015) (doi:10.1007/978-94-017-9944-7, pdf)