nLab t-structure
Context
Stable Homotopy theory
Ingredients
Contents
Contents
Definition
Originally, t-structures were defined
These typically arise as homotopy categories of t-structures
On triangulated categories
Definition
(t-structure on a triangulated category)
Let be a triangulated category. A t-structure on is a pair of strictly full subcategories
such that
-
for all and the hom object is the zero object: ;
-
the subcategories are closed under suspension/desuspension: and .
-
For all objects there is a fiber sequence (i.e. an exact triangle) with and .
On stable -categories
Definition
(t-structure in a stable -category)
A t-structure on a stable (∞,1)-category is a t-structure in the above sense (Def. ) on its underlying homotopy category (which is triangulated, see there).
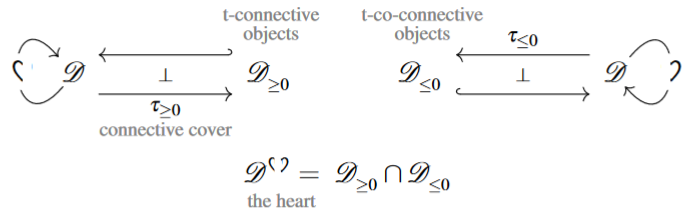
Therefore, a t-structure on a stable -category is a system of full sub-(∞,1)-categories , , .
Proposition
-
the are reflective sub-(∞,1)-categories
-
the are coreflective sub-(∞,1)-categories;
Properties
General
Proposition
The heart of a stable -category is an abelian category.
(BBD 82, Higher Algebra, remark 1.2.1.12, FL16, Ex. 4.1 and FLM19, §3.1)
Relation to spectral sequences
If the heart (Def. ) of a t-structure on a stable (∞,1)-category with sequential limits is an abelian category, then the spectral sequence of a filtered stable homotopy type converges (see there).
Relation to normal torsion theories
In the (∞,1)-category theory, -structures arise as torsion/torsionfree classes associated with suitable factorization systems on stable ∞-categories .
-
In stable ∞-category-theory, the relevant sub-(∞,1)-categories are closed under de/suspension simply because they are (co-)reflective, arising from co/reflective factorization systems on .
-
A bireflective factorization system on a -category consists of a factorization system where both classes satisfy the two-out-of-three property.
-
A bireflective factorization system on a stable -category is called normal if the diagram obtained from the reflection and the coreflection (where the category is obtained as under the adjunction described at reflective factorization system and in CHK85; see also FL16, §1.1) is exact, meaning that the square in
is a fiber sequence for any object ; see FL16, Def 3.5 and Prop. 3.10 for equivalent conditions for normality.
Remark
CHK85 established a hierarchy between the three notions of simple, semi-exact and normal factorization system: in the setting of stable -category the three notions turn out to be equivalent: see FL16, Thm 3.11.
Proposition
There is a bijective correspondence between the class of -structures and the class of normal torsion theories on a stable -category , induced by the following correspondence:
-
On the one side, given a normal, bireflective factorization system on we define the two classes of a -structure to be the torsion and torsionfree classes associated to the factorization .
-
On the other side, given a -structure on we set
This is FL16, Theorem 3.13
Proposition
There is a natural monotone action of the group of integers on the class (now confused with the class of normal torsion theories on ) given by the suspension functor: goes to .
This correspondence leads to study families of -structures ; more precisely, we are led to study -equivariant multiple factorization systems .
Proposition
Let and correspond each other under the above bijection (Prop. ); then the following conditions are equivalent:
-
, i.e. ;
-
is a stable -category;
-
the class is closed under pullback.
In each of these cases, we say that or is stable.
This is FLM19, Theorem 6.3
This results allows us to recognize -structures with stable classes precisely as those which are fixed in the natural -action on .
Two “extremal” choices of -chains of -structures draw a connection between two apparently separated constructions in the theory of derived categories: Harder-Narashiman filtrations and semiorthogonal decompositions on triangulated categories: we adopt the shorthand to denote the tuple , each of the being a -structure on , and we denote similarly . Then
- In the stable case the tuple is endowed with a (monotone) -action, and the map is equivariant with respect to this action; the absence of nontrivial -actions on forces each to be stable.
- In the orbit case we consider an infinite family of -structures on , obtained as the orbit of a fixed with respect to the natural -action.
Towers
The HN-filtration induced by a -structure and the factorization induced by a semiorthogonal decomposition on both are the byproduct of the tower associated to a tuple :
Examples
The archetypical and historically motivating example (cf. Gelfand & Manin (1996), IV.4 §1) is the following:
Example
(canonical t-structure on the derived category of an abelian category)
For an abelian category, its unbounded derived category
-
carries a t-structure (Def. ) for which (rep. ) is the full subcategory of objects presented by chain complexes whose chain homology-groups are trivial in degrees (resp. );
-
whose heart (Def. ) is equivalent to (embedded as the chain complexes which are concentrated in degree 0).
(eg. Gelfand & Manin (1996), IV.4 §3)
Example
(canonical t-structure on spectra)
The stable (infinity,1)-category of spectra, , carries a canonical t-structure for which
-
is the sub-category of connective spectra, with the connective cover-construction.
-
…
(e.g. Lurie, Higher Algebr, pp. 150)
Related concepts
References
the notion is due to
-
Alexander Beilinson, Joseph Bernstein, Pierre Deligne, Faisceaux pervers, Astérisque 100 (1982) [ISBN:978-2-85629-878-7, pdf, MR86g:32015]
(otherwise introducing perverse sheaves)
Further development:
-
Sergei Gelfand, Yuri Manin, Section IV.4 of: Methods of homological algebra, transl. from the 1988 Russian (Nauka Publ.) original, Springer (1996, 2002) [doi:10.1007/978-3-662-12492-5]
-
Donu Arapura, Triangulated categories and -structures [pdf]
-
Dan Abramovich, Alexander Polishchuk, Sheaves of t-structures and valuative criteria for stable complexes, J. reine angew. Math. 590 (2006) 89-130 [arXiv:math/0309435, doi:10.1515/CRELLE.2006.005]
-
A. L. Gorodentsev, S. A. Kuleshov, A. N. Rudakov, t-stabilities and t-structures on triangulated categories, Izv. Ross. Akad. Nauk Ser. Mat. 68 (2004), no. 4, 117-150
-
Alexander Polishchuk, Constant families of t-structures on derived categories of coherent sheaves, Moscow Math. J. 7 (2007) 109-134 [arXiv:math/0606013]
-
John Collins, Alexander Polishchuk, Gluing stability conditions [arxiv/0902.0323]
- Jacob Lurie, Section 1.2.1 in: Higher Algebra
On reflective factorization systems:
-
C. Cassidy, M. Hébert, Max Kelly, Reflective subcategories, localizations, and factorization systems, J. Austral. Math Soc. (Series A) 38 (1985) 287-329 [doi:10.1017/S1446788700023624]
-
Jiri Rosicky, Walter Tholen, Factorization, Fibration and Torsion, Journal of Homotopy and Related Structures, Vol. 2(2007), No. 2, pp. 295-314 [arXiv:0801.0063, publisher]
and on normal torsion theories in stable -categories:
-
Domenico Fiorenza, Fosco Loregian, -Structures are normal torsion theories, Appl Categor Struct 24 (2016) 181–208 [arxiv:1408.7003, doi:10.1007/s10485-015-9393-z]
-
Domenico Fiorenza, Fosco Loregian, Giovanni Marchetti, Hearts and towers in stable -categories, J. Homotopy Relat. Struct. 14 (2019) 993–1042 [arXiv:1501.04658, doi:10.1007/s40062-019-00237-0]
-
Fosco Loregian, Simone Virili Triangulated factorization systems and t-structures, Journal of Algebra 550 (2020) 219-241 [doi:10.1016/j.jalgebra.2019.12.021]
Last revised on April 20, 2023 at 06:33:40. See the history of this page for a list of all contributions to it.