nLab Smith normal form
Context
Linear algebra
homotopy theory, (∞,1)-category theory, homotopy type theory
flavors: stable, equivariant, rational, p-adic, proper, geometric, cohesive, directed…
models: topological, simplicial, localic, …
see also algebraic topology
Introductions
Definitions
Paths and cylinders
Homotopy groups
Basic facts
Theorems
Algebra
Algebraic theories
Algebras and modules
Higher algebras
-
symmetric monoidal (∞,1)-category of spectra
Model category presentations
Geometry on formal duals of algebras
Theorems
Contents
Statement
Proposition
(matrices over principal ideal domains equivalent to Smith normal form)
For a commutative ring which is a principal ideal domain (for instance the integers), every matrix with entries in is matrix equivalent to a diagonal matrix filled up with zeros:
There exist invertible matrices and such that the product matrix is of the following form:
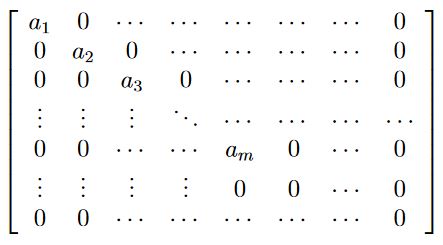
such that, moreover, each divides .
Related concepts
References
The results is named after
- H.J.S. Smith, On systems of linear indeterminate equations and congruences Philos. Trans. Royal Soc. London cli, 293-326. Reprinted in The Collected Mathematical Papers of Henry John Stephen Smith, Volume 1. New York: Chelsea (1965)
Lecture notes include
-
Patrick Morandi, The Smith Normal Form of a Matrix, 2005 (pdf)
-
Sam Evans, Smith normal form over the integers (pdf)
-
Bill Casselman: Hermite and Smith forms (2011) [pdf, pdf]
-
George Havas, Leon Sterling, Integer matrices and abelian groups (pdf, doi:10.1007/3-540-09519-5_94)
-
George Havas, Bohdan Majewski, Integer matrices and diagonalization, J. Symbolic Computation (1997) 24, 399-408 (pdf)
See also
- Wikipedia, Smith normal form
Last revised on March 22, 2025 at 17:20:44. See the history of this page for a list of all contributions to it.