nLab Fredholm operator

Definition

Definition

A bounded linear operator $F \colon B_1\to B_2$ between Banach spaces is Fredholm if it has finite dimensional kernel and finite dimensional cokernel.

(e.g. Murphy 1990 p. 23)

Remark

Some (older) text may also require that the image of $F$ be closed, but that is in fact implied by the cokernel of $F$ having finite dimension (cf. Prop. below). Moreover, some (older) texts do not require $F$ to be bounded, but just ask that it be closed with dense domain of definition (e.g. Schechter 1967 §1).

Definition

The difference between the dimensions of the kernel and the cokernel of a Fredholm operator $F$ is called its index (the Fredholm index)

$$\begin{array}{ccl} ind F &\coloneqq& dim (ker F) - dim (coker F) \\ &=& dim (ker F) - codim (im F) \mathrlap{\,.} \end{array}$$

Examples

• Elliptic operators on compact manifolds are naturally Fredholm, when understood between the appropriate Sobolev spaces.

• charged vacua of free Dirac field in Coulomb background are characterized by Fredholm operators

Properties

General

Proposition

The image (range) of a Fredholm operator is closed.

(cf. Atiyah 1967 p. 153-4, Murphy 1990 Thm. 1.4.7)

Proposition

The topological subspace $Fred(B_1,B_2)\subset B(B_1,B_2)$ of Fredholm operators (in the space of bounded linear operators equipped with the norm topology) is open.

On this subspace with its subspace topology, the Fredholm index (Def. ) is a continuous map.

(Murphy 1990 Thm. 1.4.17)

Remark

In other words, Prop. says that given a Fredholm operator $F$ then there exists a real number $\epsilon \gt 0$ such that every bounded linear operator $G$ with operator norm ${\Vert G - F \Vert} \lt \epsilon$ is itself Fredholm of the same index.

Proposition

For Banach spaces $B_1$, $B_2$, $B_3$ and Fredholm operators $F_1 \colon B_1 \longrightarrow B_2$ and $F_2 \colon B_2 \longrightarrow B_3$

1. the composite $F_2 \,\circ\, F_1 \colon B_1 \longrightarrow B_3$ is Fredholm

2. with index (Def. ) the sum of the indices of the factors:

   $$ind(F_2\circ F_1) \;=\; ind(F_1) + ind(F_2) \,.$$

(Murphy 1990 Thm. 1.4.8)

Remark

In other words, Prop. says that Banach spaces with Fredholm operators between them form a category on which the Fredholm index is a functor to the delooping groupoid of the integers; hence the endomorphic Fredholm operators $F \colon B \longrightarrow B$ form a monoid with the Fredholm index being a homomorphism of monoids.

Via parametrices

An equivalent characterization of Fredholm operators is the following:

Definition

A parametrix of a bounded linear operator $F \colon \mathcal{H}_1 \to \mathcal{H}_2$ is a reverse bounded operator $P \colon \mathcal{H}_2 \to \mathcal{H}_1$ which is an “inverse up to compact operators”, i.e. such that $F \circ P - id_{\mathcal{H}_2}$ and $P \circ F - id_{\mathcal{H}_1}$ are both compact operators.

Proposition

(Atkinson's theorem)
A bounded linear operator $F \colon B_1\to B_2$ between Banach spaces is Fredholm, def. , precisely if it admits a parametrix, def. .

(cf. Murphy 1990 Thm. 1.4.16)

Relation to topological K-theory

Proposition

(Atiyah-Jänich theorem)
The space of Fredholm operators $Fred(\mathscr{H})$ on a (countably infinite-dimensional, separable, complex) Hilbert space $\mathscr{H}$ is a classifying space for topological K-theory $K(-)$:

For $X$ a compact Hausdorff space, the homotopy classes of continuous maps from $X$ to $Fred(\mathscr{H})$ are in natural bijection with $K(X)$

$$\pi_0 \, Map\big( X, \, Fred(\mathscr{H}) \big) \xrightarrow[\sim]{ind_X} K(X) \,,$$

where $ind_X$ is an $X$-parameterized enhancement of the Fredholm index.

Several variants of the ordinary space of Fredholm operators retain the same homotopy type and hence all serves as classifying spaces for topological K-theory, but differ in further properties they have, cf. Atiyah & Segal 2004 §3.

A definition which makes a good classifying space also for twisted K-theory and equivariant K-theory is the following:

Definition

Given any $C_2$-graded infinite-dimensional (separable complex) Hilbert space $\mathscr{H} \simeq \mathscr{H}_+ \oplus \mathscr{H}_i$, write $Fred^{(0)}(\mathscr{H})$ for the set of bounded linear operators $F \,\colon\, \mathscr{H} \to \mathscr{H}$ which are

1. self-adjoint: $F^\dagger = F$

2. odd-graded: $F(\mathscr{H}_{\pm}) \subset \mathscr{H}_{\mp}$,

3. idempotent up to compact operators: $F^2 - Id \,\in\, \mathcal{K}(\mathscr{H})$,

equipped with the topology of the topological subspace, via

$$F \,\mapsto\, \big(F ,\, F^2 - id\big) \,,$$

of the product space of the spaces of

1. bounded linear operators, $\mathcal{B}$, equipped with the compact-open topology and

2. compact operators, $\mathcal{K}$, equipped with the norm topology:

(This is due to Atiyah & Segal 2004 Def. 3.2, following Atiyah & Singer 1969 p 7, see also Freed, Hopkins & Teleman 2011 Def. A.39.)

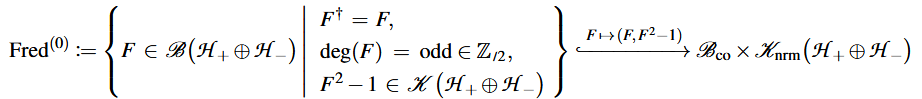
Remark

In view of Def. and Prop. , the condition $F^2 - id \in \mathcal{K}$ in Def. asserts that $F$ not just has a parametrix, but is its own parametrix. Or rather, together with the condition that $F$ is odd, hence $F = F_+ + F_-$ with $F_\pm \colon \mathscr{H}_{\pm} \to \mathscr{H}_{\mp}$, it says that $F_+$ and $F_-$ are parametrices of each other.

Indeed the Fredholm index map on $Fred^{(0)}(\mathscr{H})$ assigns the Fredholm index (Def. ) of one of these components (say $F_+$). (This follows by tracing through the equivalences indicated in AS04 §3.)

Finally, together with the grading, the condition $F^\ast = F$ implies that in fact $F_\pm = (F_\mp)^\ast$, whence the index is actually just (the dimension of) the kernel of $F$, but regarded as (the dimension of) a virtual vector space.

Self-adjoint Fredholm operators

General

The following is a digest of Booß-Bavnbek & Wojciechowski 1993.

All linear operators in the following act on a given separable countably infinite-dimensional complex Hilbert space.

Consider and denote:

• $\mathcal{B}$ — the space of bounded operators,

• $\mathcal{K} \subset \mathcal{B}$ — the subspace of compact operators,

• $\mathcal{C} \coloneqq \mathcal{B}/\mathcal{K}$ — the Calkin algebra regarded as a $C^\ast$-algebra,

• $\mathbf{p} \colon \mathcal{B} \twoheadrightarrow \mathcal{C}$ — the quotient coprojection,

• $\mathcal{G} \coloneqq \mathcal{C}^\times = GL(\mathcal{C})$ — the group of units of the Calkin algebra,

• $\widehat{\mathcal{G}} \subset \mathcal{G}$ — the subgroup of self-adjoint elements,

• $\widehat{\mathcal{G}}_{\pm} \subset \widehat{\mathcal{G}}$ — the subgroup of elements with purely positibe/negative essential spectrum,

• $\widehat{\mathcal{G}}_{\ast} \subset \widehat{\mathcal{G}}$ — the remaining subspace, of elements whose essential spectrum is both positive and negative,

• $G \coloneqq \mathrm{U}(\mathcal{C}) \subset GL(\mathcal{C})$ — the unitary group of the Calkin algebra,

• $\widehat{G} \coloneqq \widehat{\mathcal{G}} \cap G$ — the group of self-adjoint unitary elements of the Calkin algebra

• $\widehat{G}_{\pm} \coloneqq \widehat{\mathcal{G}}_{\pm} \cap G$ — the group of self-adjoint unitary elements of the Calkin algebra with purely positive/negative essential spectrum,

• $\widehat{G}_{\ast} \coloneqq \widehat{\mathcal{G}}_{\ast} \cap G$ — the group of self-adjoint unitary elements of the Calkin algebra with both positive and negative essential spectrum,

• $\mathcal{F} \simeq \mathbf{p}^{-1}(\mathcal{G})$ — the space of Fredholm operators,

• $\widehat{\mathcal{F}} \subset \mathcal{F}$ — the subspace of self-adjoint Fredholm operators,

• $\widehat{\mathcal{F}}_{\pm} \subset \widehat{\mathcal{F}}$ — the subspace of operators with purely positive/negative essential spectrum,

• $\widehat{\mathcal{F}}_{\ast} \subset \widehat{\mathcal{F}}$ — the remaining subspace, that of operators with both positive and negative essential spectrum,

Proposition

We have homeomorphisms:

$$\begin{aligned} \widehat{\mathcal{G}} & \;\simeq\; \widehat{\mathcal{G}}_+ \sqcup \widehat{\mathcal{G}}_- \sqcup \widehat{\mathcal{G}}_\ast \\ \widehat{\mathcal{F}} & \;\simeq\; \widehat{\mathcal{F}}_+ \sqcup \widehat{\mathcal{F}}_- \sqcup \widehat{\mathcal{F}}_\ast \\ \widehat{G}_\pm & \;\simeq\; \{ \pm id \} \\ \widehat{G}_\ast & \;\simeq\; \big\{ g \in \mathcal{C} \;\Big\vert\; g^\ast = g ,\; g^2 = id ,\; g \neq \pm id \big\} \\ & \;\simeq\; \big\{ g \in \mathcal{C} \;\Big\vert\; g^\ast = g ,\; spec(g) = \{+1, -1\} \big\} \\ & \;\simeq\; \underset{ Gr(\mathcal{C}) }{ \underbrace{ \big\{ p \in \mathcal{C} \,\big\vert\, p^2 = p = p^\ast \neq 0, id \big\} }} \end{aligned}$$

and homotopy equivalences:

$$\begin{aligned} \widehat{\mathcal{G}}_{\pm} & \;\sim\; \widehat{G}_{\pm} \;\sim\; \ast \\ \widehat{\mathcal{F}}_{\pm} & \;\sim\; \mathbf{p}^{-1}\big(\widehat{\mathcal{G}}_{\pm}\big) \;\sim\; \ast \\ \widehat{\mathcal{F}}_{\ast} & \;\sim\; \mathbf{p}^{-1}\big(\widehat{\mathcal{G}}_{\ast}\big) \\ \mathbf{p} \;\colon\; \widehat{\mathcal{F}}_\ast & \;\sim\; \widehat{\mathcal{G}}_\ast \;\sim\; \widehat{G}_\ast \end{aligned}$$

For $j_0 \in \widehat{G}_\ast$ any base point, the conjugation action map

$$\begin{array}{c} G &\overset{}{\longrightarrow}& \widehat{G}_\ast \\ u &\mapsto& u \circ j_0 \circ u^{-1} \end{array}$$

is a fiber bundle (in fact a principal bundle) with typical fiber $G \times G$.

(Booß-Bavnbek & Wojciechowski 1993, following Atiyah & Singer 1969, see also DSBW23 section 8.3)

The exponential map

Recall the notation $\widehat{\mathcal{F}}_\ast$ (from above) for the space of self-adjoint Fredholm operators with both positive and negative essential spectrum.

Proposition

The further subspace of self-adjoint Fredholm operators

(1)$$\widehat{\mathrm{F}}_\ast \;\coloneqq\; \Big\{ f \in \widehat{\mathcal{F}}_\ast \;\Big\vert\; spec_{ess}(f) = \{+1, -1\} ,\, \vert f \vert = 1 \Big\} \hookrightarrow \widehat{\mathcal{F}}_\ast$$

(whose essential spectrum is not just positive and negative but actually concentrated at $+1$ and $-1$, and whose operator norm is unity) is a deformation retract.

(Atiyah & Singer 1969 below (2.5))

Proposition

The exponential map

$$\exp\big(\pi \mathrm{i} (-) \big) \;\colon\; \widehat{\mathrm{F}}_\ast \longrightarrow - \Big\{ U \in \mathrm{U}(\mathscr{H}) \,\Big\vert\, U - id \in \mathcal{K}(\mathscr{H}) \Big\}$$

(from (1) to the image under negation of the unitary Fredholm group) is a homotopy equivalence.

(AS69 Prop. 3.3)

Observe that if $f \in \widehat{\mathrm{F}}_\ast$ satisfies $f^2 = 1$, hence if not just its essential spectrum but the actual spectrum is $\{+1,-1\}$, then (by Euler's formula)

$$\exp\big( \pi \mathrm{i} f \big) \;=\; cos(\pi)\cdot id + sin(\pi) \cdot f \;=\; -id \,.$$

Proposition

The subspace

(2)$$\widehat{\mathrm{F}}{}_\ast^0 \;\coloneqq\; \Big\{ f \in \widehat{\mathcal{F}}_\ast \;\Big\vert\; spec(f) = \{+1, -1\} ,\, \vert f \vert = 1 \Big\} \hookrightarrow \widehat{\mathrm{F}}_\ast$$

is contractible and its inclusio is a Hurewicz cofibration.

Proof

For the first statement: These operators are equivalently choices of direct sum decompositions $\mathscr{H} \simeq \mathscr{H} \oplus \mathscr{H}$. The space of these is the Grassmannian $\mathrm{U}(\mathscr{H})/\big( \mathrm{U}(\mathscr{H}) \times \mathrm{U}(\mathscr{H}) \big)$ — but U(ℋ) is contractible (Kuiper's theorem).

For the second statement: Since all spaces of operators in question are metric spaces (via the operator norm) they are perfectly normal Hausdorff spaces. Moreover, $\widehat{\mathrm{F}}{}_\ast^0 \subset \widehat{\mathrm{F}}{}_\ast$ is a closed subspace (being the preimage of $\{0\}$ under the map $\widehat{\mathrm{F}}_\ast \to \mathcal{B}(\mathscr{H}) \colon f \mapsto f^2 - Id$). Therefore it is sufficient (by this Prop.) to see that the inclusion is a strong deformation retract of a neighbourhood (this Def.). That neighbourhood may be taken to be the invertible operators among $\widehat{\mathrm{F}}_\ast$, and the retraction may then be given by functional calculus, shifting all points in the spectrum to their sign in $\pm 1$.

Hence we can sharpen Prop. to:

Corollary

The exponential map

$$\exp\big(\pi \mathrm{i} (-) \big) \;\colon\; \widehat{\mathrm{F}}_\ast \big/ \widehat{\mathrm{F}}{}_\ast^0 \longrightarrow - \Big\{ U \in \mathrm{U}(\mathscr{H}) \,\Big\vert\, U - id \in \mathcal{K}(\mathscr{H}) \Big\}$$

is a homotopy equivalence.

Example

(Fredholm representative of $\pi_3(KU_1)$)
Regarding

• $S^3 \simeq D^3/\partial D^3$ — the 3-sphere as the one-point compactification of the closed 3-ball,

• $D^3 \simeq D(\mathbb{H}_{im})$ — the closed 3-ball as the closed unit ball in the space of imaginary quaternions,

• $\mathbb{H}_{im} \hookrightarrow Mat^{herm}_{2 \times 2}(\mathbb{C})$ — via the Pauli matrices $\{\sigma_i\}_{i = 1}^3$,

• $Mat_{2 \times 2}(\mathbb{C}) \hookrightarrow \mathcal{F}$ by forming the Hilbert space direct sum $X \mapsto X \oplus \bigoplus_{n \in \mathbb{N}} diag(+1,-1)$,

then Cor. implies that we have a map

$$\begin{array}{ccccc} D^3 / \partial D^3 &\overset{}{\longrightarrow}& \widehat{\mathrm{F}}_\ast \big/ \widehat{\mathrm{F}}{}_\ast^0 &\overset{ exp(\pi \mathrm{i} (-)) }{\longrightarrow}& - C \\ x &\mapsto& \sigma \cdot x \mathrlap{\,.} \end{array}$$

But the total map now is now essentially just the exponential map from the unit ball in the Lie algebra $\mathfrak{su}(2)$ to the Lie group SU(2) and as such represents a generator of

$$\pi_3\big(C\big) = \mathbb{Z} \,.$$

This construction (in consequence of Atiyah & Singer 1969 Prop. 3.3) follows SS26 §4.3.3, completing a suggestion by Witten 2001 p. 6-8 following Hořava 1998 (3.7, 3.17).

Generalizations

Fredholm operators generalize to Fredholm complexes. A finite chain complex

$$0 \to C_0 \overset{d_0}{\longrightarrow} C_1 \overset{d_1}{\longrightarrow} C_2 \longrightarrow \cdots \longrightarrow C_n \to 0$$

of Banach spaces and bounded linear operators between them is said to be a Fredholm complex if the images $d_i$ are closed and the chain homology of the complex is finite dimensional. The Euler characteristic (the alternative sum of the dimensions of the homology groups) is then called the index of the Fredholm complex. Each Fredholm operator can be considered as a Fredholm complex concentrated at zero. Each Fredholm complex produces a Fredholm operator from the sum of the even- to the sum of the odd-numbered spaces in the complex.

One can consider Fredholm almost complexes, where $d_i \circ d_{i-1}$ is not zero but the image of that operator is compact. Out of every Fredholm almost complex one can make a complex by correcting the differentials by compact perturbation terms. Elliptic complexes give examples of Fredholm complexes.

Related concepts

• Fredholm module

• Fredholm determinant

• Fredholm group

• Dirac operator

• Toeplitz operator

• topological K-theory, KK-theory

• Atiyah-Jänich theorem

• index theory

References

General

The concept of Fredholm operators originates with and is named after:

• Ivar Fredholm: Sur une classe d’équations fonctionnelles, Acta Math. 27 (1903) [doi:10.1007/BF02421317, euclid:10.1007/BF02421317]

Textbook accounts:

• Ronald G. Douglas: Compact Operators, Fredholm Operators, and Index Theory, chapter 5 in Banach Algebra Techniques in Operator Theory, Pure and Applied Mathematics 49 (1972) 121-148 [doi:10.1016/S0079-8169(08)60364-5]

• Gerard Murphy: §1.4 in: $C^\ast$-algebras and Operator Theory, Academic Press (1990) [doi:10.1016/C2009-0-22289-6]

• William Arveson, §3.3 of: A Short Course on Spectral Theory, Graduate Texts in Mathematics 209, Springer (2002) [doi:10.1007/b97227]

• M. W. Wong: Fredholm Operators, ch. 20 of: An Introduction to Pseudo-Differential Operators, Series on Analysis, Applications and Computation 6, World Scientific (2014) 143-153 [doi:10.1142/9789814583091_0020]

• Nora Doll, Hermann Schulz-Baldes, Nils Waterstraat: Bounded Fredholm Operators, Chapter 3 in: Spectral Flow — A Functional Analytic and Index-Theoretic Approach, Studies in Mathematics 94, De Gruyter (2023) [doi:10.1515/9783111172477, hdl:20.500.12657/63798]

Review:

• Martin Schechter: Fredholm Operators and the Essential Spectrum, New York University Courant Institute (1965) [pdf]

  (focus on their essential spectrum)

• Martin Schechter: Basic theory of Fredholm operators, Annali della Scuola Normale Superiore di Pisa - Scienze Fisiche e Matematiche, Serie 3, 21 2 (1967) 261-280 [numdam:ASNSP_1967_3_21_2_261_0]

• Ethan Y. Jaffe: Atkinson’s Theorem [pdf]

  (focus on Atkinson's theorem)

Discussion of the space of Fredholm operators as a classifying space for topological K-theory (Atiyah-Jänich theorem):

• Klaus Jänich: Vektorraumbündel und der Raum der Fredholm-Operatoren, Mathematische Annalen 161 (1965) 129–142 [doi:10.1007/BF01360851]

• Michael Atiyah, Appendix of: K-theory, Harvard Lecture 1964 (notes by D. W. Anderson), Benjamin (1967) [pdf, pdf]

• Michael Atiyah, §2 in: Algebraic Topology and Operators in Hilbert Space, in: Lectures in Modern Analysis and Applications I, Lecture Notes in Mathematics 103, Springer (1969) 101-121 [doi:10.1007/BFb0099987, pdf, pdf]

• DSBW23 ch 8: Homotopy theory of Fredholm operators [pdf]

and variants that serve as classifying spaces also for KO-theory and in any degree:

• Michael Atiyah, Isadore Singer: Index theory for skew-adjoint Fredholm operators, Publications Mathématiques de l’Institut des Hautes Scientifiques 37 1 (1969) 5-26 [doi:10.1007/BF02684885, pdf]

• Max Karoubi: Espaces Classifiants en K-Théorie, Trans. Amer. Math. Soc. 147 (1970) 75-115 [doi:10.2307/1995218, jstor:1995218, Engl. transl: pdf]

and relation to twisted equivariant K-theory:

• Michael Atiyah, Graeme Segal, §3 in: Twisted K-theory, Ukrainian Math. Bull. 1 3 (2004) [arXiv:math/0407054, journal page, published pdf]

• Daniel Freed, Michael Hopkins, Constantin Teleman, §A.5 in: Loop Groups and Twisted K-Theory I, J. Topology 4 (2011) 737-789 [arXiv:0711.1906, doi:10.1112/jtopol/jtr019]

See also:

• Wikipedia, Fredholm operator

• Alexander Grothendieck; La théorie de Fredholm, Bulletin de la Société Mathématique de France 84 (1956) 319-384 [numdam:1956__84__319_0]

• S. Rempel, B-W. Schulze, Index theory of elliptic boundary problems, Akademie–Verlag (1982)

• A. S. Mishchenko, Векторные расслоения и их применения (Vector bundles and their applications), Nauka, Moscow, (1984) [MR87f:55010]

• Lars Hörmander: The analysis of linear partial differential operators. III. Pseudo-differential operators, (1994, 2007)

• Pietro Aiena, Fredholm and local spectral theory, with applications to multipliers, Springer (2004) [doi:10.1007/1-4020-2525-4]

• Otgonbayar Uuye, A simple proof of the Fredholm Alternative [arxiv:1011.2933]

• Marina Prokhorova, Spectral Sections, arXiv:2008.04672.

• Marina Prokhorova, Spaces of unbounded Fredholm operators. I. Homotopy equivalences, arXiv:2110.14359.

• Marina Prokhorova, The continuity properties of discrete-spectrum families of Fredholm operators, arXiv:2201.09869.

• Marina Prokhorova, From graph to Riesz continuity, arXiv:2202.03337.

On Fredholm complexes:

• Graeme Segal, Fredholm complexes, Quarterly Journal of Mathematics 21:4 (1970), 385–402. doi.

Self-adjoint

On (the space of) self-adjoint Fredholm operators:

• Nora Doll, Hermann Schulz-Baldes, Nils Waterstraat: Bounded Self-Adjoint Fredholm Operators, Section 3.6 in: Spectral Flow — A Functional Analytic and Index-Theoretic Approach, Studies in Mathematics 94, De Gruyter (2023) [doi:10.1515/9783111172477, hdl:20.500.12657/63798]

As a classifying space for topological K-theory $KU^1$, $KO^1$ and $KSp^1$ (see at Atiyah-Jänich theorem):

• Michael F. Atiyah, Isadore M. Singer: Index theory for skew-adjoint Fredholm operators, Publications Mathématiques de l’IHÉS 37 (1969) 5-26 [doi:10.1007/BF02684885, numdam:PMIHES_1969__37__5_0, pdf]

• Max Karoubi: Espaces Classifiants en K-Théorie, Trans. Amer. Math. Soc. 147 (1970) 75-115 [doi:10.2307/1995218, jstor:1995218, Engl. transl: pdf]

More on the homotopy groups of this space:

• Bernhelm Booß-Bavnbek, Krzysztof P. Wojciechowski: The Homotopy Groups of the Space of Self-Adjoint Fredholm Operators, §16 in: Elliptic Boundary Problems for Dirac Operators Mathematics: Theory & Applications, Birkhäuser (1993) 127-137 [doi:10.1007/978-1-4612-0337-7_16, pdf]

• Hisham Sati, Urs Schreiber: The 3-Sphere of Self-Adjoint Fredholm Operators, §4.3.3 of: Orientations of Orbi-K-Theory measuring Topological Phases and Brane Charges, Part I of: Geometric Orbifold Cohomology, CRC Press (2026)

Specifically regarding their spectral flow:

• Michael F. Atiyah, Vijay K. Patodi, Isadore M. Singer: Spectral asymmetry and Riemannian Geometry. I, Mathematical Proceedings of the Cambridge Philosophical Society 77 1 (1975) 43-69 [doi:10.1017/S0305004100049410]

  (original mentioning, on p. 47-48, in the context of introducing the eta-invariant)

• John Phillips: Self-Adjoint Fredholm Operators And Spectral Flow, Canadian Mathematical Bulletin 39 4 (1996) 460-467 [doi:10.4153/CMB-1996-054-4]

• Alan Carey, John Phillips: Fredholm modules and spectral flow J. Canadian Math. Soc. 50 (1998) 673-718 [cms:10.4153/CJM-1998-038-x]

• Bernhelm Booß-Bavnbek, Matthias Lesch, John Phillips: Unbounded Fredholm Operators and Spectral Flow, Canadian Journal of Mathematics 57 2 (2005) 225-250 [doi:10.4153/CJM-2005-010-1, arXiv:math/0108014]

• Nils Waterstraat: Fredholm Operators and Spectral Flow [arXiv:1603.02009]

• Robert Skiba, Nils Waterstraat: The Index Bundle for Selfadjoint Fredholm Operators and Multiparameter Bifurcation for Hamiltonian Systems [arXiv:2012.05691]

• Nora Doll, Hermann Schulz-Baldes, Nils Waterstraat: Spectral Flow for Bounded Fredholm Operators, Chapter 4 in: Spectral Flow — A Functional Analytic and Index-Theoretic Approach, Studies in Mathematics 94, De Gruyter (2023) [doi:10.1515/9783111172477]

category: analysis