nLab
closed subspace

This entry is about closed subsets of a topological space. For other notions of “closed space” see for instance closed manifold.

---

Definition

A subspace $A$ of a space $X$ is closed if the inclusion map $A\hookrightarrow X$ is a closed map.

The closure of any subspace $A$ is the smallset closed subspace that contains $A$, that is the intersection of all open subspaces of $A$. The closure of $A$ is variously denoted $\mathrm{Cl}(A)$, ${\mathrm{Cl}}_X(A)$, $\overline{A}$, $\overline{A}$, etc.

(There is a lot more to say, about convergence spaces, smooth spaces, schemes, etc.)

Topological spaces

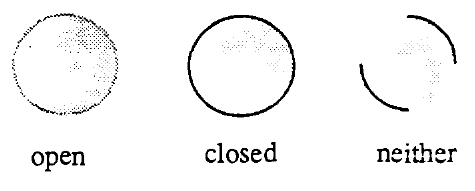
For a point-based notion of space such as a topological space, a closed subspace is the same thing as a closed subset.

A subset is closed precisely if

• it contains all its limit points.

Locales

In locale theory, every open $U$ in the locale defines a closed subspace which is given by the closed nucleus

$$j_{U\prime }:V\mapsto U\cup V.$$j_{U'}\colon V \mapsto U \cup V .

The idea is that this subspace is the part of $X$ which does not involve $U$ (hence the notation $U\prime$, or any other notation for a complement), and we may identify $V$ with $U\cup V$ when we are looking only away from $U$.

Related concepts

• open subset, clopen subset

• locally closed set

• closed cover

• Moore closure