nLab Fukaya category

Idea

The Fukaya category (named after Fukaya 1993) of a symplectic manifold $X$ is an A-∞ category having Lagrangian submanifolds of $X$ as objects.

When two Lagrangian submanifolds $L_1$ and $L_2$ of $X$ meet transversally, their hom-space in the Fukaya category is roughly defined as the vector space spanned by the intersection points $x\in L_1\cap L_2$.

One of the main difficulties in giving a rigorous definition of the Fukaya category in general relies precisely in the problem of correctly defining the hom-spaces for nontransversal intersections. As one could expect, the same difficulty carries on to the definition of the multilinear operations in the Fukaya category: when Lagrangians $L_1, L_2,\dots,L_{k+1}$ intersect transversally one has a clear geometric intuition of the multiplication

$$m_k \,\colon\, Hom(L_1,L_2)\otimes\cdots\otimes Hom(L_k,L_{k+1}) \longrightarrow Hom(L_1,L_{k+1})$$

in terms of counting pseudo-holomorphic disks into $X$ whose boundaries lie on the given Lagrangian submanifolds, but when intersections are nontransverse, the definition of $m_k$ becomes more elusive.

When defined, the Fukaya category is an $A_\infty$-category which consitutes one side of the duality of homological mirror symmetry.

In string theory

A-Model topological string

In string theory, the Fukaya category of a symplectic manifold $X$ represents the category of D-branes in the A-model – the A-branes – with target space $X$. For Landau-Ginzburg models, the category of A-branes is described by Fukaya-Seidel categories.

The assignment that sends a symplectic manifold to its Fukaya category extends to a functor on a variant of the symplectic category with Lagrangian correspondences as morphisms. This is supposed to be the FQFT incarnation of Donaldson theory. See at Lagrangian correspondences and category-valued TFT for more on this and see at homological mirror symmetry.

Yukawa couplings in intersecting D-brane models

In intersecting D-brane models Yukawa couplings are encoded by worldsheet instantons of open strings stretching between the intersecting D-branes (see Marchesano 03, Section 7.5). Mathematically this is encoded by derived hom-spaces in a Fukaya category (see Marchesano 03, Section 7.5).

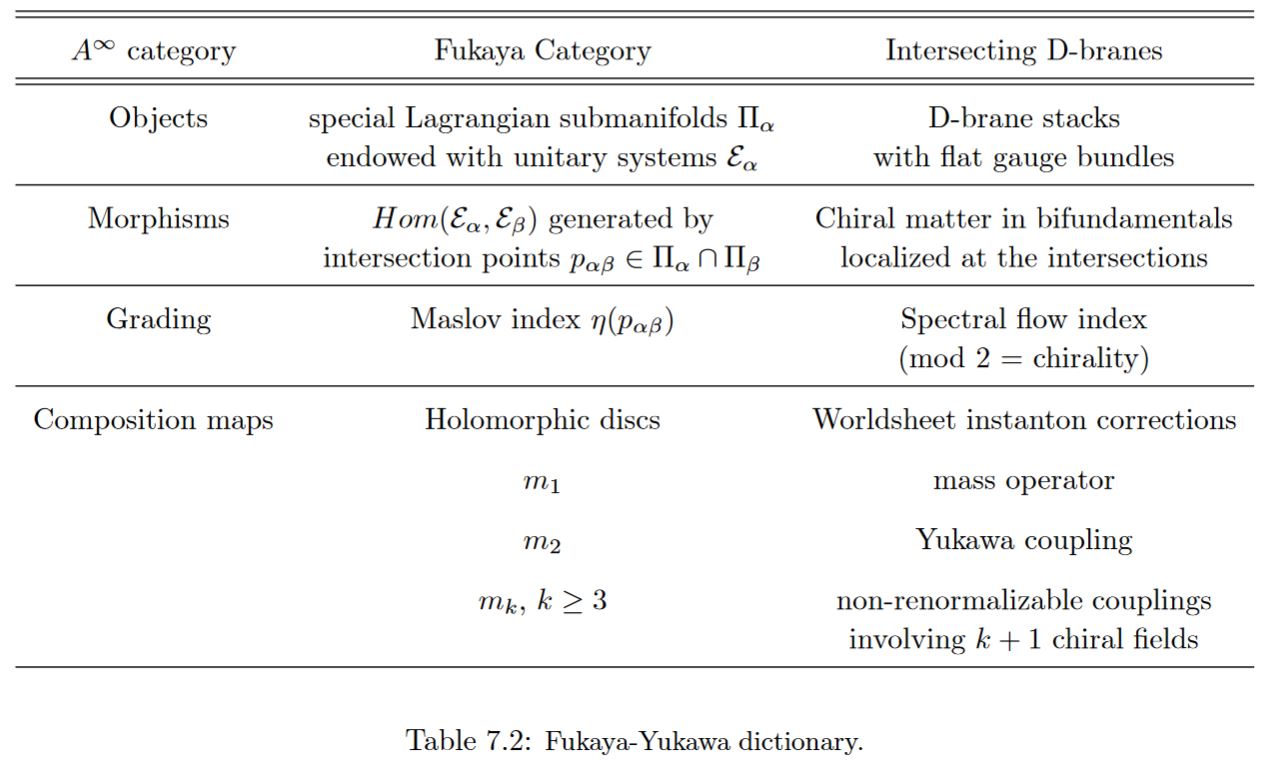
table grabbed from Marchesano 03

Related concepts

• Weinstein symplectic category

• intersecting D-brane model, Yukawa coupling

References

Fukaya categories are named after:

• Kenji Fukaya, Morse homotopy, $A_\infty$-category, and Floer homologies, Proceedings of GARC Workshop on Geometry and Topology ‘93 (Seoul, 1993) [pdf]

Monographs:

• Kenji Fukaya Yong-Geun Oh, Hiroshi Ohta, Karo Ono, Lagrangian intersection Floer theory - anomaly and obstruction, Studies in Advanced Mathematocs 46, AMS (2009) [ISBN:978-0-8218-5253-8]

• Paul Seidel, Fukaya categories and Picard-Lefschetz theory, EMS (2008) [doi:10.4171/063]

Introduction:

• Denis Auroux, A beginner’s introduction to Fukaya categories, lectures at Summer School on Contact and Symplectic Topology, Université de Nantes (June 2011) [arXiv:1301.7056]

and with an eye towards mirror symmetry:

• Felipe Espreafico G. Ramos, Mirror Symmetry and Fukaya Categories (2020) [pdf, pdf]

On the relation to Lagrangian cobordism:

• David Nadler, Hiro Tannaka, A stable infinity-category of Lagrangian cobordisms (arXiv:1109.4835)

Relation to Yukawa couplings in intersecting D-brane models:

• Fernando Marchesano, section 7.5 of Intersecting D-brane Models [arXiv:hep-th/0307252]