Schreiber Equivariant Super Homotopy Theory

a talk that I gave:

---

• Urs Schreiber,

  Equivariant Super Homotopy Theory

  talk at

  Geometry in Modal Homotopy Type Theory

  CMU Pittsburgh, 11-15 March 2019

  (slides, talk recording I, talk recording II)

---

$\,$

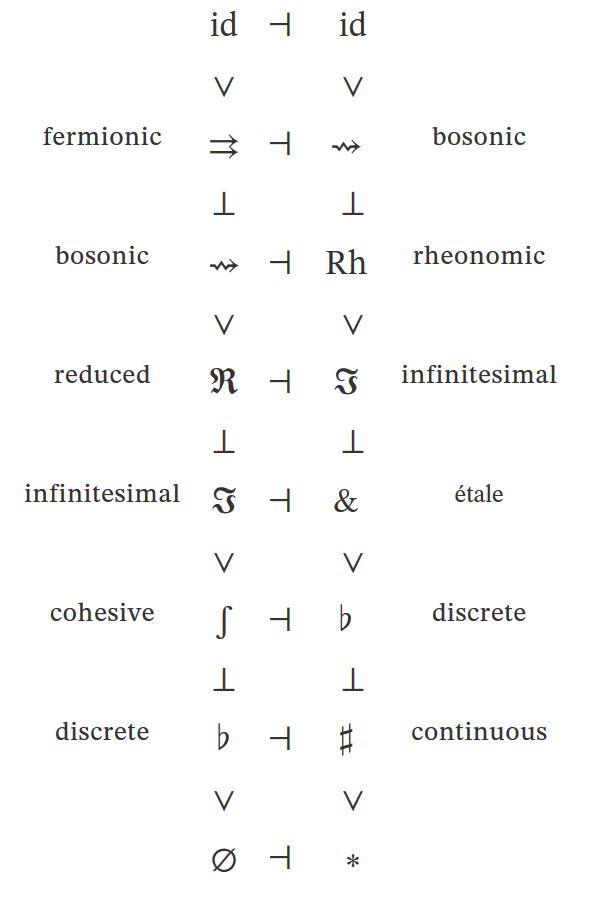
Abstract. Adding systems of adjoint modal operators to
$\;\phantom{\sim}$homotopy-type theory
$\;\sim$homotopy type-theory
makes it an elegant and powerful formal language
for reasoning about higher geometry,
specifically (and incrementally) for:

higher geometry	modal homotopy theory
differential topology, differential cohomology	cohesive
higher differential geometry, higher Cartan geometry	elastic
higher supergeometry	solid
orbifold cohomology	equivariant

The first two of these stages are discussed in other talks at this meeting (see in particular thesis Wellen)

In this talk I will:

1. describe higher supergeometry as intended categorical semantics for the full system of modalitites;

2. mention interesting theorems that should lend themselves to formalization in type theory;

3. indicate motivation from and application to the unofficial Millennium Problem of formulating M-theory (“Hypothesis H”, joint with Hisham Sati and Domenico Fiorenza).

---

$\,$

---

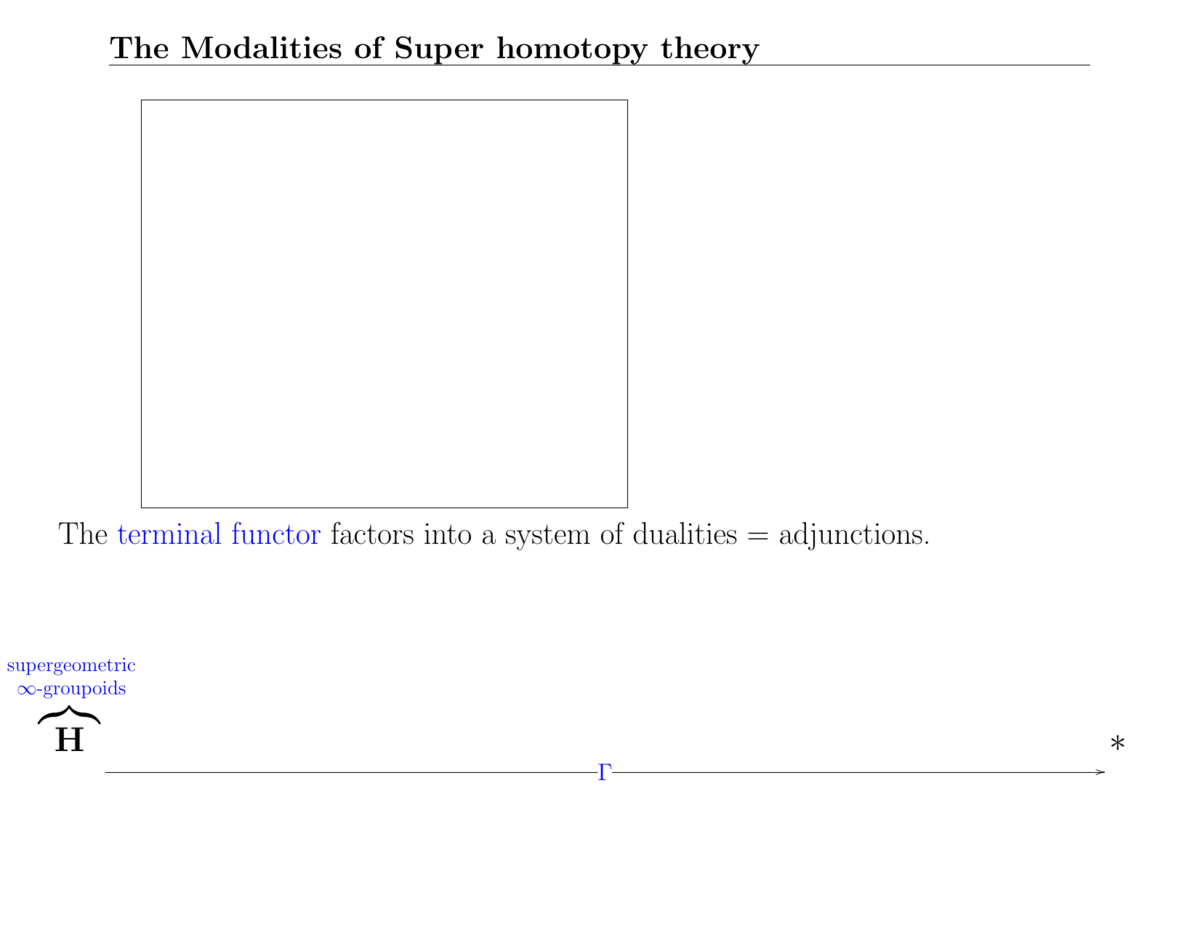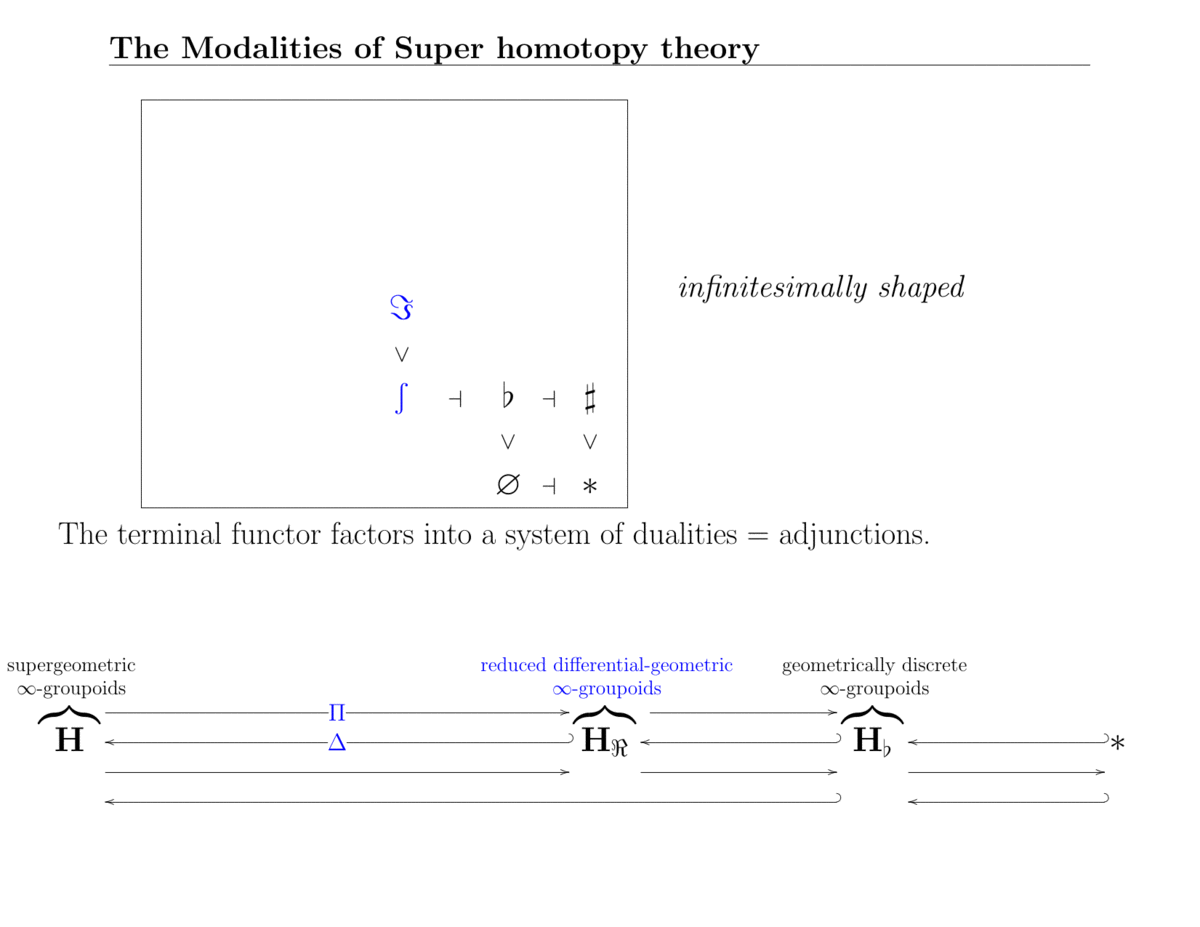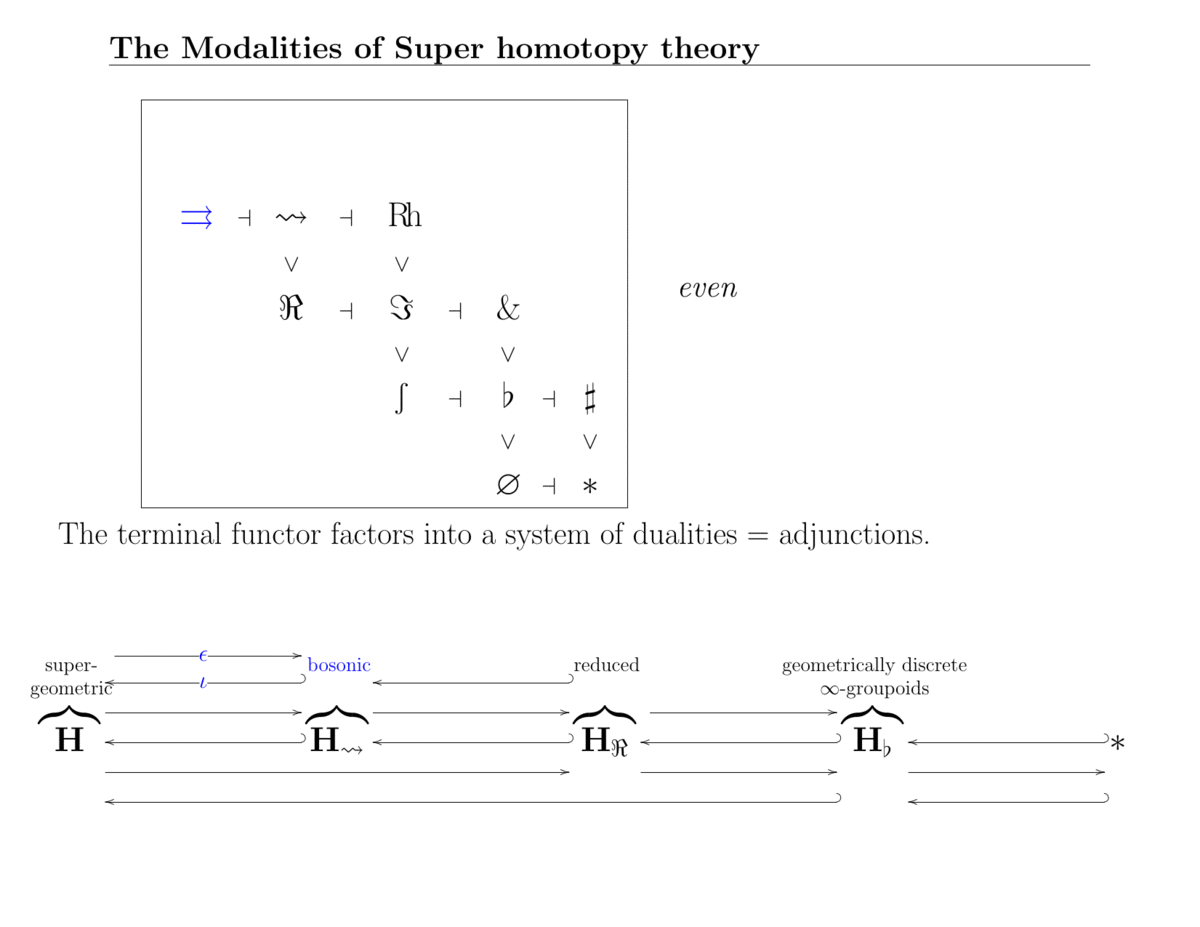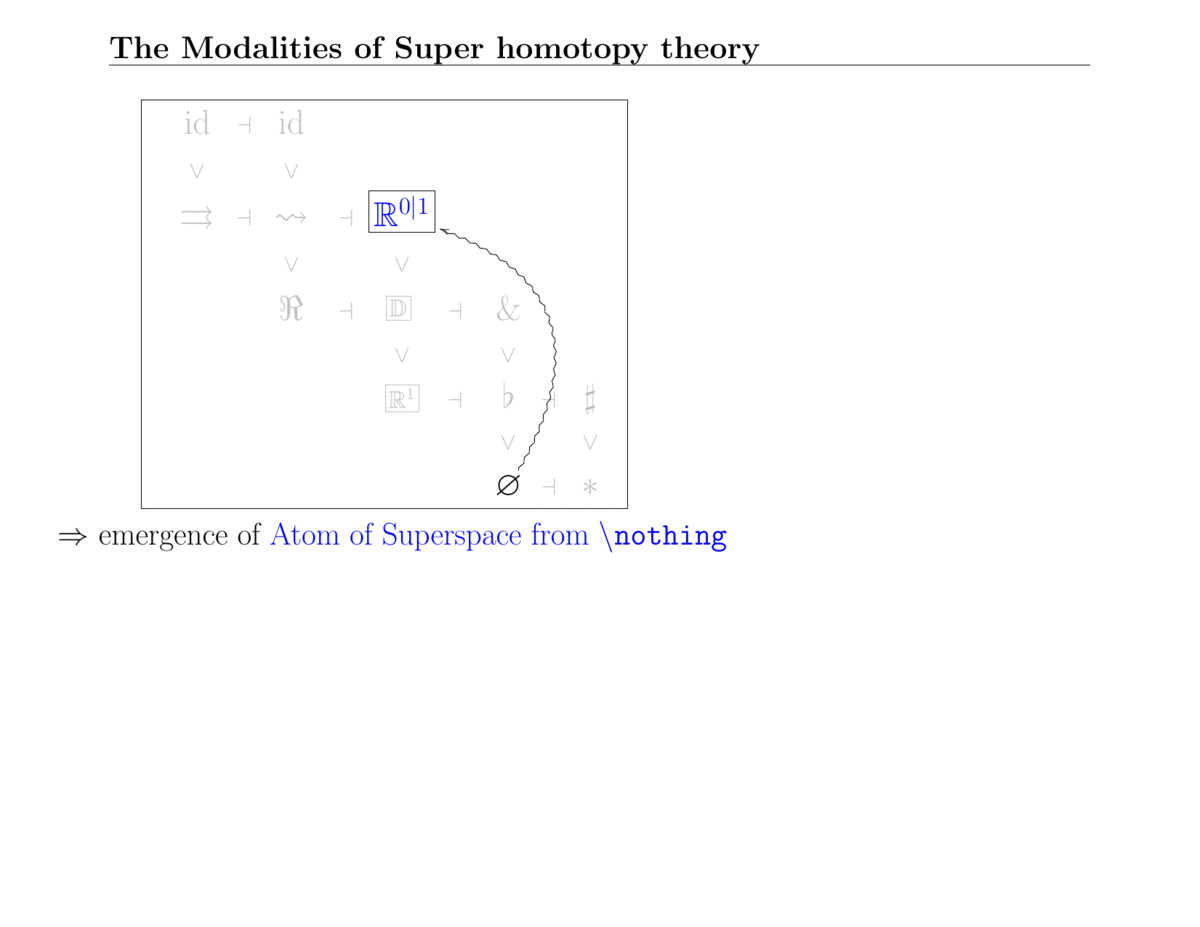
Related articles

This material eventually appeared as:

• Hisham Sati, Urs Schreiber:

  Proper Orbifold Cohomology

  arXiv:2008.01101

Related talks

• Super p-Brane Theory emerging from Super Homotopy Theory

  talk at StringMath2017, Hamburg 2017

• Equivariant Cohomotopy and Branes

  talk at String and M-Theory: The New Geometry of the 21st Century, Singapore 2018

• The rational higher structure of M-theory

  talk at Higher Structures in M-Theory, Durham 2018