nLab balanced monoidal category

Definition

A twist, or balance, in a braided monoidal category $\mathscr{C}$ is a natural isomorphism $\theta$ from the identity functor on $\mathscr{C}$ to itself satisfying the following compatibility condition with the braiding:

$$\theta_{A\otimes B}=\beta_{B,A}\circ \beta_{A,B}\circ (\theta_A\otimes \theta_B),\,\, \forall A,B\in\mathscr{C}$$

where $\beta$ is the braiding on $\mathscr{C}$. A balanced monoidal category is a braided monoidal category equipped with such a balance. Equivalently, a balanced monoidal category can be described as a braided pivotal category.

Balanced monoidal categories should not be confused with the other unrelated notation of a balanced category.

Properties

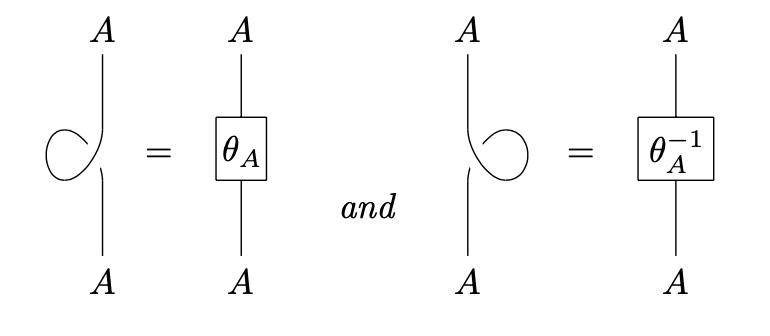
Every symmetric monoidal category is balanced in a canonical way. In fact, the identity natural transformation on the identity functor of $\mathscr{C}$ is a balance on $\mathscr{C}$. In this way, the twist can be seen as a way of “controlling” the non-symmetric behavior of the braiding.

In the language of string diagrams, the balancing is represented by a 360-degree twist:

References

This definition is taken from Jeff Egger (Appendix C), but the original definition can be found in chapter 4 of this paper by Joyal and Street:

• A. Joyal, R. Street, The geometry of tensor calculus I, Adv. Math. 88(1991), no. 1, 55–112, doi.