nLab function complex
Context
Mapping spaces
General abstract
-
hom-set, hom-object, internal hom, exponential object, derived hom-space
-
loop space object, free loop space object, derived loop space
Topology
Simplicial homotopy theory
Differential topology
Stable homotopy theory
Geometric homotopy theory
Homotopy theory
homotopy theory, (∞,1)-category theory, homotopy type theory
flavors: stable, equivariant, rational, p-adic, proper, geometric, cohesive, directed…
models: topological, simplicial, localic, …
see also algebraic topology
Introductions
Definitions
Paths and cylinders
Homotopy groups
Basic facts
Theorems
Contents
Idea
In categories of simplicial objects, like SimplicialSets, the sSet-valued hom-object between two objects is often called the function complex (“simplicial complex of functions from to ”).
The function complex of two simplicial sets is the collection of maps, left homotopies and higher homotopies between them, all itself organized into a simplicial set.
More formally, SimplicialSets is a Cartesian monoidal category and the corresponding internal hom is what is traditionally known as the function complex.
Definition
General
Suppose is a category that admits small coproducts.
Given simplicial objects , their function complex is the simplicial set
whose set of -simplices is the set of maps
where denotes the copowering of simplicial objects over simplicial sets given by
In simplicial sets
Specializing the general definition above to sSet itself, the required tensoring is just the product of simplicial sets and so
Definition
The function complex between simplicial sets is the cartesian internal hom given by
Properties
In simplicial sets
Proposition
(component formula for evaluation map on function complexes)
In SimplicialSets, the evaluation map of the function complex (from Def. ) — defined as the counit of the -adjunction — is given by:
where
denotes the unique non-degenerate -cell inside .
Proposition
(simplicial nerve intertwines function complexes with functor categories)
Let be small strict categories and write for their functor category. Then under the simplicial nerve this is isomorphic to the function complex (Def. ) between their separate simplicial nerves:
Examples
General
Example
(simplicial mapping complex between nerves of groupoids) For the nerves of groupoids, their simplicial mapping complex is isomorphic to the nerve of the functor groupoid from to :
Nerves of inertia groupoids
Example
(evaluation map on function complexes which are nerves of inertia groupoids)
Consider a discrete group . We write for its delooping groupoid, here specifically for its strict version . The nerve of this groupoid is the canonical model for the simplicial classifying space: The functor groupoid
is also known as the inertia groupoid of .
The simplicial nerve of this functor groupoid is the following function complex of simplicial sets (using Prop. ):
Here in the second step we introduced the simplicial set
which is the minimal model for , whose unique non-degenerate 1-cell we will denote by
We now describe in detail the evaluation map on the simplicial function complex
To that end, we denote by
the -cell in the nerve of the inertia groupoid that corresponds to the sequence of natural transformation which start at the functor
and successively have components .
Throughout we are writing “” for hom-sets and “” for hom-objects, i.e. for internal homs and we keep tacitly going back and forth through the bijections in (2).
If we abbreviate (this follows conventions familiar in discussion of transgression in group cohomology)
then this corresponds to a sequence of composable morphisms in the inertia groupoid of this form:
The simplicial map (2) maps the non-degenerate -cells in the product of simplicial sets (see the discussion there) of (1) with the simplicial -simplex as follows:
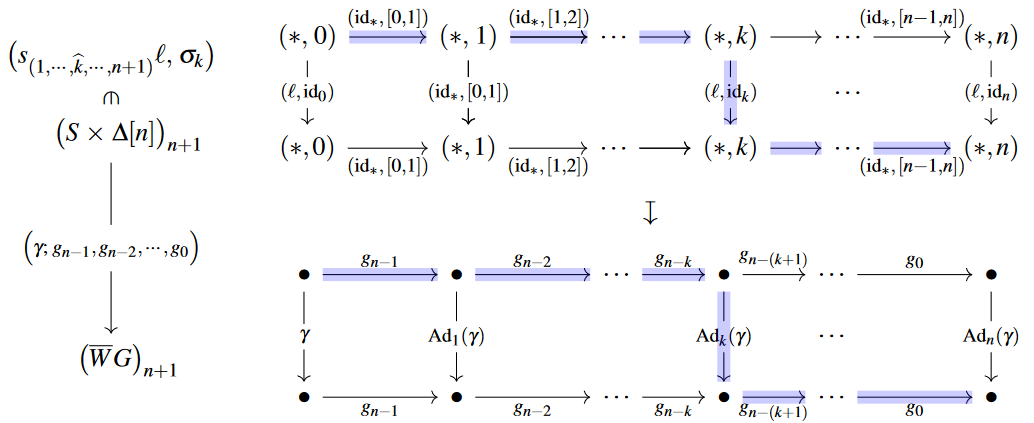
This is not exactly what we need unwinding the evaluation map, but it is close: the image of (2) under the th degeneracy map evidently gives the following mapping:

This is the type of mapping that appears in the component formula for the evaluation map on function complexes (from Prop. ). Hence we find that this evaluation map is given on non-degenerate -simplices in the product (see again there) as follows:
The expressions on the right happen to be those that appear in the formula for transgression in group cohomology, see there.
Related concepts
Related concepts
References
The original definition of a function complex in the generality stated above:
- Daniel M. Kan, On c.s.s. categories, Boletín de la Sociedad Matemática Mexicana 2 (1957), 82–94. PDF.
In function complxes in the generality of simplicial presheaves
- William Dwyer, Daniel Kan, Function complexes for diagrams of simplicial sets, Indagationes Mathematicae (Proceedings) 86 2 (1983) 139-147 [doi:10.1016/1385-7258(83)90051-3, pdf]
Textbook account in simplicial homotopy theory:
- Paul Goerss, J. F. Jardine, Section I.5 of: Simplicial homotopy theory, Progress in Mathematics, Birkhäuser (1999) Modern Birkhäuser Classics (2009) [doi:10.1007/978-3-0346-0189-4, webpage]
Introduction with an eye towards quasicategories:
- Charles Rezk, Section 15 of: Introduction to quasicategories (2022) [pdf, pdf]
Last revised on June 14, 2025 at 16:50:40. See the history of this page for a list of all contributions to it.