Schreiber The Character Map in Equivariant Twistorial Cohomotopy

An article that we have written at CQTS:

---

• Hisham Sati$\;$ and $\;$ Urs Schreiber:

  
  

  The Character Map in Twisted Equivariant Nonabelian Cohomology

  
  

  in:

  
  

  Applied Algebraic Topology

  special issue of

  Beijing Journal of Pure and Applied Mathematics

  (March 2025, in print)

  
  

  download:

  • pdf (v3: title and abstract adjusted)

  • arXiv:2011.06533

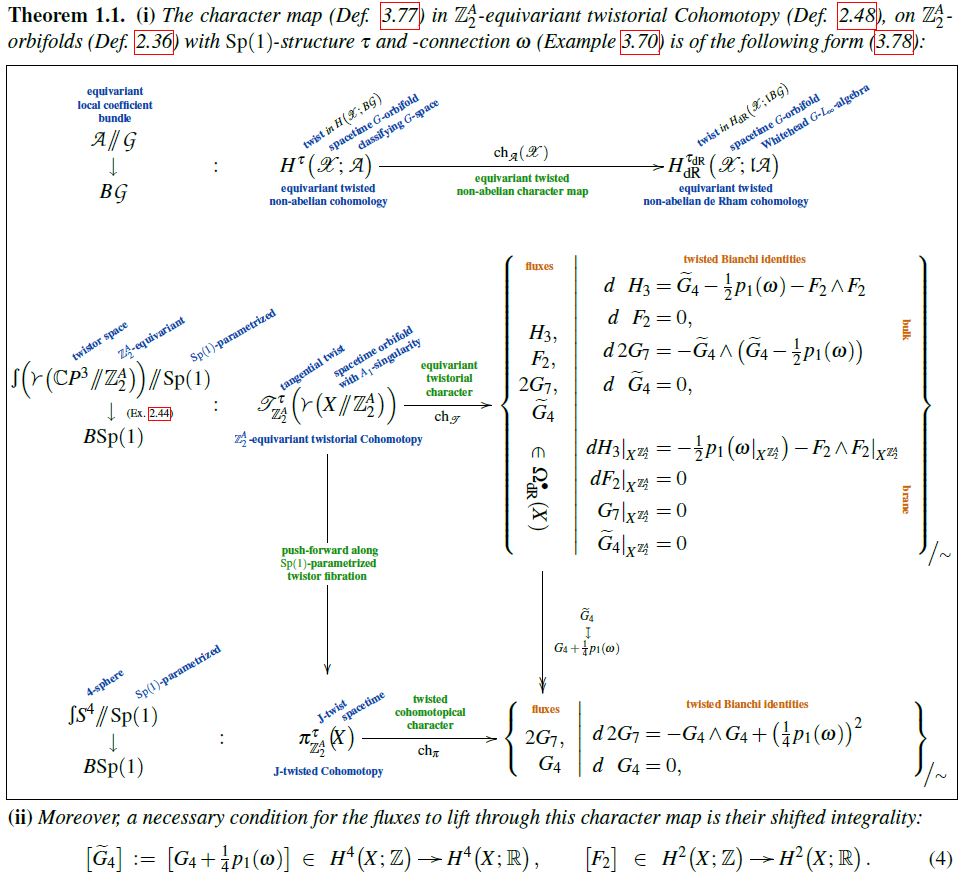
Abstract. The fundamental notion of non-abelian generalized cohomology gained recognition in algebraic topology as the nonabelian Poincaré-dual to “factorization homology”, and in theoretical physics as providing flux-quantization for non-linear Gauß laws. However, already the archetypical example — unstable Cohomotopy, first studied almost a century ago by Pontrjagin — has remained underappreciated as a cohomology theory and has only recently received attention as a flux quantization law (“Hypothesis H”).

Here we lay out a general construction of the analogue of the Chern character map on twisted equivariant non-abelian cohomology theories (with equivariantly simply-connected classifying spaces) and illustrate the construction by spelling out a twisted equivariant form of Cohomotopy as an archetypical and intriguing running example, essentially by computing its equivariant Sullivan model.

We close with an outlook on the application of this result to the the rigorous deduction of the proposed anyonic quantum states on M5-branes wrapped over Seifert 3-orbifolds.

Building on:

• The Character Map in Non-Abelian Cohomology

• Twistorial Cohomotopy implies Green-Schwarz anomaly cancellation

Followup article:

• Anyons on M5-Probes of Seifert 3-Orbifolds

---

---