Schreiber The Higher Structure of 11d Supergravity
A talk that I gave:
-
The Higher Structure
of
11-dimensional Supergravity,talk at
IHP Paris, May 2019
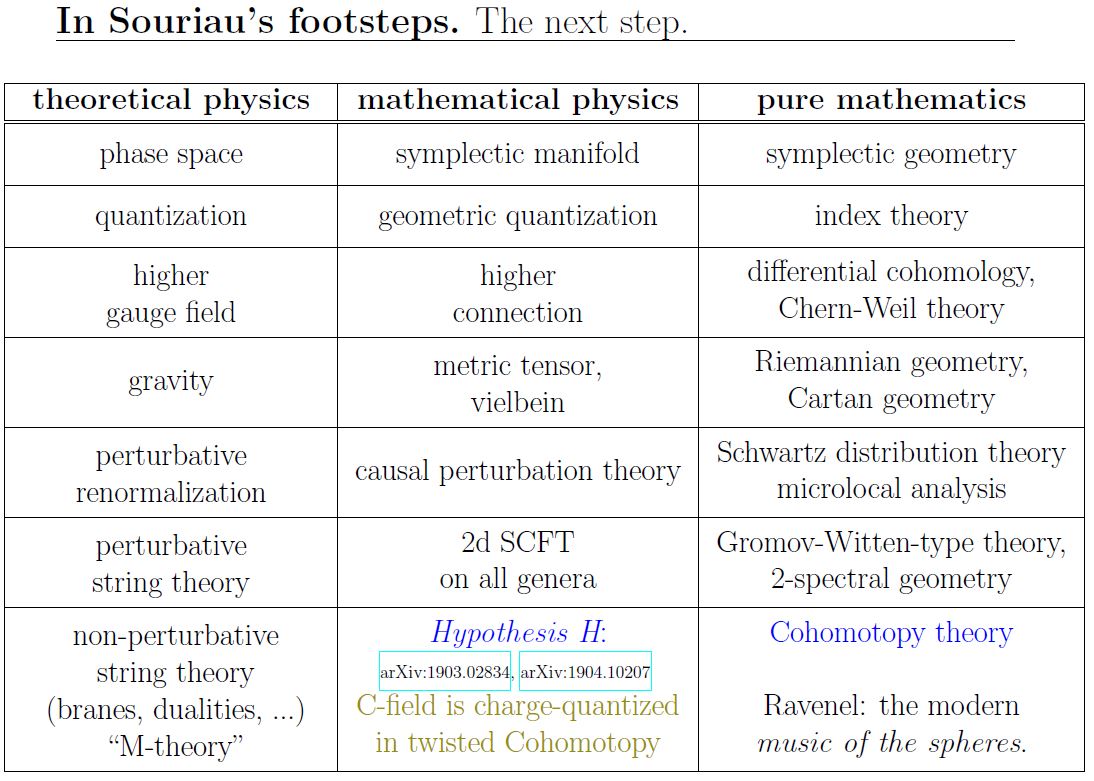
Abstract. Souriau‘s work has shown the immense fruitfulness of giving basic concepts in theoretical physics a precise mathematical foundation. This program of mathematical physics has been outstandingly successful throughout the 20th century, ranging from the mathematical formalization of classical gravity (via Riemannian geometry/Cartan geometry) and gauge theory (via Chern-Weil theory/differential cohomology) over perturbative renormalization (Schwartz distribution theory/microlocal analysis) to perturbative string theory (2d conformal field theory on all genera). However, the success story got stuck with the 2nd superstring revolution: The D-branes/M-branes and weak/strong-coupling string dualities that constitute the modern picture of putative “M-theory” (UV-completed 11-dimensional supergravity) have remained mathematical folklore. But it is precisely these non-perturbative effects that, via holographic QCD (intersecting D-brane models) plausibly solve the Millennium Problem of theoretical physics: non-perturbative QCD.
This talk presents recent progress (FSS 19a, FSS 19b, FSS 19c) on identifying mathematical foundations for M-theory in (co-)homotopy theory (aka “ higher structures”).
Based on:
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber:
The rational higher structure of M-theory,
Proceedings of the LMS-EPSRC Durham Symposium:
Higher Structures in M-Theory, August 2018,
Fortschritte der Physik, 2019
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber:
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber:
Twisted Cohomotopy implies level quantization of the full 6d Wess-Zumino-term of the M5-brane
Related talks:
-
Super p-Brane Theory emerging from Super Homotopy Theory
talk at StringMath2017,
Hamburg 2017
-
Equivariant Cohomotopy and Branes
talk at String and M-Theory: The New Geometry of the 21st Century,
Singapore 2018
-
The rational higher structure of M-theory
talk at Higher Structures in M-Theory,
Durham 2018
Last revised on June 22, 2019 at 17:44:19. See the history of this page for a list of all contributions to it.