nLab non-perturbative effect

Idea

An effect in non-perturbative quantum field theory that cannot be seen in perturbative quantum field theory is called a non-perturbative effect.

More in detail, theories with instanton field configurations (such as in Yang-Mills theory, hence in QCD and QED) or branes (such as in string theory), etc., are expected to have observables which as functions of the coupling constant $g$ are transseries of the form

(1)$$Z(g) = \sum_n a_n g^n + e^{-A/g} \sum_n a_n^{(1)} g^n + e^{-B/g^2} \sum_n b_n^{(1)} g^n \,,$$

where the first sum is the Feynman perturbation series itself and where the terms with a non-analytic dependence of the form $\exp(-A/g)$ or $\exp(-A/g^2)$ are the contributions of the instantons. Since all the derivatives of the functions $g \mapsto e^{-1/g}$ or $g \mapsto e^{-1/g^2}$ vanish at coupling constant $g = 0$, the Taylor series of this part of the observable does not appear in perturbative QFT, even though it is present. Therefore this is called a non-perturbative effect.

Related is resurgence theory. See also at perturbation theory – Divergence/convergence for more.

Notice that, while non-perturbative effects are generally the least understood, they are not exotic but ubiquitous: Essentially the entire parameter space of a generic “physical theory” is non-perturbative. In fact, the non-perturbative region of parameter space is the complement of an infinitesimally thickened point (labeled “perturbative” in the following graphics):

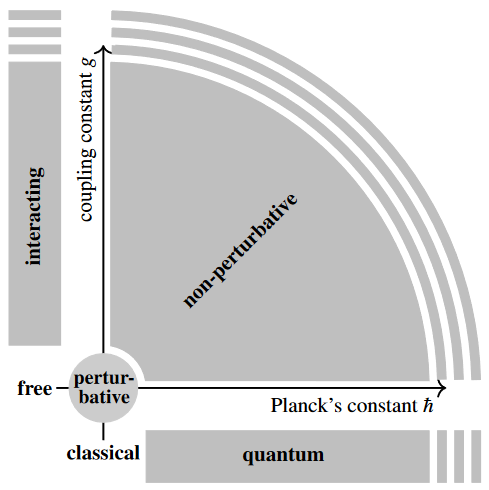
Examples

Schwinger effect

• Schwinger effect

Confinement and the mass gap

A central example of a non-perturbative effect is confinement (hence the “mass gap problem”) in Yang-Mills theory at low temperature. Perturbation theory is not suited to explain this (e.g Espiru 94, section 7).

Quark-gluon plasma

At the other extreme of high temperature QCD, also the quark-gluon plasma, while now deconfined is thought to be strongly coupled.

Hadronic physics in flavour and $g-2$ anomalies

Non-perturbative effects in hadron-physics affects the discussion of possible beyond-standard model physics as seen in

1. flavour anomalies (Nierste 18, 26/59)

2. muon anomalous magnetic moment (Jegerlehner 18b, section 2)

Worldsheet and brane instantons in string/M-theory

Consider the M-theory scales

• $\ell_P$, the Planck length in 11-dimensions;

• $R_{10}$ the length (circumference) of the $S^1_{10}$ circle fiber for KK-compactification to 10 dimensions

and the string theory scales

• $\ell_s$, the string length scale;

• $g_{s}$, the string coupling constant of perturbative string theory.

Then under the duality between M-theory and type IIA string theory these scales are related as follows:

$$\ell_P \;=\; g_{st}^{1/3} \ell_s \,, \phantom{AAA} R_{10} \;=\; g_{st} \ell_s$$

equivalently

$$\ell_s \;=\; R_{10}/ g_{st} \,, \phantom{AAA} \ell_P \;=\; g_{st}^{-2/3} R_{10}$$

equivalently

$$g_{st} \;=\; (R_{10}/\ell_P)^{3/2} \,, \phantom{AAA} \ell_s \;=\; \ell_P (R_{10}/\ell_P)^{-1/2} \,.$$

Hence a membrane instanton, which on a 3-cycle $C_3$ gives a contribution

$$\exp\left( - \frac{ vol(C_3) }{ \ell^3_P } \right)$$

becomes

1. if the cycle wraps, $C_3 = C_2 \cup S^1_{10}$, a worldsheet instanton

   $$\exp\left( - \frac{ vol(C_3) }{ \ell_P^3 } \right) \;=\; \exp\left( - \frac{ R_{10} vol(C_2) }{ g_{st} \ell_s^3 } \right) \;=\; \exp\left( - \frac{ vol(C_2) }{ \ell_s^2 } \right)$$

2. the cycle does not wrap, a spacetime instanton contribution, specifically a D2-brane instanton?

   $$\exp\left( - \frac{ vol(C_3) }{ \ell_P^3 } \right) \;=\; \exp\left( - \frac{ vol(C_3)/\ell_s^3 }{ g_{st} } \right)$$

(This unification of the two different non-perturbative effects in perturbative string theory (worldsheet instantons and spacetime instantons), to a single type of effect (membrane instanton) in M-theory was maybe first made explicit in Becker-Becker-Strominger 95. Brief review includes Marino 15, sections 1.2 and 1.3).

Related concepts

• non-perturbative quantum field theory

• strict deformation quantization

• transseries, resurgence theory

• fiber bundles in physics

• instanton, brane, renormalon

  • membrane instanton

• lattice gauge theory

• perturbation theory – convergence/divergence

• higher curvature correction

• interacting vacuum

• proton spin crisis

• AdS/CFT correspondence, AdS/QCD correspondence

• string theory FAQ – Isn’t it fatal that the string perturbation series does not converge?

References

In field theory

• Alexander P. Bakulev, Dmitry Shirkov, Inevitability and Importance of Non-Perturbative Elements in Quantum Field Theory, Proceedings of the 6th Mathematical Physics Meeting, Sept. 14–23, 2010, Belgrade, Serbia, pp. 27–54 (arXiv:1102.2380, ISBN 978-86-82441-30-4, book webpage, book TOC pdf)

Discussion of traditional algebraic quantum field theory as being about non-perturbative effects (and therefore lacking any interesting models in 3+1 dimensions, so far…):

• Franco Strocchi, An Introduction to Non-Perturbative Foundations of Quantum Field Theory, Oxford University Press (2013) [doi:10.1093/acprof:oso/9780199671571.001.0001]

Genral introduction and toy examples (e.g. phi^4-theory or anharmonic oscillator):

• Mario Flory, Robert C. Helling, Constantin Sluka, Section 2 of: How I Learned to Stop Worrying and Love QFT (arXiv:1201.2714)

• Mariño 12, section 2, section 3.1

Discussion for phi^4 theory is in

• Marco Serone, from 2:46 on in A look at $\phi^4_2$ using perturbation theory, January 2018 (recording)

Discussion for QCD:

• Marcos Mariño: Instantons and Large $N$ – An Introduction to Non-Perturbative Methods in QFT, Cambridge University Press (2015) [doi:10.1017/CBO9781107705968, pdf]

and for (super-)Yang-Mills theory and string theory:

• Marcos Mariño, Lectures on non-perturbative effects in large N gauge theories, matrix models and strings, Fortschritte der Physik 62 5‐6 (2014) 455-540 [arXiv:1206.6272]

• Marcos Mariño: Non-perturbative effects in string theory and AdS/CFT, Spring School on Superstring Theory and Related Topics 2015 [pdf, pdf, recording]

In QCD (hadrodynamics) via operator product expansion:

• Mikhail Shifman, OPE-based Methods in Nonperturbative QCD, Eur. Phys. J. C (2022) [arXiv:2208.10600]

In phenomenology

In flavour anomalies:

• Ulrich Nierste, Flavour Anomalies: Phenomenology and BSM Interpretations, 2018 (pdf)

• Fred Jegerlehner, The Role of Mesons in Muon $g-2$ (arXiv:1809.07413)

In cosmology

In cosmology:

• Anna Ijjas, Frans Pretorius, Paul Steinhardt, Stability and the gauge problem in non-perturbative cosmology, Journal of Cosmology and Astroparticle Physics, Volume 2019, January 2019 (arXiv:1809.07010, doi:10.1088/1475-7516/2019/01/015)

In string theory

The form of the contribution of non-perturbative effects in string theory was originally observed in

• Stephen Shenker, The Strength of nonperturbative effects in string theory, presented at the Cargese Workshop on Random Surfaces, Quantum Gravity and Strings, Cargese, France, May 1990 (spire)

The interpretation via D-branes of non-perturbative effects in the string coupling constant is due to

• Joseph Polchinski, Combinatorics of boundaries in string theory, Phys. Rev. D 50, 6041 (1994) (arXiv:hep-th/9407031)

The identification of non-perturbative contributions in string theory with brane contributions is due to

• Katrin Becker, Melanie Becker, Andrew Strominger, Five-branes, membranes and nonperturbative string theory, Nucl. Phys. B 456, 130 (1995) (hep-th/9507158)

Review includes

• Marino 15

Reviews specifically in type II string theory include

• Hugo Looyestijn, Non-perturbative effects in type IIA string theory, Master Thesis 2006 (pdf)

• Angel Uranga, Non-perturbative effects and D-brane instanton resummation in string theory (pdf, pdf)

In M-Theory

The relation of non-perturbative effects in string theory to M-theory goes back to

• Edward Witten, section 2.2 of String Theory Dynamics In Various Dimensions, Nucl.Phys.B443:85-126,1995 (arXiv:hep-th/9503124)

In Becker-Becker-Strominger 95 it was realized that the worldsheet instantons and D-brane instantons of string theory unify to membrane instantons (see Marino 15, section 1.3)