nLab permutative category

Idea

A permutative category is a symmetric monoidal category (possibly taken to be internal to Top) in which associativity and unitality hold strictly. Also known as a symmetric strict monoidal category.

Definition

(May, def. 1) (Elmendorf-Mandell, def. 3.1).

A permutative category is a strict monoidal category equipped with a natural transformation $B_{x,y}:x \otimes y \rightarrow y \otimes x$ such that:

• $(B_{x,y} \otimes Id_{z});(Id_{y} \otimes B_{x,z}) = B_{x,y \otimes z}$
• $B_{x,y};B_{y,x} = Id_{x \otimes y}$

In string diagrams:

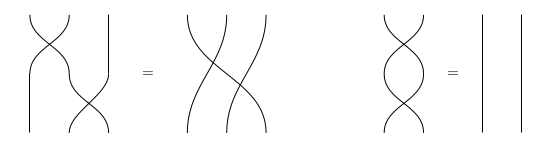
Properties

Every symmetric monoidal category is equivalent to a permutative one (Isbell).

The nerve of a permutative category is an E-infinity space, and therefore can be infinitely delooped to obtain an infinite loop space as its group completion.

Proposition

In a permutative category, for every object $x$, we have $B_{x,1}=Id_{x}$.

Proof

Apply the two equations of the definition by putting $y=1$ and $z=1$. We obtain:

• $(B_{x,1})^{2} = B_{x,1}$
• $B_{x,1};B_{1,x} = Id_{x}$

We obtain that $B_{x,1}=Id_{x}$ by postcomposing the first equation by $B_{1,x}$.

Note that it is not really possible to do this proof by using string diagrams.

The equation $B_{x,1}=Id_{x}$ is taken as an axiom of a permutative category in the references above. This is maybe the consequence of a lack of care about what is an identity natural transformation in the definition of a strict monoidal category. The equations $f \otimes Id_{1} = f = Id_{1} \otimes f$ are of critical importance in the proposition above and they are obtained by requiring that the structural natural isomorphims $\lambda_{x}:1 \otimes x \rightarrow x$ and $\rho_{x}:x \otimes 1 \rightarrow x$ are identity natural transformations. However, even knowing this, the proposition is not completely trivial and appears in a version for non-strict braided monoidal categories in the paper “Braided monoidal categories” (Joyal, Street, 1986).

Related concepts

• PermCat

• K-theory of a permutative category

• bipermutative category

References

An original account is in

• John Isbell, On coherent algebras and strict algebras, J. Algebra 13 (1969)

Discussion in relation to symmetric spectra is in

• Stefan Schwede, chapter I, section 7.5 of Symmetric spectra (2012)

Discussion in the context of K-theory of a permutative category is in

• Peter May, The spectra associated to permutative categories, Topology 17 (1978) (pdf)

• Peter May, $E_\infty$-spaces, group completions, and permutative categories, London Math. Soc. Lecture Notes No. 11, 1974, 61-94 (doi:10.1017/CBO9780511662607.008, pdf)

• Peter May, $E_\infty$ Ring Spaces and $E_\infty$ Ring spectra, Springer lectures notes in mathematics, Vol. 533, (1977) (pdf) chaper VI

• Anthony Elmendorf, Michael Mandell, Rings, modules and algebras in infinite loop space theory, K-Theory 0680 (web, pdf)

Discussion in the context of equivariant stable homotopy theory is in

• Bert Guillou, Peter May, Permutative $G$-categories in equivariant infinite loop space theory, Algebr. Geom. Topol. 17 (2017) 3259-3339 (arXiv:1207.3459)