Schreiber Rational sphere valued supercocycles in M-theory

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Authors |

An article that we have written:


Abstract

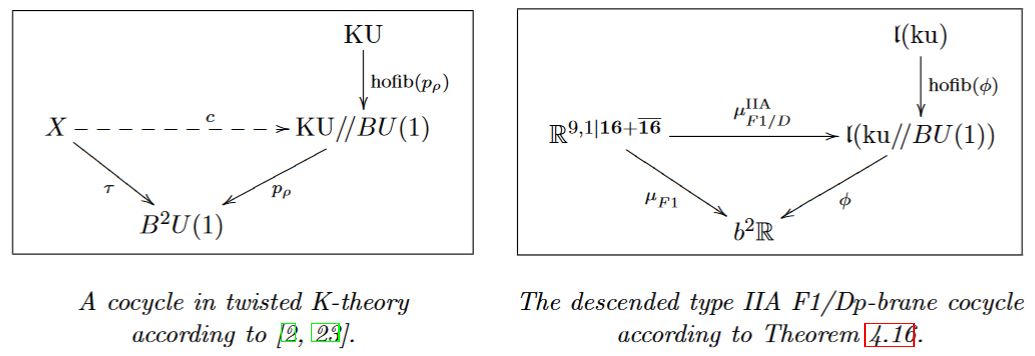
We show that supercocycles on super L-infinity algebras capture, at the rational level, the twisted cohomological charge structure of the fields of M-theory of type IIA string theory. We show that rational 4-sphere-valued supercocycles for M-branes in M-theory descend to supercocycles in type IIA string theory to yield the Ramond-Ramond fields predicted by the rational image of twisted K-theory, with the twist given by the B-field. In particular, we derive the M2/M5 ↔\leftrightarrow F1/Dp/NS5 correspondence via double dimensional reduction of sphere-valued L-∞ supercocycles in rational homotopy theory.


Related talks:


Related articles


Review and overview:


Followups:


Further development (going beyond rational Cohomotopy):



Last revised on December 2, 2022 at 16:00:13. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (15 revisions) Print Source