nLab lens space
Context
Manifolds and cobordisms
manifolds and cobordisms
cobordism theory, Introduction
Definitions
Genera and invariants
Classification
Theorems
Contents
Idea
A lens space is the quotient space
of a (2d+1)-dimensional sphere by a free action of a finite cyclic group (cf. group actions on spheres); specifically — under the identification of with the unit sphere in the real vector space underlying — by the action induced from a linear representation of the form
for natural numbers coprime to : .
Often this is considered by default for , in which case lens spaces are examples of 3-manifolds, and as such are in some sense the simplest after the 3-sphere and the product manifold of the 2-sphere with the circle.
Properties
Classification
Theorem
Two lens spaces and are homotopy equivalent iff there exists a with
In both theorems, the same for both lens spaces is a necessary condition for them to be homeomorphic or homotopy equivalent as follows with their respective fundamental group.
Cohomology
The ordinary integral cohomology of the lens space is [e.g. Maxim, p. 2]:
(where is the cyclic group or order , hence a torsion group).
Homotopy
Lens spaces are the only 3-manifolds with finite, cyclic, non-trivial fundamental group. The reverse direction of this statement (that a 3-manifold with such a fundamental group must be a lens space) is closely related to the Poincaré conjecture.
Lens spaces are important in geometric topology. They offer the simplest examples of smooth manifolds which cannot be distinguished by homotopy theory.
Via Dehn surgery
The 3-dimensional lens space , for coprime integers and , can be constructed by Dehn surgery on an unknot with coefficient . One can instead construct by an integral Dehn surgery on the framed ‘generalised Hopf link’
where the unknots have framings , , from left to right, for a continued fraction representation of as follows.
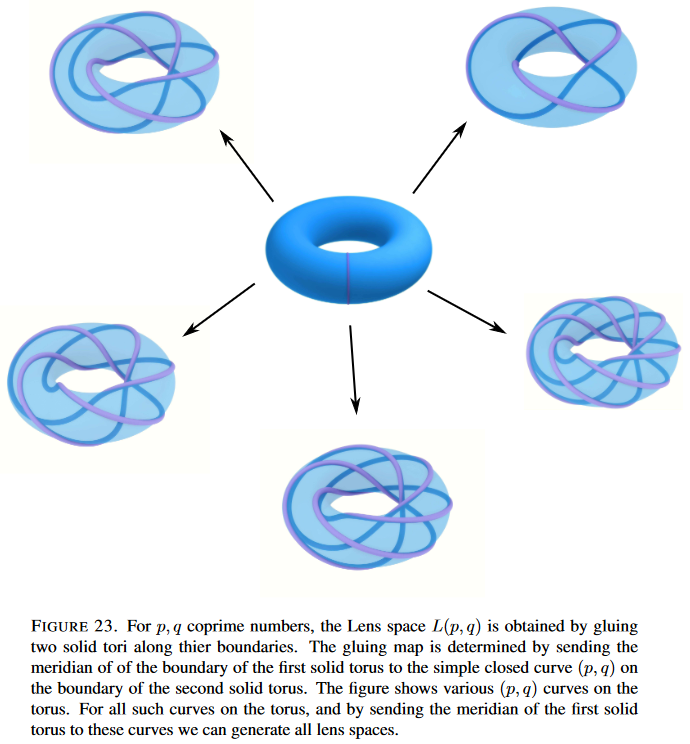
Related concepts
References
See also
-
Wikipedia, Lens space.
-
Neil Strickland, section 9 of: A Bestiary of Topological Objects [pdf, pdf]
In the context of orbifolds:
-
Siddhartha Gadgil, Equivariant framings, lens spaces and contact structures, Pacific Journal of Mathematics, Vol. 208 (2003), No. 1, 73–84 (doi:10.2140/pjm.2003.208.73)
-
Michel Boileau, Steven Boyer, Radu Cebanu, Genevieve S. Walsh, Section 3 of: Knot commensurability and the Berge conjecture, Geom. Topol. 16 (2012) 625-664 (arXiv:1008.1034)
On classification of lens spaces:
-
Paul Olum, Mappings of manifolds and the notion of degree, Ann. of Math. (2) 58 (1953), p. 458–480. JSTOR:1969748
-
E. J. Brody, The topological classification of the lens spaces, Ann. of Math. 71 1 (1960) 163–184. [doi:10.2307/1969884, jstor:, MR0116336 (22 #7125) Zbl 0119.18901]
-
John Milnor, Whitehead torsion, Bull. Amer. Math. Soc. 72 (1966) 358–426 [doi:10:1090/S0002-9904-1966-11484-2, maths.ed.ac.uk, jstor:bams/1183527946]
On the ordinary cohomology of lens spaces:
Via Dehn surgery:
- Mohamed Elhamdadi, Mustafa Hajij, Kyle Istvan, Fig 23 in: Framed Knots, Math Intelligencer 42 (2020) 7–22 [doi:10.1007/s00283-020-09990-0, arXiv:1910.10257]
On lens spaces in cosmic topology:
- Ralf Aurich, Sven Lustig, A survey of lens spaces and large-scale CMB anisotropy, Mon. Not. Roy. Astron. Soc. 424 (2012) 1556-1562 [arXiv:1203.4086, doi:10.1111/j.1365-2966.2012.21363.x]
On the 3d-3d correspondence for lens spaces:
- Du Pei, Chapter III of: 3d-3d correspondence for Seifert manifolds, 2016 (spire:1469350, pdf)
Last revised on May 10, 2025 at 07:34:55. See the history of this page for a list of all contributions to it.