Contents
Context
Algebra
Geometric quantization
Contents
Idea
In deformation quantization of Poisson manifolds the commutative product of the commutative algebra of functions is replaced by a noncommutative associative product. This is often called a star product and denoted “”.
An archetypal example is the Moyal star product (example below) that deforms the function algebra on a Poisson vector space, and often “star product” is by default understood to be a Moyal star product. Indeed every star product induced from a constant rank-2 tensor on a vector space is isomorphic to a Moyal star product (prop. below).
More recently also nonassociative “star products” have been proposed to be of interest.
Definition
On finite-dimensional vector spaces
Let be a finite dimensional vector space and let be an element of the tensor product (not necessarily skew symmetric at the moment).
We may canonically regard as a smooth manifold, in which case is canonically regarded as a constant rank-2 tensor. As such it has a canonical action by forming derivatives on the tensor product of the space of smooth functions:
If is a linear basis for , identified, as before, with a basis for , then in this basis this operation reads
where denotes the partial derivative of the smooth function along the th coordinate, and where we use the Einstein summation convention.
For emphasis we write
for the pointwise product of smooth functions.
Definition
(star product induced by constant rank-2 tensor)
Given as above, then the star product induced by on the formal power series algebra in a formal variable (“Planck's constant”) with coefficients in the smooth functions on is the linear map
given by
Hence
Proof
Observe that the product rule of differentiation says that
Using this we compute as follows:
In the last line we used that the ordinary pointwise product of functions is associative, and wrote for the unique pointwise product of three functions.
The last expression above is manifestly independent of the choice of order of the arguments in the triple star product, and hence it is clear that an analogous computation yields
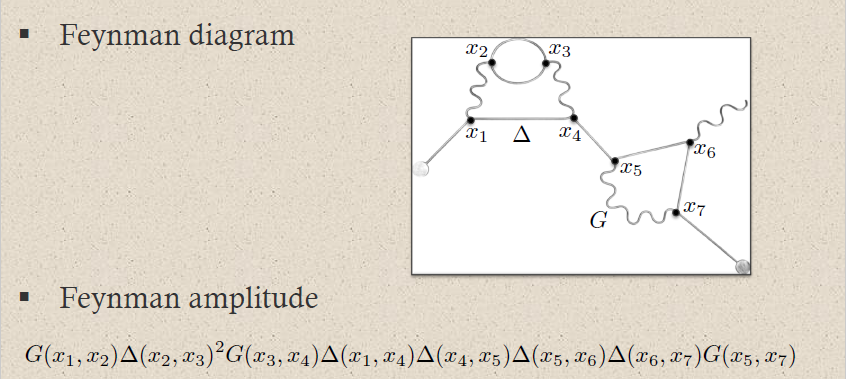
On polynomial observables in field theory
Definition
(star products on regular polynomial observables induced from propagators)
Let be a free Lagrangian field theory with field bundle , and let be a distribution of two variables on field histories.
On the off-shell regular polynomial observables with a formal paramater adjoined consider the bilinear map
given as in def. , but with partial derivatives replaced by functional derivatives
As in prop. this defines a unital and associative algebra structure.
If the Euler-Lagrange equations of motion induced by the Lagrangian density are Green hyperbolic differential equations and if is a propagator for these differential equations, then this star product algebra descends to the on-shell regular polynomial observables
(Dito 90, Dütsch-Fredenhagen 00 Dütsch-Fredenhagen 01, Hirshfeld-Henselder 02)
Properties
Equivalences of star products
Proposition
(shift by symmetric contribution is isomorphism of star products)
Let be a vector space, a rank-2 tensor and a symmetric rank-2 tensor.
Then the linear map
constitutes an isomorphism of star product algebras (prop. ) of the form
hence identifying the star product induced from with that induced from .
In particular every star product algebra is isomorphic to a Moyal star product algebra (example ) with the skew-symmetric part of , this isomorphism being exhibited by the symmetric part .
Proof
We need to show that
hence that
To this end, observe that the product rule of differentiation applied twice in a row implies that
Using this we compute
Integral representations
Proposition
(integral representation of star product)
If skew-symmetric and invertible, in that there exists with , and if the functions admit Fourier analysis (are functions with rapidly decreasing partial derivatives), then their star product (def. ) is equivalently given by the following integral expression:
(Baker 58)
Proof
We compute as follows:
Here in the first step we expressed and both by their Fourier transform (inserting the Fourier expression of the delta distribution from this example) and used that under this transformation the partial derivative turns into the product with (this prop.). Then we identified again the Fourier-expansion of a delta distribution and finally we applied the change of integration variables and then evaluated the delta distribution.
Next we express this as the groupoid convolution product of polarized sections of the symplectic groupoid. To this end, we first need the following definnition:
Definition
(symplectic groupoid of symplectic vector space)
Assume that is the inverse of a symplectic form on . Then the Cartesian product
inherits the symplectic structure
given by
The pair groupoid on equipped with this symplectic form on its space of morphisms is a symplectic groupoid.
A choice of potential form for , hence with , is given by
Choosing the real polarization spanned by a polarized section is function such that
hence
(1)
Proposition
(polarized symplectic groupoid convolution product of symplectic vector space is given by Moyal star product)
Given a symplectic vector space , then the groupoid convolution product on polarized sections (1) on its symplectic groupoid (def. ), given by convolution product followed by averaging (integration) over the polarization fiber, is given by the star product (def. ) for the corresponding Poisson tensor , in that
(Weinstein 91, p. 446, Garcia-Bondia & Varilly 94, section V)
Proof
We compute as follows:
The first line just unwinds the definition of polarized sections from def. , the following lines each implement a change of integration variables and fnally in the last line we used prop. .
Examples
General examples
Some examples of star products as in def. :
Example
If in def. , then the star product is the plain pointwise product.
Wick algebras of normal ordered products
Finite dimensional
Definition
(Kähler vector space)
An Kähler vector space is a real vector space equipped with a linear complex structure as well as two bilinear forms such that the following equivalent conditions hold:
-
and ;
-
with regarded as a smooth manifold and with regarded as constant tensors, then is an almost Kähler manifold.
Example
(standard Kähler vector spaces)
Let equipped with the complex structure which is given by the canonical identification , hence, in terms of the canonical linear basis of , this is
Moreover let
and
Then is a Kähler vector space (def. ).
The corresponding Kähler manifold is regarded as a smooth manifold in the standard way and equipped with the bilinear forms extended as constant rank-2 tensors over this manifold.
If we write
for the standard coordinate functions on with
and
for the corresponding complex coordinates, then this translates to
being the differential 2-form given by
and with Riemannian metric tensor given by
The Hermitian form is given by
(for more see at Kähler vector space this example).
Definition
(Wick algebra of a Kähler vector space)
Let be a Kähler vector space (def. ). Then its Wick algebra is the formal power series vector space equipped with the star product (def. ) which is given by the bilinear form
hence:
(e.g. Collini 16, def. 1)
Proof
This follows directly from that fact that in the imaginary part coincides with the skew-symmetric part, so that
Example
(Wick algebra of a single mode)
Let be the standard Kähler vector space according to example , with canonical coordinates denoted and . We discuss its Wick algebra according to def. and show that this reproduces the traditional definition of products of “normal ordered” operators as above.
To that end, consider the complex linear combination of the coordinates to the canonical complex coordinates
which we use in the form
(with “” the traditional symbol for the amplitude of a field mode).
Now
so that with
we get
Using this, we find the star product
to be as follows (where we write for the plain commutative product in the formal power series algebra):
and so forth, for instance
If we instead indicate the commutative pointwise product by colons and the star product by plain juxtaposition
then this reads
This is the way the Wick algebra with its operator product and normal-ordered product is traditionally presented.
Infinite-dimensional
References
In perturbative quantum field theory: