nLab comonoid
Context
Monoid theory
monoid theory in algebra:
Algebra
Algebraic theories
Algebras and modules
Higher algebras
-
symmetric monoidal (∞,1)-category of spectra
Model category presentations
Geometry on formal duals of algebras
Theorems
Category theory
Concepts
Universal constructions
Theorems
Extensions
Applications
Contents
Definition
A comonoid (or comonoid object) in a monoidal category is a monoid object in the opposite category (which canonically becomes a monoidal category via the same tensor product operation as in ).
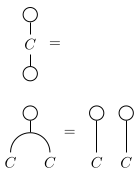
With the usual definition of monoids as having a unit, this means that a comonoid is equipped with a counit, which in string diagram-notation for is of this form:
One speaks of a unital comonoid (or unital comonoid object; internal to Vect also called an augmented coalgebra) if is in addition equipped with a morphism
which verifies the properties that the unit would verify if was a bimonoid, ie. in string diagrams:
Examples
Example
(coassociative coalgebras) A comonoid object in VectorSpaces (with its usual tensor product of vector spaces) is called a coalgebra.
(Beware though that the term “coalgebra” is overused in many ways, for instance in coalgebras for endofunctor?. To be more specific to the linear-algebraic context one can say coassociative coalgebra.)
Example
(cartesian comonoids)
Every set carries a unique structure of a comonoid in the category of Sets with respect to the usual cartesian product.
Generally, every object in a cartesian monoidal category becomes (see also there) a (cocommutative) comonoid by taking the
-
counit to be the terminal morphism
-
coproduct to be the diagonal morphism .
The analogous statement remains true for cartesian monoidal (infinity,1)-categories (see there).
Obvious as Exp. may be, it plays a somewhat profound role in various contexts:
Example
(suspension coring spectra)
In the case of topological spaces or other models of classical homotopy types, and using that the suspension spectrum-construction is a strong monoidal functor, Exp. implies the remarkable fact that suspension spectra carry coring spectrum-structure via smash-monoidal diagonals.
Related concepts
| (co)monad name | underlying endofunctor | (co)monad structure induced by |
|---|---|---|
| reader monad | on cartesian types | unique comonoid structure on |
| coreader comonad | on cartesian types | unique comonoid structure on |
| writer monad | on monoidal types | chosen monoid structure on |
| cowriter comonad | on monoidal types | chosen monoid structure on chosen comonoid structure on |
| Frobenius (co)writer | on monoidal types | chosen Frobenius monoid structure |
Last revised on December 20, 2023 at 07:53:32. See the history of this page for a list of all contributions to it.