nLab
Hopf invariant one

Idea

In algebraic topology, Hopf invariant one refers to homotopy classes of continuous functions between spheres of the form

$$\phi \;\colon\; S^{2n-1} \longrightarrow S^n \,,$$

hence to elements in the homotopy groups of spheres

$$[\phi] \in \pi_{2n-1}(S^n) \,,$$

whose Hopf invariant is equal to one:

$$h(\phi) = 1 \,.$$

Often this is regarded not in the integers but in its quotient to the cyclic group of order 2 $\mathbb{Z}/2$, where it hence says that the actual integer value is an odd number.

The Hopf invariant one problem, once a famous open problem, solved in Adams 60, is the classification of maps for which Hopf invariant is indeed equal to one.

Properties

Relation to H-space structure and normed division algebras

The existence of an element of Hopf invariant one in $\pi_{2n-1}(S^n)$ is equivalent to the existence of an H-space structure on $S^{n-1}$.

A celebrated theorem due to (Adams 60, introducing and using the Adams spectral sequence) states that maps of Hopf invariant one correspond precisely to the the Hopf constructions on the four normed division algebras (see also at Hurwitz theorem): the real Hopf fibration, the complex Hopf fibration, the quaternionic Hopf fibration and the octonionic Hopf fibration.

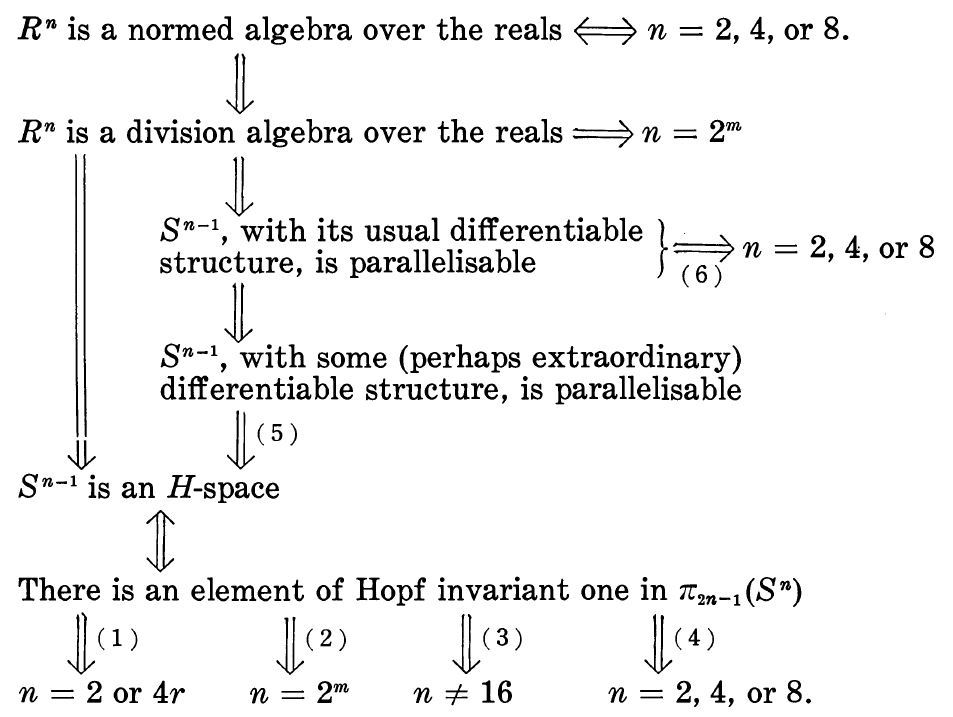
(Adams 60)

History

Stable homotopy theory emerged as a distinct branch of algebraic topology with Adams‘ introduction of his eponymous spectral sequence and his spectacular conceptual use of the notion of stable phenomena in his solution to the Hopf invariant one problem.

(Elmendorf-Kriz-May 95)

Related concepts

• e-invariant

• division algebra and supersymmetry

• cross product

• parallelizablen-sphere

exceptional spinors and real normed division algebras

Lorentzian spacetime dimension	$\phantom{AA}$spin group	normed division algebra	$\,\,$ brane scan entry
$3 = 2+1$	$Spin(2,1) \simeq SL(2,\mathbb{R})$	$\phantom{A}$ $\mathbb{R}$ the real numbers	super 1-brane in 3d
$4 = 3+1$	$Spin(3,1) \simeq SL(2, \mathbb{C})$	$\phantom{A}$ $\mathbb{C}$ the complex numbers	super 2-brane in 4d
$6 = 5+1$	$Spin(5,1) \simeq$ SL(2,H)	$\phantom{A}$ $\mathbb{H}$ the quaternions	little string
$10 = 9+1$	Spin(9,1) ${\simeq}$ “SL(2,O)”	$\phantom{A}$ $\mathbb{O}$ the octonions	heterotic/type II string

• Arf-Kervaire invariant problem

References

The original proof that the only maps of Hopf invariant one are the Hopf constructions on the four normed division algebras is due to

• Frank Adams, On the non-existence of elements of Hopf invariant one, Ann. Math., Vol. 72, No. 1, 72 (1): 20–104, (1960) (jstor:1970147)

• John Adams, Section 8 of: On the groups $J(X)$ IV, Topology 5: 21,(1966) (pdf, doi:10.1016/0040-9383(66)90004-8)

and made use of the classical Adams spectral sequence.

Review:

• Doug Ravenel, chapter 1, section 2 in: Methods of computing $\pi_\bullet(S^n)$ in: Complex cobordism and stable homotopy groups of spheres

• John Rognes, around lemma 4.14, theorem 4.15 of The Adams spectral sequence, 2012 (pdf)

• Joseph Victor, Section 2.4 of Stable Homotopy Groups of Spheres and The Hopf Invariant One Problem, 2013 (pdf)

Comments on the impact of this proof on the development of stable homotopy theory:

• Anthony Elmendorf, Igor Kriz, Peter May, Modern foundations for stable homotopy theory, in Ioan Mackenzie James, Handbook of Algebraic Topology, Amsterdam: North-Holland, (1995) pp. 213–253, (pdf)

Another proof that instead uses topological K-theory, Adams operations and the Atiyah-Hirzebruch spectral sequence was given in

• Frank Adams, Michael Atiyah, K-theory and the Hopf invariant, Quart. J. Math. Oxford (2), 17 (1966), 31-38 (pdf, doi:10.1093/qmath/17.1.31)

see also Adams 66, Sections 7, 8.

Review of the Hopf invariant one problem via Adams operations in topological K-theory:

• Dale Husemöller, chapter 15 of Fibre Bundles, Graduate Texts in Mathematics 20, Springer New York (1966)

• Klaus Wirthmüller, section 12 of: Vector bundles and K-theory, 2012 (pdf)

• Allen Hatcher, section 2.3 of Vector bundles and K-theory (web)

• Marcelo Aguilar, Samuel Gitler, Carlos Prieto, section 10.6 of Algebraic topology from a homotopical viewpoint, Springer (2002) (toc pdf)

• Michael Hopkins (notes by Akhil Mathew), Lecture 9 in: Spectra and stable homotopy theory, 2012 (pdf, pdf)

• Akhil Mathew, K-Theory and the Hopf invariant, 2012

• Gereon Quick, The Hopf invariant one problem via K-theory, lecture notes in: Advanced algebraic topology, 2014 (pdf)

• Ulrich Pennig, K-theory and the Hopf invariant

• Akhil Mathew, K-Theory and the Hopf invariant, 2012

• Ishan Banerjee, The Hopf invariant one problem, 2016 (pdf)

Review using the BP-Adams-Novikov spectral sequence includes

• Doug Ravenel, chapter 5, section 2 Complex cobordism and stable homotopy groups of spheres