nLab normed division algebra
Context
Algebra
- algebra, higher algebra
- universal algebra
- monoid, semigroup, quasigroup
- nonassociative algebra
- associative unital algebra
- commutative algebra
- Lie algebra, Jordan algebra
- Leibniz algebra, pre-Lie algebra
- Poisson algebra, Frobenius algebra
- lattice, frame, quantale
- Boolean ring, Heyting algebra
- commutator, center
- monad, comonad
- distributive law
Group theory
- group, normal subgroup
- action, Cayley's theorem
- centralizer, normalizer
- abelian group, cyclic group
- group extension, Galois extension
- algebraic group, formal group
- Lie group, quantum group
Ring theory
Module theory
Gebras
Normed division algebras
Idea
A normed division algebra is a not-necessarily associative algebra over the real numbers that is:
-
unital (there is an element such that for all ),
-
a division algebra , and
-
a normed algebra such that for all .
It turns out (by Hurwitz' theorem) that over the real numbers there are precisely only four finite-dimensional normed division algebras up to isomorphism: the algebras of
In this sense real normed division algebras may be thought of as a natural generalization of the real numbers and the complex numbers.
Moreover, if one regards the real numbers as a star-algebra with trivial anti-involution, then each step in the above sequence is given by applying the Cayley-Dickson construction. (While the process of applying the Cayley-Dickson construction continues, next with the sedenions, these and the following are no longer division algebras.)
This classification of real normed division algebras is closely related to various other systems of exceptional structures in mathematics and physics:
-
The Hopf invariant one theorem says that the only continuous functions between spheres of the form whose Hopf invariant is equal to 1 are the Hopf constructions on the four real normed division algebras, namely
-
Patterns related to Majorana spinors in spin geometry are intimately related to the four normed division algebras, and, induced by this, so is the classification of supersymmetry in the form of super Poincaré Lie algebras and super Minkowski spacetimes (which are built from these real spin representations). For more on this see at supersymmetry and division algebras.
(Moreover, apparently these two items are not unrelated, see here.)
Definition
A normed division algebra is
-
that is also a Banach algebra
-
such that
While the norm in a Banach algebra is in general only submultiplicative (), the norm in a normed division algebra must obey the stronger condition . Accordingly, this norm is considered to be an absolute value and often written instead of . There is also a converse: if the norm on a Banach algebra is multiplicative (including ), then it must be a division algebra. While the usual definition of a ‘normed division algebra’ does not include the completeness condition of a Banach algebra, in fact (UrbanikWright60) the only examples have finite dimension and are therefore complete, hence Banach algebras.
Any normed division algebras is in particular a composition algebra.
Properties
Classification
Over the complex numbers, the only normed division algebra is the algebra of complex numbers themselves.
The Hurwitz theorem says that over the real numbers there are, up to isomorphism, exactly four finite-dimensonal normed division algebras :
- , the algebra of real numbers,
- , the algebra of complex numbers,
- , the algebra of quaternions,
- , the algebra of octonions.
In fact these are also exactly the real alternative division algebras:
Proposition
The only division algebras over the real numbers which are also alternative algebras are the real numbers themselves, the complex numbers, the quaternions and the octonions.
(Zorn 30).
Each of these is produced from the previous one by the Cayley–Dickson construction; when applied to , this construction produces the algebra of sedenions, which do not form a division algebra.
The Cayley–Dickson construction applies to an algebra with involution; by the abstract nonsense of that construction, we can see that the four normed division algebras above have these properties:
- is associative, commutative, and with trivial involution,
- is associative and commutative but has nontrivial involution,
- is associative but noncommutative and with nontrivial involution,
- is neither associative, commutative, nor with trivial involution.
However, these algebras do all have some useful algebraic properties; in particular, they are all alternative (a weak version of associativity). They are also all composition algebras.
A normed field is a commutative normed division algebra; it follows from the preceding that the only normed fields over are and (e.g. Tornheim 52).
It is in fact true that all unital normed division algebras over are already finite dimensional, by (Urbanik-Wright 1960) (the authors give a reference on a non-unital infinite-dimensional normed division algebra). Hence the Hurwitz theorem together with Urbanik-Wright 1960 says that the above four exhaust all real normed division algebras.
Automorphisms
The automorphism groups of the real normed division algebras, as normed algebras, are
-
, the trivial group
-
the group of order 2, acting by complex conjugation;
-
, the special orthogonal group acting via its canonical representation on the 3-dimensional space of imaginary quaternions;
-
, the exceptional Lie group G2.
Incidentally, there is a sense in which this sequence of groups continues, with the infinity-group G3 (the Dwyer-Wilkerson H-space):
| 0 | 1 | 2 | 3 | 4 | |
|---|---|---|---|---|---|
| 1 | Z/2 | SO(3) | G2 | G3 | |
| = Aut(R) | = Aut(C) | = Aut(H) | = Aut(O) |
Relation to H-space structures on sphere (Hopf invariant one)
The Hopf invariant one theorem says that the spheres carrying H-space structure are precisely the unit spheres in one of the normed division algebras
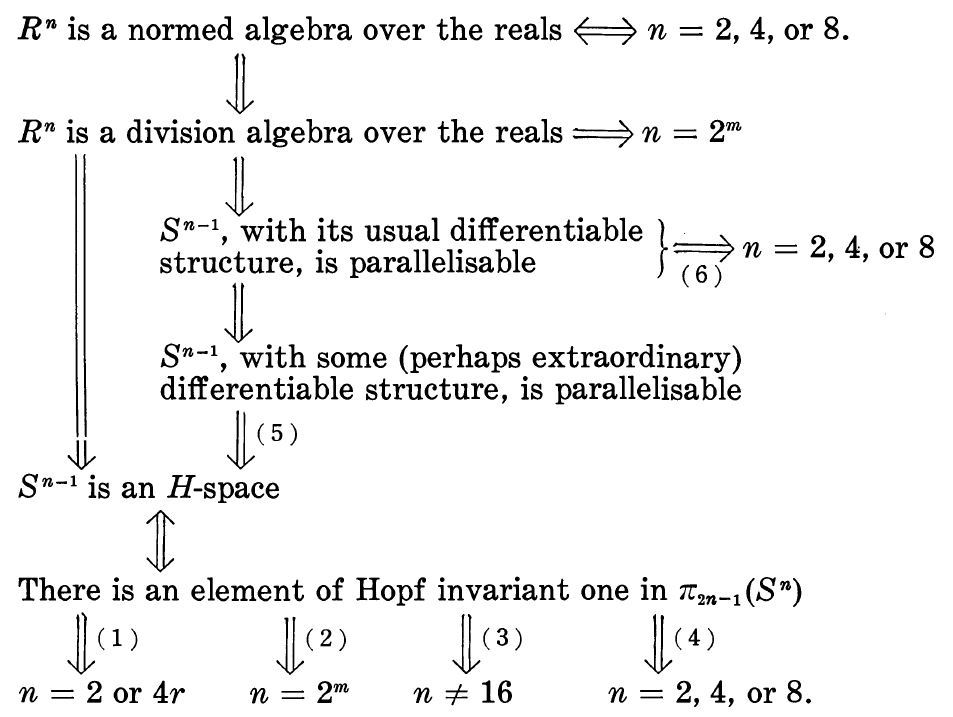
(Adams 60)
Magic square
The Freudenthal magic square is a special square array of Lie algebras/Lie groups labeled by pairs of real normed division algebras and including all the exceptional Lie groups except G2.
Related concepts
exceptional spinors and real normed division algebras
see division algebra and supersymmetry
(Leung 02)
References
The classification of real division composition algebras is originally due (Hurwitz theorem) to
- Adolf Hurwitz, Über die Composition der quadratischen Formen von beliebig vielen Variabeln, Nachr. Ges. Wiss. Göttingen (1898) 309–316
The alternative classification as real alternative division algebras is due to
- Max Zorn, Theorie der alternativen Ringe, Abhandlungen des Mathematischen Seminars der Universität Hamburg 8 (1930), 123-147
General discussion includes includes
-
Leonard Tornheim, Normed fields over the real and complex fields, Michigan Math. J. Volume 1, Issue 1 (1952), 61-68. (Euclid)
-
Silvio Aurora, On normed rings with monotone multiplication, Pacific J. Math. Volume 33, Number 1 (1970), 15-20 (JSTOR)
The result about removing the assumption of finite-dimensionality from unital normed division algebras appears in:
- Kazimierz Urbanik and Fred B. Wright, Absolute-valued algebras, Proc. Amer. Math. Soc. 11 (1960), 861-866, doi:10.1090/S0002-9939-1960-0120264-6
Exposition with emphasis on the octonions is in
Discussion of Riemannian geometry and special holonomy modeled on the different normed division algebras is in
- Naichung Conan Leung, Riemannian Geometry Over Different Normed Division Algebras, J. Differential Geom. Volume 61, Number 2 (2002), 289-333. (euclid)
Last revised on August 21, 2024 at 01:47:33. See the history of this page for a list of all contributions to it.