nLab wallpaper group

Definition

A wallpaper group is a crystallographic group (space group) in dimension 2, hence a subgroup $G \subset Iso(\mathbb{R}^2)$ of the isometry group of the Euclidean plane such that

1. the part of $G$ inside the translation group is generated by two linearly independent vectors;

2. the point group is finite.

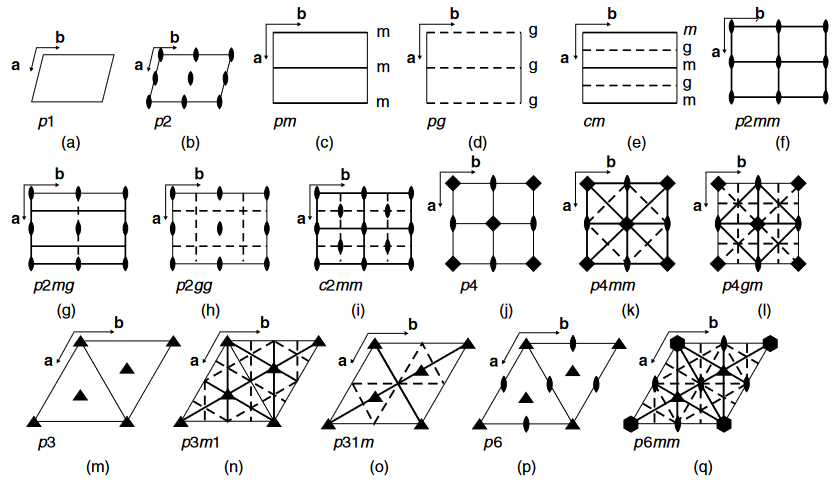
Tabulation

Crystallographic diagrams

Fig. 3.10 in Tilley 2020:

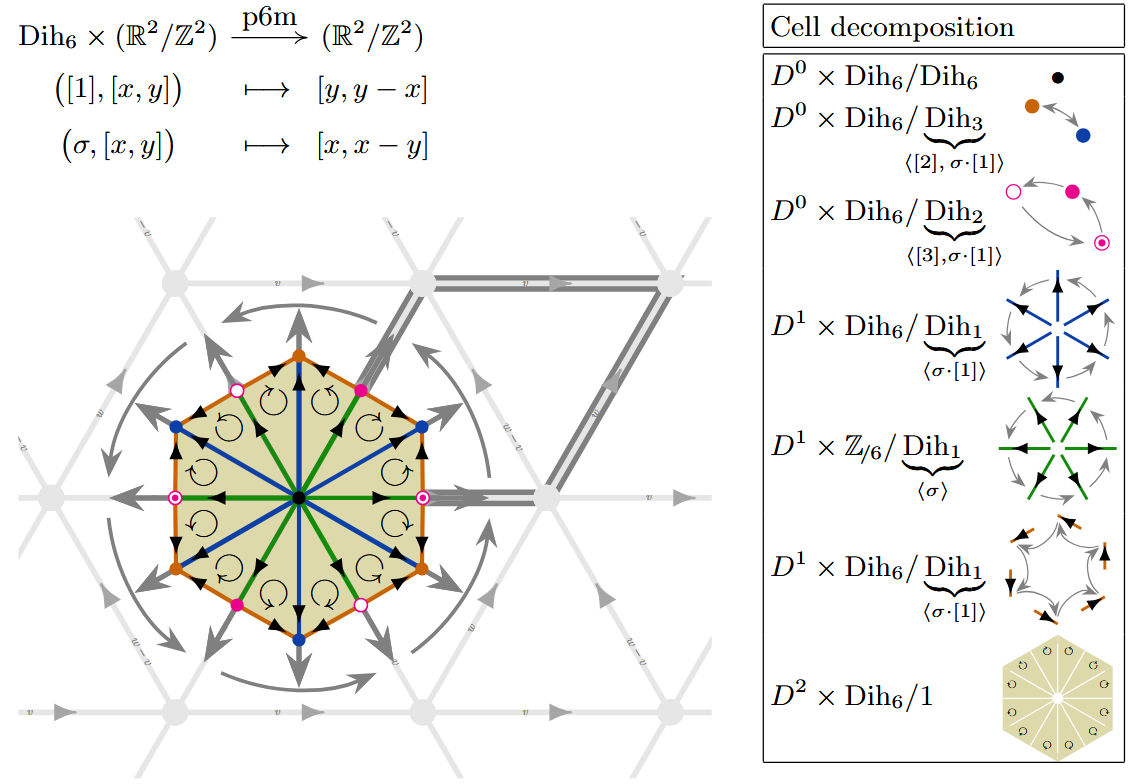
Equivariant cell structure on 2-tori

The following shows, for the symmorphic 2D crystallographic groups (wallpaper groups) $G \ltimes \mathbb{Z}^2 \subset Iso(2)$, the $G$-CW complex structure on the resulting tori $T^2 \coloneqq \mathbb{R}^2/\mathbb{Z}^2$ (graphics from SS25).

(The remaining non-symmorphic cases follow.)

The point groups $G$ arising are the cyclic groups $\mathbb{Z}_{/1} =$$1$, $\mathbb{Z}_{/2}$, $\mathbb{Z}_{/3}$, $\mathbb{Z}_{/4}$, $\mathbb{Z}_{/6}$ and the dihedral groups $Dih_1$, $Dih_2$, $Dih_3$, $Dih_4$, and $Dih_6$ (making 10 distinct point groups $G$, but the first three of the latter come with two inequivalent actions each).

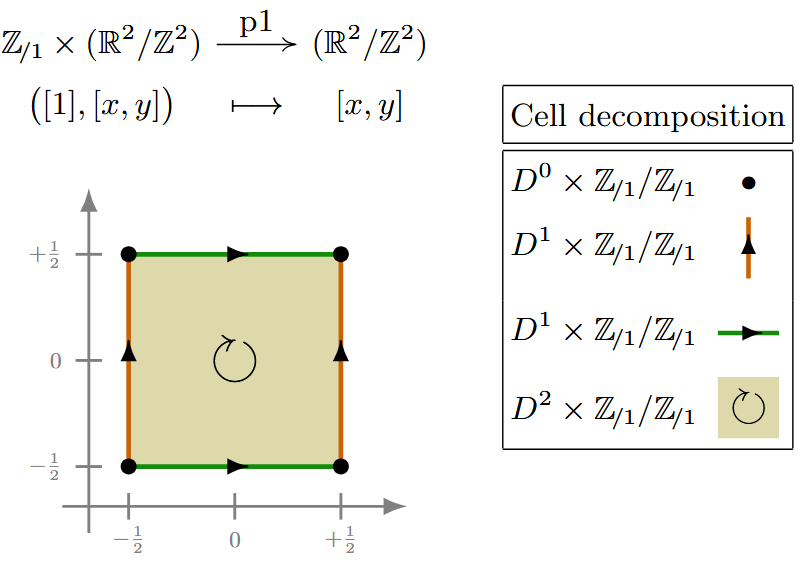
Example

(p1) For completeness, here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with trivial group-action, corresponding to the $p 1$ wallpaper group:

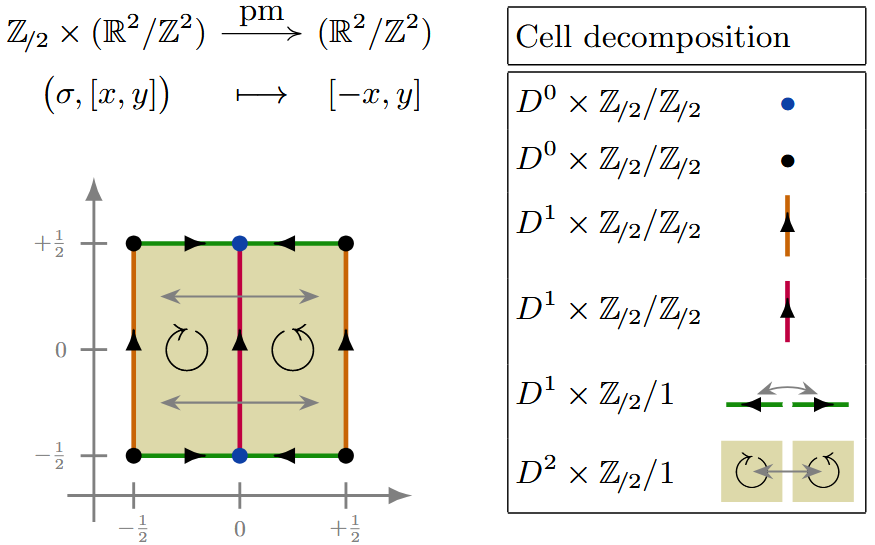
Example

(pm) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $Dih_1$ $\simeq$ $\mathbb{Z}_{/2}$-action which reflects one of the two coordinate axes, corresponding to the $p m$ wallpaper group:

Example

(cm) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $Dih_1$ $\simeq$ $\mathbb{Z}_{/2}$-action which reflects along the coordinate diagonal, corresponding to the $c m$ wallpaper group:

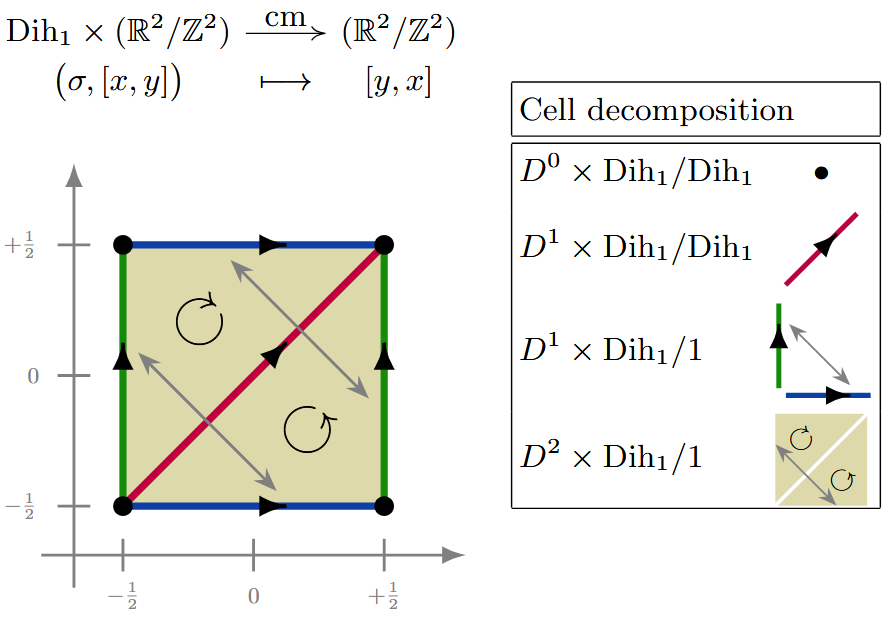
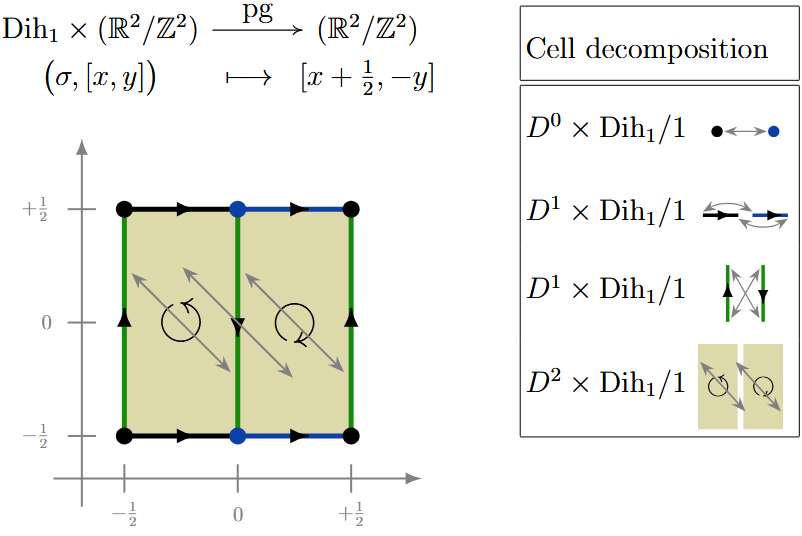
Example

(p2) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $\mathbb{Z}_{/2}$-action which rotates by multiples of $\pi$ around the origin, corresponding to the wallpaper group $p2$:

Example

(pmm) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with $Dih_{2}$-action according to the wallpaper group pmm:

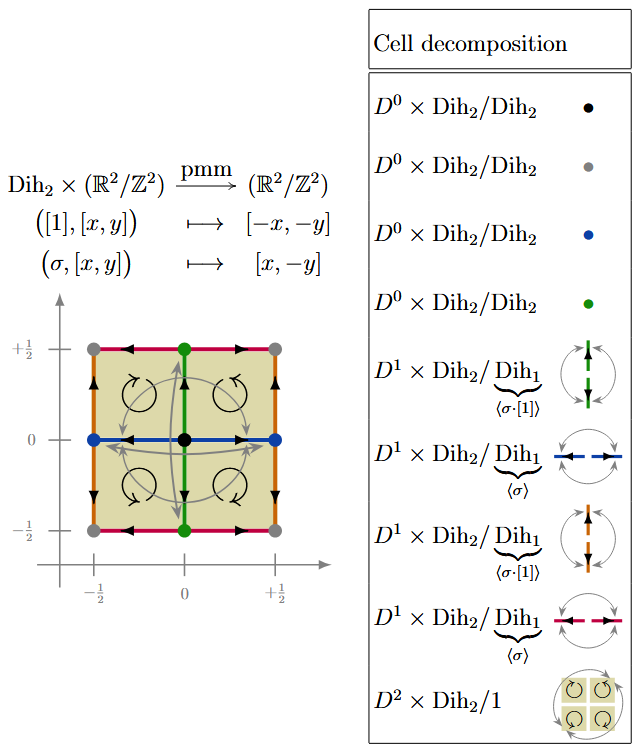
Example

(cmm) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with $Dih_{2}$-action according to the wallpaper group cmm:

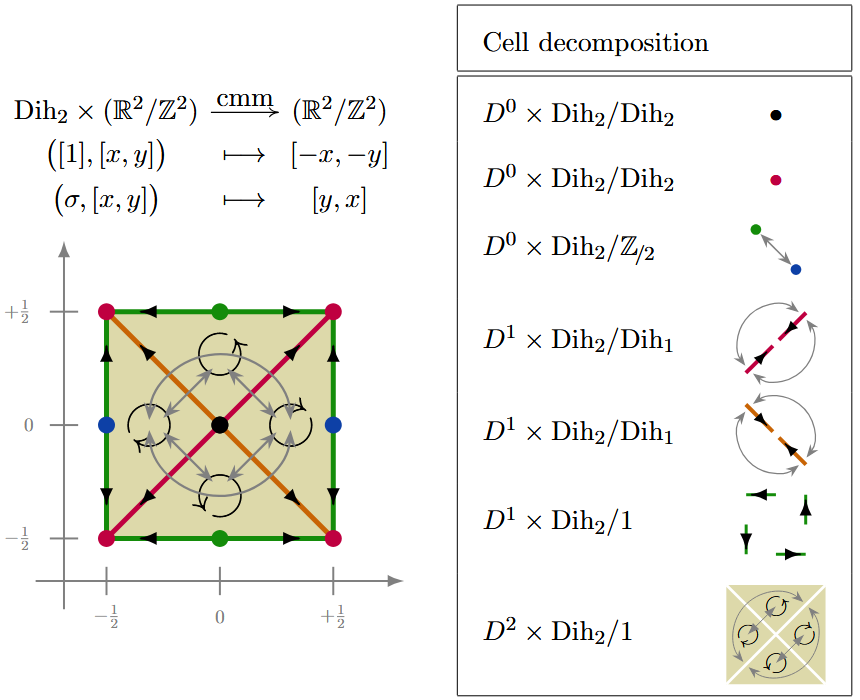
Example

(p4) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $\mathbb{Z}_{/4}$-action which rotates by multiples of $\pi/2$ around the origin:

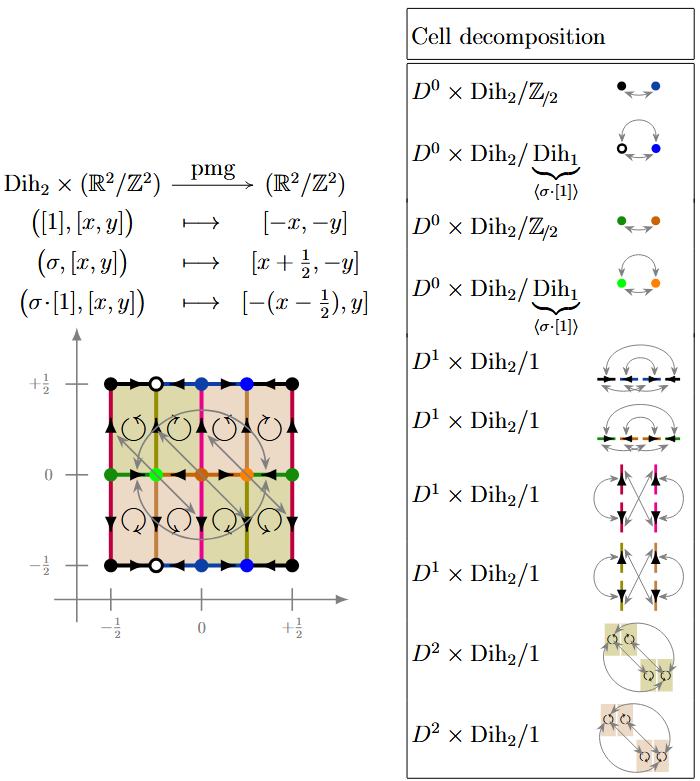
Example

(p4m) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with $Dih_{4}$-action:

Example

(p3) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $\mathbb{Z}_{/3}$-action which rotates by multiples of $2\pi/3$ around the origin:

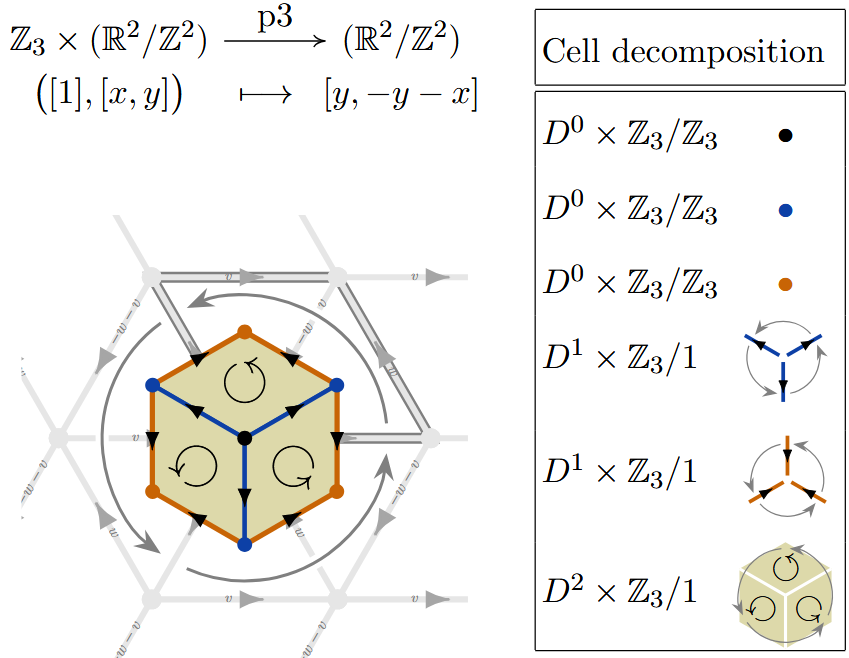
Example

(p31m) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with $Dih_{3}$-action corresponding to the $p31m$ wallpaper group:

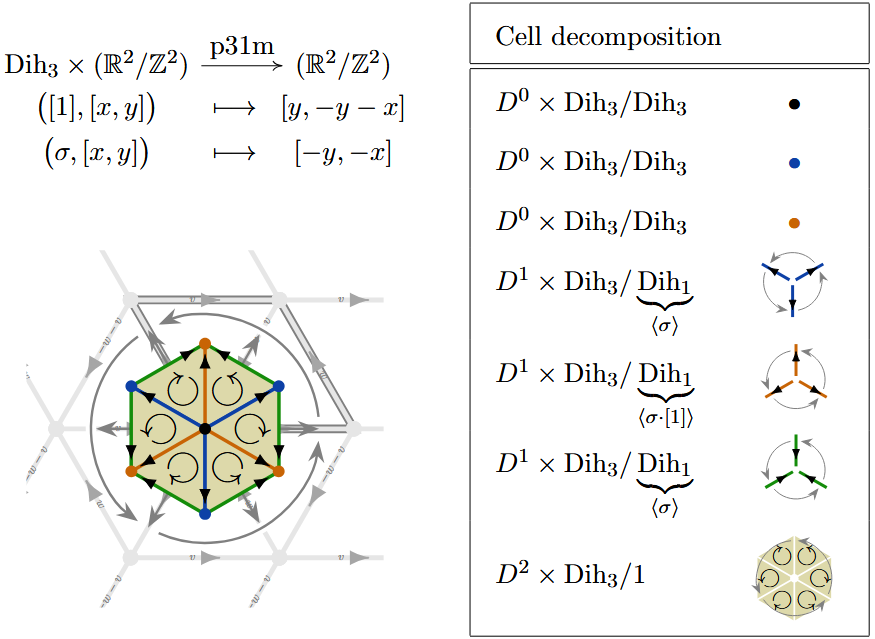
Example

(p3m1) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $Dih_{3}$-action corresponding to the $p3m1$ wallpaper group:

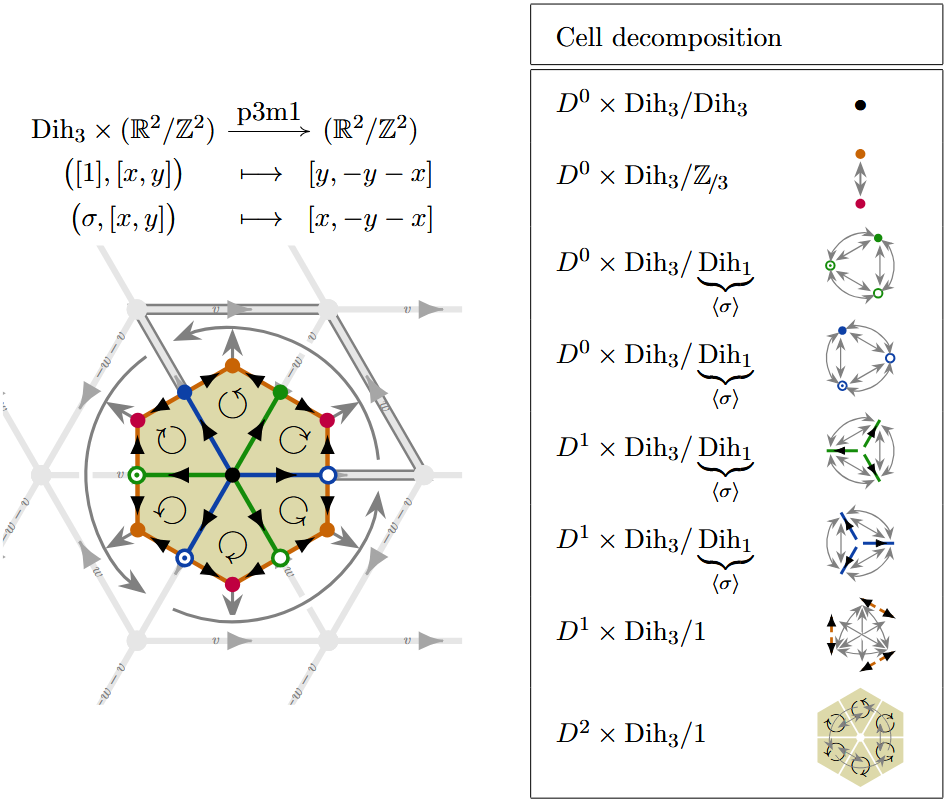
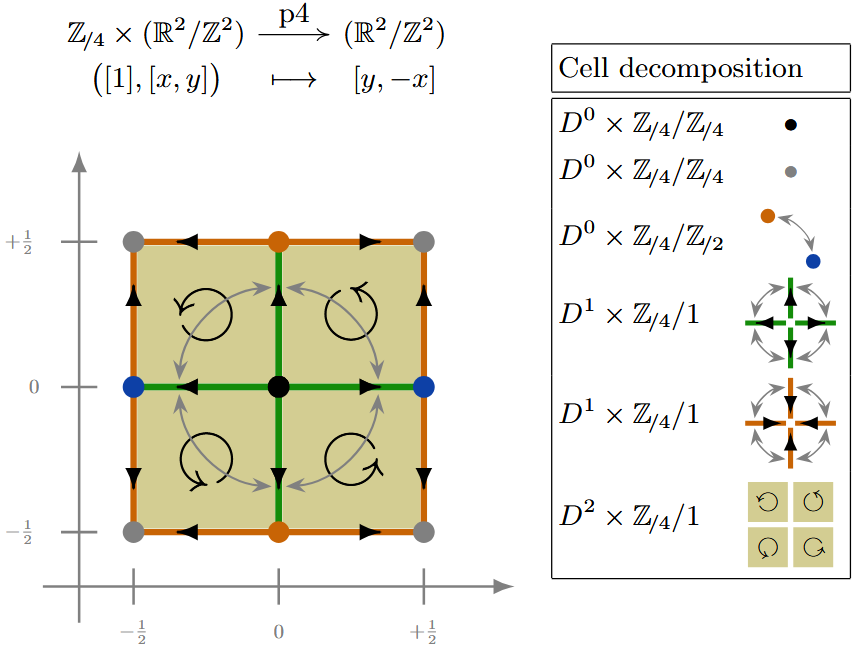
Example

(p6) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with the $\mathbb{Z}_{/6}$-action which rotates by multiples of $\pi/3$ around the origin:

Example

(p6m) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with $Dih_{6}$-action:

This completes the list of the symmorphic cases. One of the remaining 4 non-symmorphic cases follows:

Example

(pg) Here is a $G$-CW complex structure for the torus $T^2 \,\equiv\, \mathbb{R}^2/\mathbb{Z}^2$ equipped with glide reflection action corresponding to the wallpaper group $p g$:

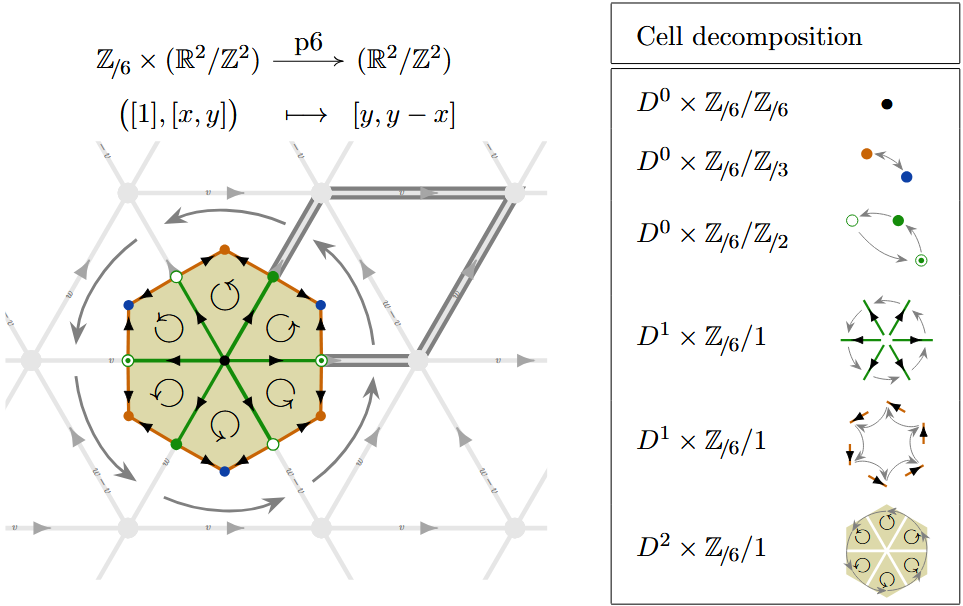
Related concepts

• flat orbifold

• finite rotation group

• tesselation

References

Review:

• Doris Schattschneider: The plane symmetry groups and their recognition, American Mathematical Monthly 85 (1978) 439-450 [pdf, pdf]

• Mark A. Armstrong, chapters 25-26 of: Groups and Symmetry, Undergraduate Texts in Mathematics, Springer (1988) [doi:10.1007/978-1-4757-4034-9, pdf]

• Patrick Morandi: The Classification of Wallpaper Patterns: From Group Cohomology to Escherís Tessellations (2003) [pdf, pdf]

• John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss: The Symmetries of Things, CRC Press (2008) [ISBN:9781568812205]

• Julija Zavadlav: Wallpaper Groups (2012) [pdf, pdf]

• Christopher Hammond, chapter 2 of: The Basics of Crystallography and Diffraction, Oxford University Press (2015) [ISBN:9780198738688]

• Richard Tilley, Sec. 3.5 in: Crystals and Crystal Structure, Wiley (2020) [ISBN:978-1-119-54838-6]

• Angela Zhao: A Brief Survey on Wallpaper Groups, MIT Mathematics (2023) [pdf]

See also:

• Wikipedia, Wallpaper group

Discussion of meromorphic functions on the complex plane invariant under wallpaper groups:

• Richard Chapling, Invariant Meromorphic Functions on the Wallpaper Groups (arXiv:1608.05677)