nLab 7-sphere

Idea

The sphere of dimension 7.

This is one of the parallelizable spheres, as such corresponds to the octonions among the division algebras, being the manifold of unit octonions, and is the only one of these which does not carry (Lie) group structure but just Moufang loop structure.

Properties

Quaternionic Hopf fibration

The 7-sphere participates in the quaternionic Hopf fibration, the analog of the complex Hopf fibration with the field of complex numbers replaced by the division ring of quaternions or Hamiltonian numbers $\mathbb{H}$.

$$\array{ S^3 &\hookrightarrow& S^7 \\ && \downarrow^\mathrlap{p} \\ && S^4 &\stackrel{}{\longrightarrow}& \mathbf{B} SU(2) }$$

Here the idea is that $S^7$ can be construed as $\{(x, y) \in \mathbb{H}^2: {|x|}^2 + {|y|}^2 = 1\}$, with $p$ mapping $(x, y)$ to $x/y$ as an element in the projective line $\mathbb{P}^1(\mathbb{H}) \cong S^4$, with each fiber a torsor parametrized by quaternionic scalars $\lambda$ of unit norm (so $\lambda \in S^3$). This canonical $S^3$-bundle (or $SU(2)$-bundle) is classified by a map $S^4 \to \mathbf{B} SU(2)$.

Coset space realizations

Proposition

(coset space of Spin(7) by G₂ is 7-sphere)

Consider the canonical action of Spin(7) on the unit sphere in $\mathbb{R}^8$ (the 7-sphere),

1. This action is is transitive;

2. the stabilizer group of any point on $S^7$ is G₂;

3. all G₂-subgroups of Spin(7) arise this way, and are all conjugate to each other.

Hence the coset space of Spin(7) by G₂ is the 7-sphere

$$S^7 \;\simeq_{diff}\; Spin(7)/G_2 \,.$$

(e.g Varadarajan 01, Theorem 3)

Other coset realizations of the usual differentiable 7-sphere (Choquet-Bruhat, DeWitt-Morette 00, p. 288):

• $S^7 \simeq_{diff}$ Spin(6)$/SU(3) \simeq_{iso} SU(4)/SU(3)$ (by this Prop.);

• $S^7 \simeq_{diff} Spin(5)/SU(2)$ (Awada-Duff-Pope 83, Duff-Nilsson-Pope 83)

These three coset realizations of ‘squashed’ 7-spheres together with a fourth

• $S^7 \simeq_{diff} Spin(8)/Spin(7)$,

the realization of the ‘round’ 7-sphere, may be seen jointly as resulting from the 8-dimensional representations of even Clifford algebras in 5, 6, 7, and 8 dimensions (see Baez) and as such related to the four normed division algebras. See also Choquet-Bruhat+DeWitt-Morette00, pp. 263-274.

coset space-structures on n-spheres:

standard:
$S^{n-1} \simeq_{diff} SO(n)/SO(n-1)$	this Prop.
$S^{2n-1} \simeq_{diff} SU(n)/SU(n-1)$	this Prop.
$S^{4n-1} \simeq_{diff} Sp(n)/Sp(n-1)$	this Prop.
exceptional:
$S^7 \simeq_{diff} Spin(7)/G_2$	Spin(7)/G₂ is the 7-sphere
$S^7 \simeq_{diff} Spin(6)/SU(3)$	since Spin(6) $\simeq$ SU(4)
$S^7 \simeq_{diff} Spin(5)/SU(2)$	since Sp(2) is Spin(5) and Sp(1) is SU(2), see Spin(5)/SU(2) is the 7-sphere
$S^6 \simeq_{diff} G_2/SU(3)$	G₂/SU(3) is the 6-sphere
$S^15 \simeq_{diff} Spin(9)/Spin(7)$	Spin(9)/Spin(7) is the 15-sphere

see also Spin(8)-subgroups and reductions

homotopy fibers of homotopy pullbacks of classifying spaces:

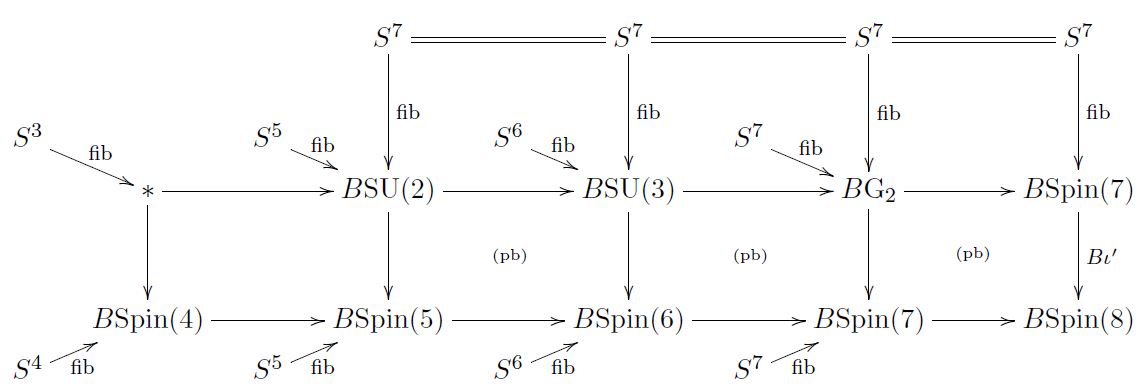
(from FSS 19, 3.4)

The following gives an exotic 7-sphere:

• $S^7 \simeq_{homeo} Sp(1)\backslash Sp(2)/Sp(1)$ (Gromoll-Meyer sphere)

Exotic 7-spheres

A celebrated result of Milnor is that $S^7$ admits exotic smooth structures (see at exotic 7-sphere), i.e., there are smooth manifold structures on the topological manifold $S^7$ that are not diffeomorphic to the standard smooth structure on $S^7$. More structurally, considering smooth structures up to oriented diffeomorphism, the different smooth structures form a monoid under a (suitable) operation of connected sum, and this monoid is isomorphic to the cyclic group $\mathbb{Z}/(28)$. With the notable possible exception of $n = 4$ (where the question of existence of exotic 4-spheres is wide open), exotic spheres first occur in dimension $7$. This phenomenon is connected to the h-cobordism theorem (the monoid of smooth structures is identified with the monoid of h-cobordism classes of oriented homotopy spheres).

One explicit construction of the smooth structures is given as follows (see Milnor 1968). Let $W_k$ be the algebraic variety in $\mathbb{C}^5$ defined by the equation

$$z_1^{6 k - 1} + z_2^3 + z_3^2 + z_4^2 + z_5^2 = 0$$

and $S_\epsilon \subset \mathbb{C}^5$ a sphere of small radius $\epsilon$ centered at the origin. Then each of the $28$ smooth structures on $S^7$ is represented by an intersection $W_k \cap S_\epsilon$, as $k$ ranges from $1$ to $28$. These manifolds sometimes go by the name Brieskorn manifolds or Brieskorn spheres or Milnor spheres.

$G_2$-structure

Let $\phi_0 \in \Omega^3(\mathbb{R}^7)$ be the associative 3-form and let

$$\Phi_0 \in \Omega^4(\mathbb{R} \oplus \mathbb{R}^7)$$

be given by

$$\Phi_0 = d x_0 \wedge \phi_0 + \star \phi_0$$

(where $x_0$ denotes the canonical coordinate on the first factor of $\mathbb{R}$ and $\phi_0$ is pulled back along the projection to $\mathbb{R}^7$) .

By construction this is its own Hodge dual

$$\Phi = \star \Phi \,.$$

This implies that as we restrict $\Phi_0$ to

$$\mathbb{R}^8 - \{0\} \simeq \mathbb{R} \times S^7$$

then there is a unique 3-form

$$\phi \in \Omega^3(S^7)$$

on the 7-sphere such that

$$\Phi_0 = r^3 \wedge \phi + r^4 \star_{S^7} \phi \;\;\;\; (on \; \mathbb{R}^8 - \{0\}) \,.$$

This 3-form $\phi$ defines a G₂-structure on $S^7$. It is nearly parallel in that

$$d \phi = 4 \star \phi \,.$$

(e.g. Lotay 12, def.2.4)

Related concepts

n-sphere

• unit sphere, polar coordinates

• round sphere, squashed sphere

• hemisphere, equator

• stereographic projection

• homotopy groups of spheres

• homotopy sphere, rational homotopy sphere, Cohomotopy

• spherical fibration, twisted Cohomotopy

• sphere spectrum, stable Cohomotopy theory

• homology sphere

• rational homotopy sphere, rational n-sphere

• motivic sphere

• group actions on spheres

• representation sphere, equivariant Cohomotopy

• Reeb sphere theorem

• sphere packing

• Music of the Spheres

low dimensional n-spheres

• circle

  • real projective space$\,\mathbb{R}P^1$

• 2-sphere

  • complex projective line$\,\mathbb{C}P^1$: Riemann sphere

  • geometric quantization of the 2-sphere

  • fuzzy 2-sphere

• 3-sphere

  • fuzzy 3-sphere

• 4-sphere

  • quaternionic projective line$\,\mathbb{H}P^1$

  • fuzzy 4-sphere

• 5-sphere

• 6-sphere

• 7-sphere

  • exotic 7-sphere

• 8-sphere

  • octonionic projective line$\,\mathbb{O}P^1$

• 13-sphere

• 15-sphere

• infinite-dimensional sphere

References

• Martin Cederwall, Christian R. Preitschopf, The Seven-sphere and its Kac-Moody Algebra, Commun. Math. Phys. 167 (1995) 373-394 (arXiv:hep-th/9309030)

• Takeshi Ôno, On the Hopf fibration $S^7 \to S^4$ over $Z$, Nagoya Math. J. Volume 59 (1975), 59-64. (Euclid)

Relation to the Milnor fibration:

• Kenneth Intriligator, Hans Jockers, Peter Mayr, David Morrison, M. Ronen Plesser, Conifold Transitions in M-theory on Calabi-Yau Fourfolds with Background Fluxes, Adv.Theor.Math.Phys. 17 (2013) 601-699 (arXiv:1203.6662)

An ADE classification of finite subgroups of $SO(8)$ acting freely on $S^7$ (see at group action on an n-sphere) such that the quotient is spin and has at least four Killing spinors (see also at ABJM model) is in

• Paul de Medeiros, José Figueroa-O'Farrill, Sunil Gadhia, Elena Méndez-Escobar, Half-BPS quotients in M-theory: ADE with a twist, JHEP 0910:038,2009 (arXiv:0909.0163, pdf slides)

• Paul de Medeiros, José Figueroa-O'Farrill, Half-BPS M2-brane orbifolds (arXiv:1007.4761)

Discussion of subgroups:

• Veeravalli Varadarajan, Spin(7)-subgroups of SO(8) and Spin(8), Expositiones Mathematicae, 19 (2001): 163-177 (pdf)

Discussion of exotic smooth structures on 7-spheres includes

• Wikipedia, Exotic sphere, link.

The explicit construction of exotic 7-spheres by intersecting algebraic varieties with spheres is described in

• John Milnor, “Singular points of complex hypersurfaces” , Princeton Univ. Press (1968).

Discussion of (nearly) G2-structures on $S^7$ and calibrated submanifolds includes

• Jason Lotay, Associative Submanifolds of the 7-Sphere, Proc. London Math. Soc. (2012) 105 (6): 1183-1214 (arXiv:1006.0361, talk slides)

On coset-realizations:

• Linus Kramer, Octonion Hermitian quadrangles, Bull. Belg. Math. Soc. Simon Stevin Volume 5, Number 2/3 (1998), 353-362 (euclid:1103409015)

• Yvonne Choquet-Bruhat, Cécile DeWitt-Morette, Analysis, manifolds and physics, Part II, North Holland (1982, 2001) $[$ISBN:9780444860170$]$

• Moustafa A. Awada, Mike Duff, Christopher Pope, $N=8$ Supergravity Breaks Down to $N=1$, Phys. Rev. Lett. 50 5 (1983) 294-297 [doi:10.1103/PhysRevLett.50.294]

• Mike Duff, Bengt Nilsson, Christopher Pope, Spontaneous Supersymmetry Breaking by the Squashed Seven-Sphere, Phys. Rev. Lett. 50, 2043 – Published 27 June 1983; Erratum Phys. Rev. Lett. 51, 846 (doi:10.1103/PhysRevLett.50.2043)

• John Baez, Rotations in the 7th Dimension, (blog post), and TWF 195, (webpage)