nLab Rozansky-Witten theory
Context
Algebraic Quantum Field Theory
algebraic quantum field theory (perturbative, on curved spacetimes, homotopical)
Concepts
quantum mechanical system, quantum probability
interacting field quantization
Theorems
States and observables
Operator algebra
Local QFT
Perturbative QFT
Knot theory
Examples/classes:
Types
Related concepts:
Contents
Idea
What is called Rozansky-Witten theory (Rozansky-Witten 96) is a topological twist of D=3 N=4 super Yang-Mills theory, or else the assignment of topological invariant quantum observables which such a perturbative quantum field theory assigns to a given 3-manifold , or more generally to a Wilson loop knot inside a 3-manifold – the Rozansky-Witten invariants.
The key result of Rozansky-Witten 96 is that, after gauge fixing and some subtle field identifications, the Feynman rules of RW-twisted D=3 N=4 super Yang-Mills theory are those of perturbative Chern-Simons theory, in that the only relevant propagator is the Chern-Simons propagator and the only relevant Feynman diagrams are trivalent, except that the Lie algebra weights assigned by Chern-Simons theory to a Feynman-Jacobi diagram, are replaced by other weight systems, now called Rozansky-Witten weight systems.
Precisely: Recalling that perturbative Chern-Simons Wilson loop observables are given by evaluating Lie algebra weight systems on the Jacobi diagram-valued universal Vassiliev invariant
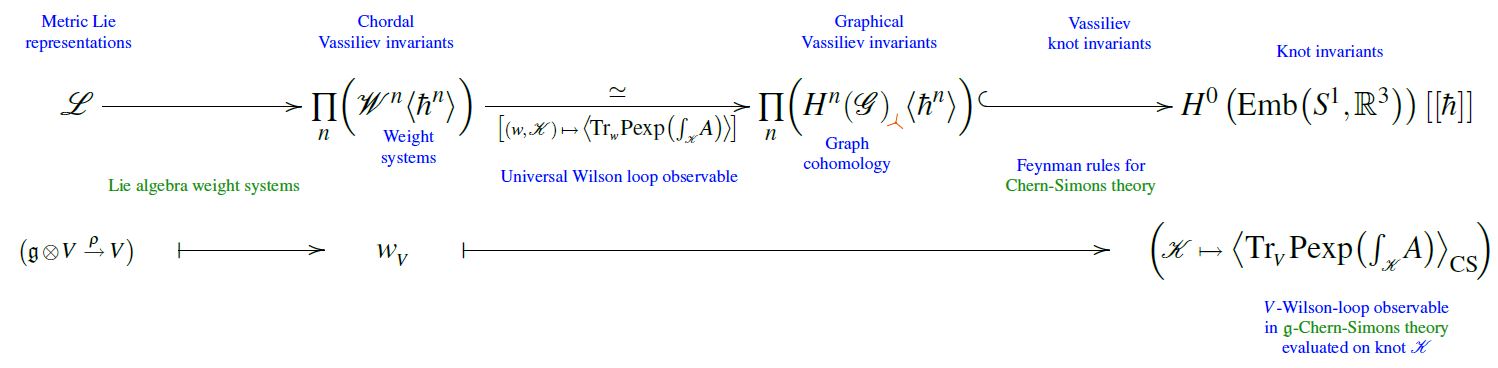
we have that Wilson loop Rozansky-Witten invariants (Rozansky-Witten 96 (2.26)) are given by evaluating Rozansky-Witten invariants on the Jacobi diagram-valued universal Vassiliev invariant
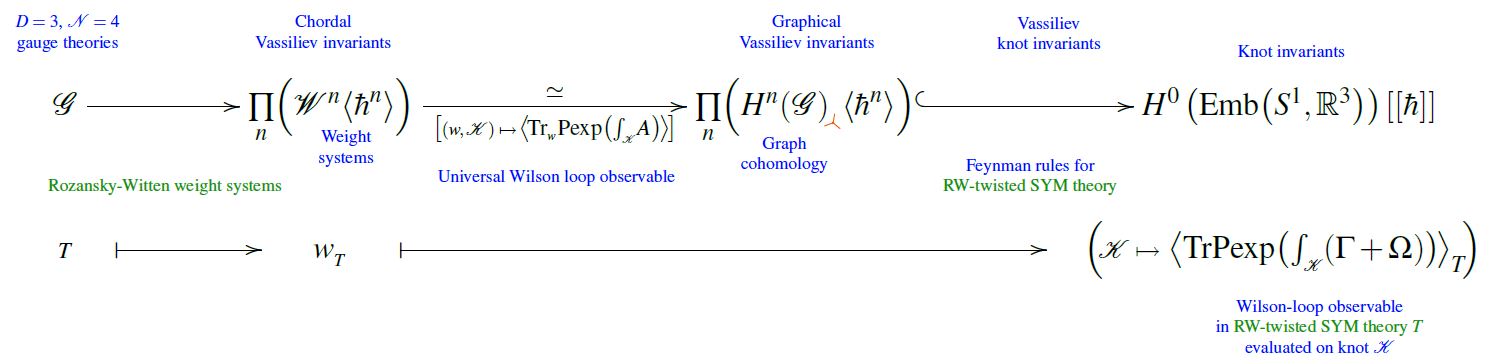
graphics from Sati-Schreiber 19c
These Rozansky-Witten weight systems depend only on the hyperkähler manifold which is the (classical) Coulomb branch of the RW-twisted D=3 N=4 super Yang-Mills theory, and in fact they are independent of the Riemannian geometry of and depend only on the underlying holomorphic symplectic manifold (Kapranov 99). Generally, they are defined for any hyperkähler manifold which is either asymptotically flat (ALE spaces) or compact topological space (compact hyperkähler manifolds).
Via the equivalent reformulation by Kapranov 99 one finds (Roberts-Willerton 10) that the Rozansky-Witten invariants are structurally Lie algebra weight systems themselves, but internal to the derived category of coherent sheaves of and composed with an integration over which makes the resulting Dolbeault cohomology-valued weights become ground field-valued.
Konsevich 97 gives a formulation of Rozansky-Witten invariants via characteristic classes of foliations and Gelfand-Fuks cohomology. He devised a formal construction, again depending on a trivalent graph of a cohomology class of the Lie algebra of formal (in the sense of formal power series) Hamiltonian vector fields on any arbitrary finite-dimensional symplectic vector space. Characteristic classes of foliations may induce examples where this construction applies; one of the examples yields Rozansky-Witten .
Properties
As KK-compactification of M5-brane worldvolume
Rozansky-Witten theory may be identified with topologically twisted KK-compactification of the D=6 N=(2,0) SCFT on the M5-brane (Gukov-Putrov-Vafa 17, Sections 3.2 and 4.2)
Examples
Rozanzky-Witten Wilson loop of unknot is -genus
Proposition
(Rozansky-Witten Wilson loop of unknot is square root of A-hat genus)
For a hyperkähler manifold (or just a holomorphic symplectic manifold) the Rozansky-Witten invariant Wilson loop observable associated with the unknot in the 3-sphere is the square root of the A-hat genus of .
This is Roberts-Willerton 10, Lemma 8.6, using the Wheels theorem and the Hitchin-Sawon theorem.
Related concepts
References
Original articles:
-
Lev Rozansky, Edward Witten, Hyper-Kähler geometry and invariants of 3-manifolds, Selecta Math., New Ser. 3 (1997), 401–458 (arXiv:hep-th/9612216, doi:10.1007/s000290050016, MR98m:57041)
-
Maxim Kontsevich, Rozansky–Witten invariants via formal geometry, Compositio Mathematica 115: 115–127, 1999, doi, arXiv:dg-ga/9704009, MR2000h:57057
-
Mikhail Kapranov, Rozansky–Witten invariants via Atiyah classes, Compositio Math. 115 (1999), no. 1, 71–113 (MR2000h:57056, doi, alg-geom/9704009)
-
Justin Roberts, Justin Sawon, Generalisations of Rozansky-Witten invariants, Geom. Topol. Monogr. 4 (2002) 263-279 (arXiv:math/0112210)
Unified description of Rozansky-Witten weight systems as Lie algebra weight systems for Lie algebra objects in the derived category of quasi-coherent sheaves, and unified Wheels theorem:
- Justin Roberts, Simon Willerton, On the Rozansky-Witten weight systems, Algebr. Geom. Topol. 10 (2010) 1455-1519 (arXiv:math/0602653)
Review:
-
Justin Sawon, Rozansky-Witten invariants of hyperkähler manifold, Cambridge 2000 (arXiv:math/0404360)
-
Justin Roberts, Rozansky-Witten theory (arXiv:math/0112209)
Further relation to Chern-Simons theory:
-
Anton Kapustin, Natalia Saulina, Chern-Simons-Rozansky-Witten topological field theory, Nucl. Phys. B823 (2009) 403-427 (arXiv:0904.1447, spire:817599/)
-
Jian Qiu, Maxim Zabzine, Odd Chern-Simons theory, Lie algebra cohomology and characteristic classes, Commun. Math. Phys. 300:789-833, 2010 (arxiv/0912.1243)
As topologically twisted KK-compactification of the D=6 N=(2,0) SCFT on the M5-brane (see D=3 N=4 super Yang-Mills theory and 3d-3d correspondence):
-
Sergei Gukov, Pavel Putrov, Cumrun Vafa, Sections 3.2 and 3.4 of: Fivebranes and 3-manifold homology, J. High Energ. Phys. (2017) 2017: 71 (arXiv:1602.05302)
-
Cyril Closset, Heeyeon Kim, Section 6.1 of: Comments on twisted indices in 3d supersymmetric gauge theories, JHEP 08 (2016) 059 (arXiv:1605.06531)
Discussion of Rozansky-Witten theory as a boundary field theory:
- Anton Kapustin, Lev Rozansky, Natalia Saulina, Three-dimensional topological field theory and symplectic algebraic geometry I (arXiv:0810.5415)
Relation to equivariant cohomology:
-
Sergei Gukov, Po-Shen Hsin, Hiraku Nakajima, Sunghyuk Park, Du Pei, Nikita Sopenko, Rozansky-Witten geometry of Coulomb branches and logarithmic knot invariants (arXiv:2005.05347)
-
Jian Qiu, Rozansky-Witten theory, Localised then Tilted (arXiv:2011.05375)
Discussion of Rozansky-Witten defect QFT as an extended TQFT via the cobordism hypothesis:
- Ilka Brunner, Nils Carqueville, Daniel Roggenkamp, Truncated affine Rozansky–Witten models as extended TQFTs, Commun. Math. Phys. (2023) [arXiv:2201.03284, doi:10.1007/s00220-022-04614-4]
reviewed in:
- Nils Carqueville, Extended defect TQFTs, talk at QFT and Cobordism, CQTS (Mar 2023) [web, pdf, pdf, video:YT]
Relation to super Chern-Simons theory:
- Nathan Geer, Matthew B. Young, Three dimensional topological quantum field theory from and Chern–Simons theory [arXiv:2210.04286]
Review:
- Matthew B. Young, Non-semisimple TFT and Chern-Simons theory, talk at QFT and Cobordism, CQTS (Mar 2023)[web]
On Rozansky-Witten theory as extended functorial field theory:
- Lorenzo Riva, Higher categories of push-pull spans, I: Construction and applications [arXiv:2404.14597]
On line defects in Rozansky-Witten theory and their braiding:
- Sergei Gukov, Babak Haghighat, Nicolai Reshetikhin: Foams and KZ-equations in Rozansky-Witten theories [arXiv:2407.19757]
Last revised on July 30, 2024 at 07:38:24. See the history of this page for a list of all contributions to it.