nLab metric Lie representation
Context
Lie theory
∞-Lie theory (higher geometry)
Background
Smooth structure
Higher groupoids
Lie theory
∞-Lie groupoids
∞-Lie algebroids
Formal Lie groupoids
Cohomology
Homotopy
Related topics
Examples
-Lie groupoids
-Lie groups
-Lie algebroids
-Lie algebras
Contents
Idea
Given a Lie algebra and a Lie algebra representation , and given non-degenerate inner products, hence “metrics”, both on and on , one may ask that all structure is compatible with these metrics. For the Lie algebra this means to have a metric Lie algebra and for the representation this means to have an orthogonal representation. Hence together this is an orthogonal representation of a metric Lie algebra, or metric Lie representation, for short.
Definition
The following table shows the data in a metric Lie representation equivalently
-
in category theory-notation;
-
in index notation:
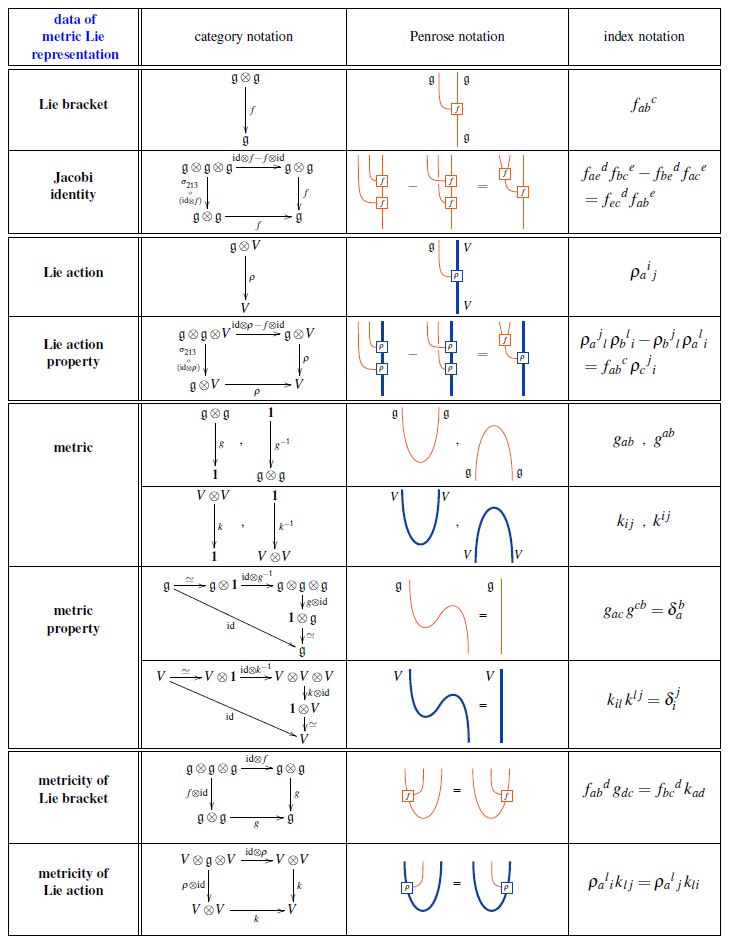
graphics from Sati-Schreiber 19c
Properties
Structures induced from metric Lie representations
The following mathematical structures are induced from the data of metric Lie representations:
-
…
See there for more.
Related concepts
References
On the Faulkner construction (which became known as the M2-brane 3-algebra constructed from a metric Lie representation):
- John Faulkner, On the geometry of inner ideals, Journal of Algebra, Volume 26, Issue 1, July 1973, Pages 1-9 (doi:10.1016/0021-8693(73)90032-X)
Last revised on December 28, 2019 at 23:49:16. See the history of this page for a list of all contributions to it.