nLab
brane

Idea

The term brane in formal high energy physics, and in particular in string theory, refers to entities that one thinks of as physical objects that generalize the notion point particles to higher dimensional objects.

The term derives from the word membrane that was originally used to describe 2-dimensional “particles”. When the need was felt to speak also about 3-, 4- and higher dimensional such “particles” the usage “3-brane”, “4-brane” etc. was introduced. Ordinary particles would be 0-branes in this counting, the strings in string theory would be 1-branes and membranes themselves 2-branes.

There are two fundamentally different concrete realizations of this somewhat vague notion.

1. D-branes and other boundary conditions

2. fundamental or $\sigma$-model branes or NS-branes

Boundary conditions or D-branes

In terms of the algebraic data of the QFT on the worldvolume

An abstractly defined $n$-dimensional quantum field theory is a consistent assignment of state-space and correlators to $n$-dimensional cobordisms with certain structure (topological structure, conformal structure, Riemannian structure, etc. see FQFT/AQFT). In an open-closed QFT the cobordisms are allowed to have boundaries.

In this abstract formulation of QFT a brane is a type of data assigned by the QFT to boundaries of cobordisms.

In $2d$ rational CFT

A well understood class of examples is this one: among all 2-dimensional conformal field theory that case of full rational 2d CFT has been understood completely, using FFRS-formalism. It is then a theorem that full 2-rational CFTs are classified by

1. a modular tensor category $𝒞$ (to be thought of as being the category of representaitons of the vertex operator algebra of the 2d CFT);

2. a special symmetric Frobenius algebra object $A$ internal to $𝒞$.

In this formulation a type of brane of the theory is precisely an $A$-module in $𝒞$ (an $A$-bimodule is a bi-brane or defect line ):

the 2d cobordisms with boundary on which the theory defined by $A\in 𝒞$ carry as extra structure on their connected boundary pieces a label given by an equivalence class of an $A$-module in $𝒞$. The assignment of the CFT to such a cobordism with boundary is obtained by

• triangulating the cobordism,

• labeling all internal edges by $A$

• labelling all boundary pieces by the $A$-module

• all vertices where three internal edges meet by the multiplication operation

• and all points where an internal edge hits a moundary by the corresponding action morphism

• and finally evaluating the resulting string diagram in $𝒞$.

So in this abstract algebraic formulation of QFT on the worldvolume, a brane is just the datum assigned by the QFT to the boundary of a cobordism. But abstractly defined QFTs may arise from quantization of sigma models. This gives these boundary data a geometric interpretation in some space. This we discuss in the next section.

In $2d$ TFT

Another case where the branes of a QFT are under good mathematical control is TCFT: the (infinity,1)-category-version of a 2d TQFT.

Particularly the A-model and the B-model are well understood.

• the branes of the B-model (“B-branes”) form the the stable (infinity,1)-category of chain complexes of quasicoherent sheaves on the target space (often considered just in terms of its homotopy category of an (infinity,1)-category, the derived category of quasicoherent sheaves);

• the branes of the A-model form the Fukaya category of the target space.

(…)

In terms of geometric data of the $\sigma$-model background

An abstractly defined QFT (as a consistent assignment of state spaces and propagators to cobordisms as in FQFT) may be obtained by quantization from geometric data :

Sich a sigma-model QFT is the quantization of an action functional on a space of maps $\Sigma \to X$ from a cobordims (“worldvolume”) $\Sigma$ to some target space $X$ that may carry further geoemtric data such as a Riemannian metric, or other background gauge fields.

One may therefore try to match the geometric data on $X$ that encodes the $\sigma$-model with the algebraic data of the FQFT that results after quantization. This gives a geometric interpretation to many of the otherwise purely abstract algebraic properties of the worldvolume QFT.

It turns out that if one checks which geometric data corresponds to the $A$-modules in the above discussion, one finds that these tend to come from structures that look at least roughly like submanifolds of the target space $X$. And typically these submanifolds themselves carry their own background gauge field data.

A well-understood case is the Wess-Zumino-Witten model: for this the target space $X$ is a simple Lie group $X=G$ and the background field is a circle 2-bundle with connection (a bundle gerbe) on $G$, representing the background field that is known as the Kalb-Ramond field.

In this case it turns out that branes for the sigma model on $X$ are given in the smplest case by conjugacy classes $D\subset G$ inside the group, and that these carry twisted vector bundle with the twist given by the Kalb-Ramond background bundle. These vector bundles are known in the string theory literature as Chan-Paton vector bundles . The geometric intuition is that a QFT with certain boundary condition comes form a quantization of spaces of maps $\Sigma \to G$ that are restricted to take the boundary of $\Sigma$ to these submanifolds.

More generally, one finds that the geometric data that corresponds to the branes in the algebraically defined 2d QFT is given by cocycles in the twisted differential K-theory of $G$. These may be quite far from having a direct interpretation as submanifolds of $G$.

The case of rational 2d CFT considered so far is only the best understood of a long sequence of other examples. Here the collection of all D-branes – identified with the colleciton of all internal modules over an internal frobenius algebra, forms an ordinary category.

More generally, at least for 2-dimensional TQFTs analogous considerations yield not just categories but stable (∞,1)-categories of boundary condition objects. For instance for what is called the B-model 2-d TQFT the category of D-branes is the derived category of coherent sheaves on some Calabi-Yau space.

Starting with Kontsevich’s homological algebra reformulation of mirror symmetry the study of (derived) D-brane categories has become a field in its own right in pure mathematics.

… lots of further things to say …

Fundamental or $\sigma$-model branes

In string theory one speaks apart from the D-branes also about fundamental branes . These are the objects $\Sigma$ in the $n$-dimensional sigma model themselves.

• For $n=0$ this describes the ordinary quantum mechanics of a point particles on $X$. And such point particles are the fundamental particles for instance of the standard model of particle physics.

• For $n=1$ this describes the quantum propagation of a string, and accordingly one speaks of the fundamental string or F1-brane (fundamental 1-brane).

• For $n=2$ this describes the quantum propagation of a membrane.

• There are good indications that there is a way to describe heterotic string theory not in terms of fundamental 1-branes but in terms of the sigma-model of a fundamental 5-brane – the magnetic dual of the 1-brane in 10-dimensions.

• etc.

Black branes

See black brane .

Properties

Worldvolume theories

Table of branes appearing in supergravity/string theory

brane	in supergravity	charged under gauge field	has worldvolume theory
black brane	supergravity	higher gauge field	SCFT
D-brane	type II	RR-field	super Yang-Mills theory
$(D=2n)$	type IIA	$$	$$
D0-brane	$$	$$	BFSS matrix model
D2-brane	$$	$$	$$
D4-brane	$$	$$	Khovanov homology observables
$(D=2n+1)$	type IIB	$$	$$
D1-brane	$$	$$	2d CFT with BH entropy
D3-brane	$$	$$	N=4 D=4 super Yang-Mills theory
D5-brane	$$	$$	$$
D7-brane	$$	$$	$$
NS-brane	type I, II, heterotic	circle n-connection	$$
string	$$	B2-field	2d SCFT
NS5-brane	$$	B6-field	little string theory
M-brane	11D	circle n-connection	$$
M2-brane	$$	C3-field	ABJM theory, BLG model
M5-brane	$$	C6-field	6d (2,0)-superconformal QFT

The super-brane scan

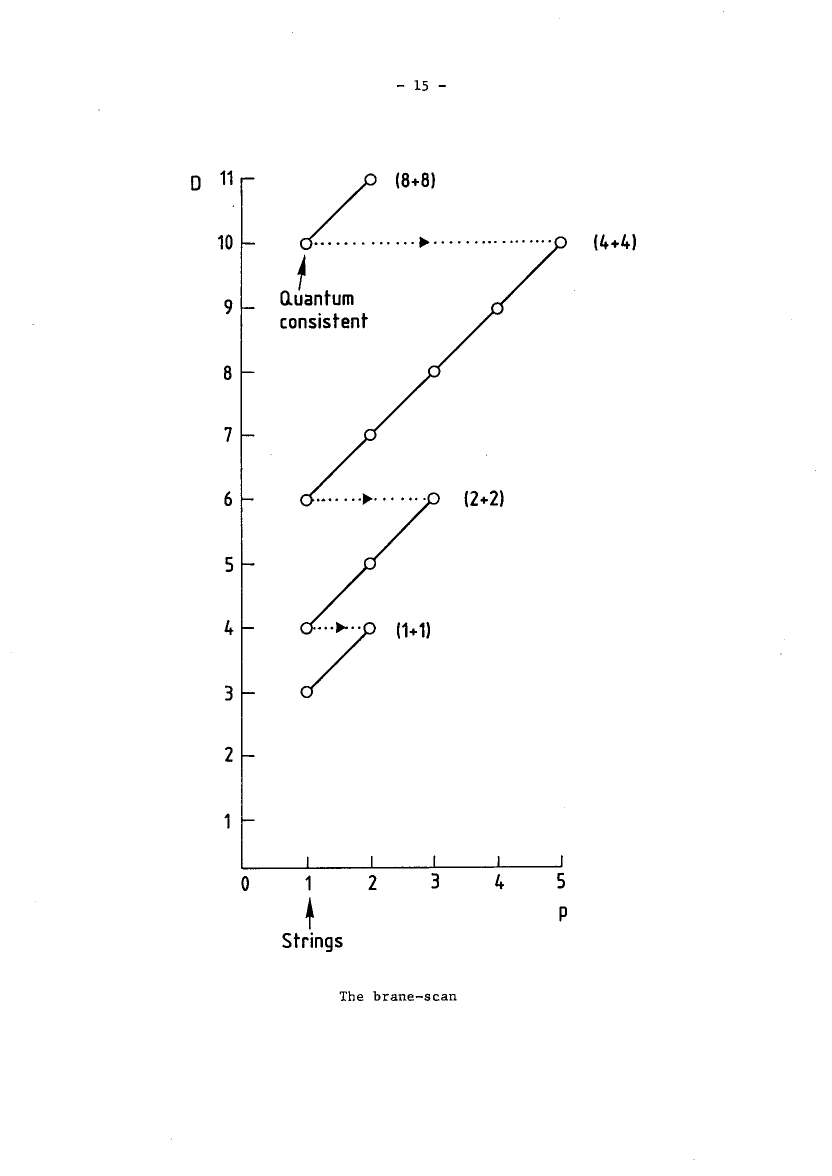
If the worldvolume QFT of the fundamental branes (for instance the worlsheet 2dCFT of the string) is required to be a supersymmetric QFT?, specifically if the Green-Schwarz action functional is used only particular combinations of the dimenion $\dim \Sigma =p+1$ of the worldvolume and $D=\dim X$ of spacetime are possible.

The corresponding table has been called the brane scan

Related concepts

• sigma-model

• particle, superparticle

• string

• membrane, M2-brane, M5-brane

• NS5-brane

• D-brane

References

General

• Joan Simon, Brane Effective Actions, Kappa-Symmetry and Applications (arXiv:1110.2422)

Boundary conditions / D-branes

(…)

See D-brane.

For exhaustive details on D-branes in 2-dimensional rational CFT see the references given at

• FFRS-formalism.

A classical text describing how the physics way to think of D-branes leads to seeing that they are objects in derived categories is

• Paul Aspinwall, D-Branes on Calabi-Yau Manifolds (arXiv)

This can to a large extent be read as a dictionary from homological algebra terminology to that of D-brane physics.

More recent similar material with the emphasis on the K-theory aspects is

• Richard Szabo, D-Branes and Bivariant K-Theory

Fundamental branes

The “brane scan” table showing the consistent dimension pairs for the Green-Schwarz action functional was depicted in

• Michael Duff, Supermembranes: the first fifteen weeks CERN-TH.4797/87 (scan)

going back to

• A. Achúcarro, J. M. Evans, Pete Townsend and D. L. Wiltshire, Super $p$-branes Physics Letters B Volume 198, Issue 4, 3 (1987)

Further developments are in

More along these lines is in

• Michael Duff, S. Ferrara, Four curious supergravities (arXiv)

See also division algebras and supersymmetry.