nLab Sen's conjecture

Idea

In bosonic string theory - The tachyon vacuum

The tachyon mode in the open bosonic string spectrum is an indication that its effective perturbative quantum field theory describes perturbation about an unstable vacuum which will decay via tachyon condensation.

It was argued in Sen 99, Section 4 that, since the open bosonic string technically propagates with its endpoints on the space-filling D25-brane, that instability must be the instability of the D25, which wants to decay to a “true bosonic string vacuum”, often called the tachyon vacuum.

This conjecture became famous as Sen’s conjecture. It was subsequently checked to be true by numerical means in open bosonic string field theory. Moreover, using string field theory it became possible to study that non-perturbative state in which the D25-brane is indeed gone. There were several arguments that indeed in the vicinity of this state the perturbative open string has disappeared and turned into the closed bosonic string.

A breakthrough happened in 2005, when Martin Schnabl found an analytic expression for this “tachyon vacuum” (Schnabl 05).

In superstring theory – The K-theory conjecture

Sen’s conjecture actually originates in superstring theory (Sen 98). While here the tachyon mode for open superstrings on supersymmetric D-brane-configurations is famously absent, signifying the stability of perturbative superstring vacua, there are still tachyon modes in open superstrings stretching between D-branes and anti-D-branes.

from Sch 18

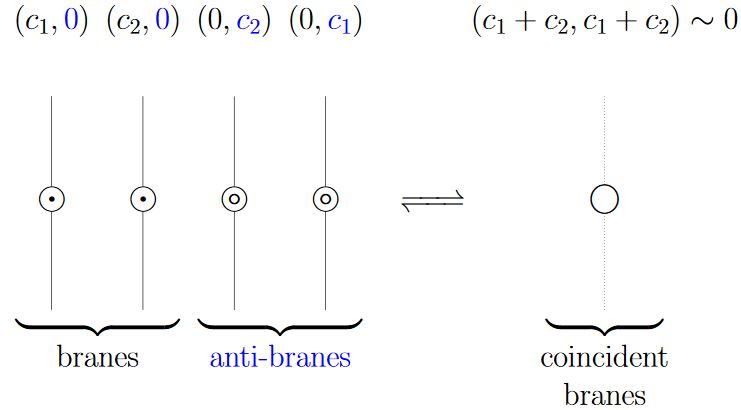
Here the conjecture states that the respective tachyon condensation witnesses the annihilation of the brane/anti-brane pair (Sen 98).

This conjectured process is one motivations for the further conjecture that D-brane charge is charge quantized in topological K-theory (Witten 98, Section 3), since this decay plausibly implements on the D-branes’s Chan-Paton vector bundles the defining equivalence relation (here) of topological K-theory.

References

General

Review:

• Ashoke Sen, Tachyon Dynamics in Open String Theory, Int. J. Mod. Phys. A20:5513-5656, 2005 (arXiv:hep-th/0410103)

For more references in the context of string field theory, see there at:

• Tachyon dynamics, decaying D-branes and Sen’s conjecture

For superstrings

Sen’s conjecture on the decay of D-brane/anti-D-brane pairs in superstring theory via open superstring tachyon condensation:

• Ashoke Sen, Tachyon Condensation on the Brane Antibrane System, JHEP 9808 012 (1998) [arXiv:hep-th/9805170, doi:10.1088/1126-6708/1998/08/012]

• Ashoke Sen, Universality of the Tachyon Potential, JHEP 9912:027, 1999 (arXiv:hep-th/9911116)

The resulting conjecture that D-brane charge is charge quantized in topological K-theory:

• Witten 98, Section 3

Discussion via superstring field theory:

• Theodore Erler, Analytic Solution for Tachyon Condensation in Berkovits’ Open Superstring Field Theory, JHEP 1311 (2013) 007 (arXiv:1308.4400)

which concludes (on p. 32) with:

It would also be interesting to see if these developments can shed light on the long-speculated relation between string field theory and the K-theoretic description of D-brane charge $[$75, 76, 77$]$. We leave these questions for future work.

Similarly in

• Theodore Erler, Four Lectures on Analytic Solutions in Open String Field Theory (arXiv:1912.00521, spire:1768105)

which lists (on p. 112) among open problems of string field theory:

Are there topological invariants of the open string star algebra representing D-brane charges?

For bosonic strings

The conjecture for bosonic strings originates with

• Sen 99, Section 4

An analytic check was found in

• Martin Schnabl, Analytic solution for tachyon condensation in open string field theory, Adv. Theor. Math. Phys.

  Volume 10, Number 4 (2006), 433-501. (arXiv:hep-th/0511286)

• Ian Ellwood, Martin Schnabl, Proof of vanishing cohomology at the tachyon vacuum, JHEP 0702:096,2007 (arXiv:hep-th/0606142)