nLab metric Lie algebra
Context
Lie theory
∞-Lie theory (higher geometry)
Background
Smooth structure
Higher groupoids
Lie theory
∞-Lie groupoids
∞-Lie algebroids
Formal Lie groupoids
Cohomology
Homotopy
Related topics
Examples
-Lie groupoids
-Lie groups
-Lie algebroids
-Lie algebras
Contents
Defintion
A metric Lie algebra or quadratic Lie algebra over some ground field is
-
Lie algebra
such that
-
is symmetric and invariant under the adjoint action, hence is an invariant polynomial on ;
-
is non-degenerate as a bilinear form, in that its tensor product-adjunct is a linear isomorphism.
(But may be indefinite.)
The following table shows the data in a metric Lie representation equivalently
-
in category theory-notation;
-
in index notation:
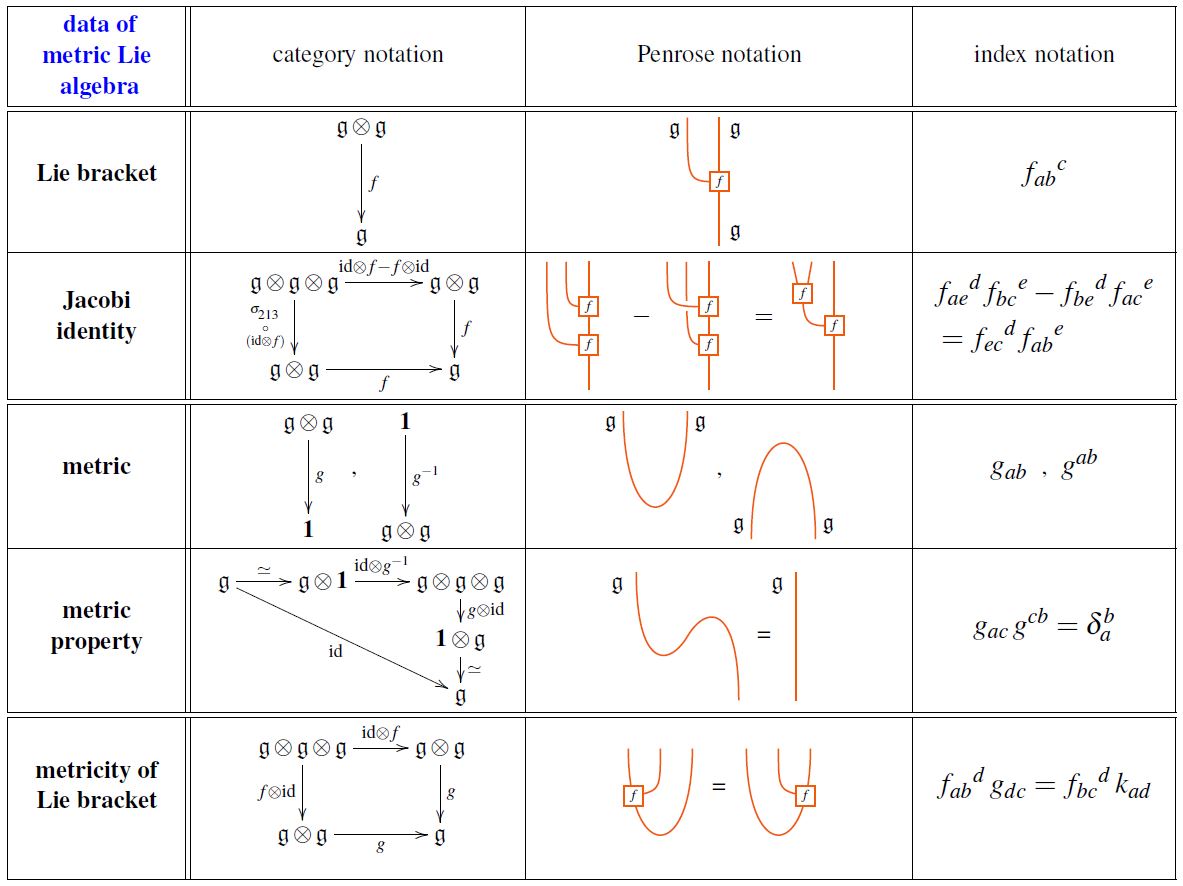
graphics from Sati-Schreiber 19c
Examples
- Every semisimple Lie algebra is a metric Lie algebra via its Killing form.
Applications
Lie algebra weight systems on chord diagrams
“Most” weight systems on chord diagrams come from metric Lie representations over metric Lie algebras: these are the Lie algebra weight systems.
Related concepts
References
General
On the Faulkner construction:
- John Faulkner, On the geometry of inner ideals, Journal of Algebra, Volume 26, Issue 1, July 1973, Pages 1-9 (doi:10.1016/0021-8693(73)90032-X)
See also
- Wikipedia, Quadratic Lie algebra
Relation to M2-brane 3-Lie algebras
The full generalized axioms on the M2-brane 3-algebra and first insights into their relation to Lie algebra representations of metric Lie algebras is due to
- Sergey Cherkis, Christian Saemann, Multiple M2-branes and Generalized 3-Lie algebras, Phys. Rev. D78:066019, 2008 (arXiv:0807.0808)
The full identification of M2-brane 3-algebras with dualizable Lie algebra representations over metric Lie algebras is due to
- Paul de Medeiros, José Figueroa-O'Farrill, Elena Méndez-Escobar, Patricia Ritter, On the Lie-algebraic origin of metric 3-algebras, Commun. Math. Phys. 290:871-902, 2009 (arXiv:0809.1086)
reviewed in
- José Figueroa-O'Farrill, slide 145 onwards in: Triple systems and Lie superalgebras in M2-branes, ADE and Lie superalgebras, talk at IPMU 2009 (pdf, pdf)
further explored in
- José Figueroa-O'Farrill, Simplicity in the Faulkner construction, Journal of Physics A: Mathematical and Theoretical, Volume 42, Number 44 (arXiv:0905.4900)
and putting to use the Faulkner construction that was previously introduced in (Faulkner 73)
See also:
-
Sam Palmer, Christian Saemann, section 2 of M-brane Models from Non-Abelian Gerbes, JHEP 1207:010, 2012 (arXiv:1203.5757)
-
Patricia Ritter, Christian Saemann, section 2.5 of Lie 2-algebra models, JHEP 04 (2014) 066 (arXiv:1308.4892)
-
Christian Saemann, appendix A of Lectures on Higher Structures in M-Theory (arXiv:1609.09815)
Last revised on December 2, 2023 at 09:30:58. See the history of this page for a list of all contributions to it.