nLab G₂/SU(3) is the 6-sphere

Statement

The coset space of the exceptional Lie group G₂ by its special unitary subgroup SU(3) is diffeomorphic to the 6-sphere:

$$G_2/SU(3) \;\simeq\; S^6 \,.$$

Related concepts

coset space-structures on n-spheres:

standard:
$S^{n-1} \simeq_{diff} SO(n)/SO(n-1)$	this Prop.
$S^{2n-1} \simeq_{diff} SU(n)/SU(n-1)$	this Prop.
$S^{4n-1} \simeq_{diff} Sp(n)/Sp(n-1)$	this Prop.
exceptional:
$S^7 \simeq_{diff} Spin(7)/G_2$	Spin(7)/G₂ is the 7-sphere
$S^7 \simeq_{diff} Spin(6)/SU(3)$	since Spin(6) $\simeq$ SU(4)
$S^7 \simeq_{diff} Spin(5)/SU(2)$	since Sp(2) is Spin(5) and Sp(1) is SU(2), see Spin(5)/SU(2) is the 7-sphere
$S^6 \simeq_{diff} G_2/SU(3)$	G₂/SU(3) is the 6-sphere
$S^15 \simeq_{diff} Spin(9)/Spin(7)$	Spin(9)/Spin(7) is the 15-sphere

see also Spin(8)-subgroups and reductions

homotopy fibers of homotopy pullbacks of classifying spaces:

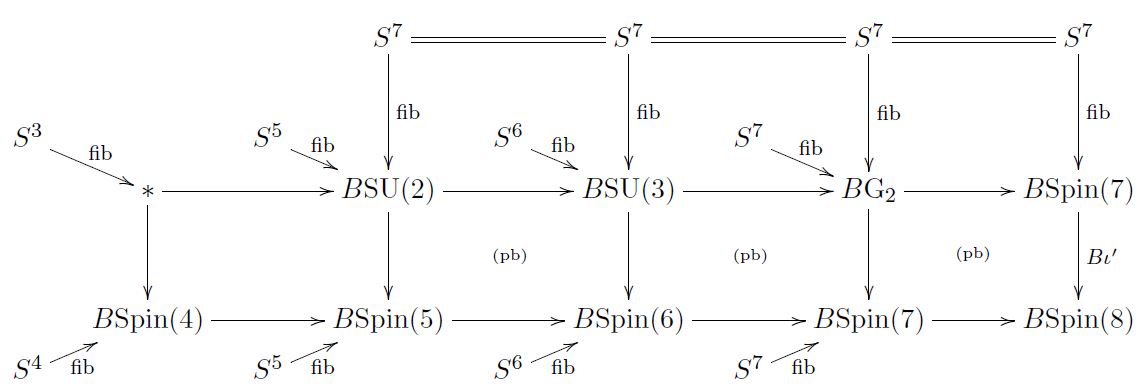
(from FSS 19, 3.4)

References

According to Gray-Green 70, p. 2 the statement is originally due to

• Deane Montgomery, Hans Samelson, Transformation Groups of Spheres, Annals of Mathematics Second Series, Vol. 44, No. 3 (Jul., 1943), pp. 454-470 (jstor:1968975)

According to ABF 17 the statement is originally due to to

• T. Fukami, S. Ishihara, Almost Hermitian structure on $S^6$, Tohoku Math J. 7 (1955), 151–156 (doi:10.2748/tmj/1178245052)

Review:

• Alfred Gray, Paul S. Green, p. 2 of Sphere transitive structures and the triality automorphism, Pacific J. Math. Volume 34, Number 1 (1970), 83-96 (euclid:1102976640)

• Robert Bryant, Section 1.3 of Manifolds with $G_2$-Holonomy, lecture notes, 1998 (web)

• Robert Bryant, Section 2.3 of Some remarks on $G_2$-structures, Proceedings of 12th Gokova Geometry-Topology Conference, 2005 (pdf)

• Simon Salamon, p. 6 of A tour of exceptional geometry, Milanj. math.72 (2002)1-0 (web)

• A. J. MacFarlane, The sphere $S^6$ viewed as a $G_2/SU(3)$-coset space, International Journal of Modern Physics A Vol. 17, No. 19, pp. 2595-2613 (2002) (doi:10.1142/S0217751X02010650)

• A. Gyenge, Section 4.2 of On the topology of the exceptional Lie group $G_2$, 2011 (pdf)

• Ilka Agricola, Aleksandra Borówka, Thomas Friedrich, $S^6$ and the geometry of nearly Kähler 6-manifolds (arXiv:1707.08591)