nLab function application to identifications
Context
Type theory
natural deduction metalanguage, practical foundations
type theory (dependent, intensional, observational type theory, homotopy type theory)
computational trinitarianism =
propositions as types +programs as proofs +relation type theory/category theory
Equality and Equivalence
-
equality (definitional, propositional, computational, judgemental, extensional, intensional, decidable)
-
identity type, equivalence of types, definitional isomorphism
-
isomorphism, weak equivalence, homotopy equivalence, weak homotopy equivalence, equivalence in an (∞,1)-category
-
Examples.
Contents
Idea
The type-theoretic version of the fact that in set theory functions preserve equality between elements in sets.
Definition
Basic definition
In dependent type theory, given types and and a function , there is induced a dependent function between the corresponding identity types
defined by Id-induction from
and called the application or action of to/on identities/identifications/equalities/paths [e.g. UFP (2013, p. 69), Rijke (2022, §5.3)].
By repeated Id-induction it readily follows, stagewise (e.g. UFP13, Lem 2.2.1), that respects the concatenation, and the inversion of identifications, up to coherent higher-order identifications, hence that it acts as an -functor on the -groupoid-structure on homotopy types :
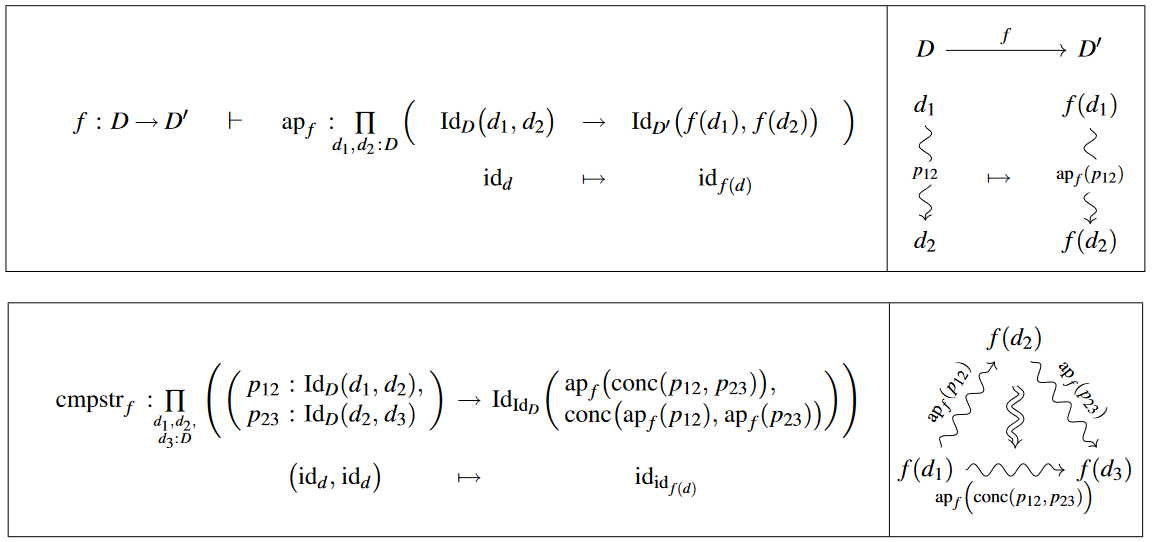
Relation to dependent function application to identifications
The function application to identifications is a special case of the dependent function application to identifications for which the type family is a constant type family, and thus the dependent identity type doesn’t depend on the path and is thus a normal identity type .
Inductive definition
If the function application to identifications is inductively defined, then it comes with rules saying that the following judgment can be formed
where is the loop space type of at .
For functions of arbitrary arity
Binary function applications to identifications
Given types , , and and a binary function , there is a dependent function
called the binary function application to identifications or binary action on identifications (Rijke (2022), §19.5), inductively defined by
where is the loop space type of at .
Binary function applications to identifications are used in proving product extensionality for product types, as well as defining multiplication on the integers from multiplication on the circle type when the integers are defined as the loop space type of the element , .
Finitary function application to identifications
Let be a natural number, let be a family of types indexed by the finite type with elements , and let be a type. Then given a function
there is a dependent function
For identifications of arbitrary arity
One can also consider function application to identifications of arity , , given a family of elements indexed by type . We have, for a function , a reflexive family
and thus by identification induction of the identity type of arity , we have a function
called the application of function to identifications of arity or the action of function on identifications of arity , such that for all ,
Related concepts
References
-
Univalent Foundations Project, §2.2 in: Homotopy Type Theory – Univalent Foundations of Mathematics (2013) [web, pdf]
-
Egbert Rijke, §5.3 in: Introduction to Homotopy Type Theory, Cambridge Studies in Advanced Mathematics, Cambridge University Press (arXiv:2212.11082)
Last revised on July 3, 2025 at 21:12:54. See the history of this page for a list of all contributions to it.