nLab Möbius strip
Context
Topology
topology (point-set topology, point-free topology)
see also differential topology, algebraic topology, functional analysis and topological homotopy theory
Basic concepts
-
fiber space, space attachment
Extra stuff, structure, properties
-
Kolmogorov space, Hausdorff space, regular space, normal space
-
sequentially compact, countably compact, locally compact, sigma-compact, paracompact, countably paracompact, strongly compact
Examples
Basic statements
-
closed subspaces of compact Hausdorff spaces are equivalently compact subspaces
-
open subspaces of compact Hausdorff spaces are locally compact
-
compact spaces equivalently have converging subnet of every net
-
continuous metric space valued function on compact metric space is uniformly continuous
-
paracompact Hausdorff spaces equivalently admit subordinate partitions of unity
-
injective proper maps to locally compact spaces are equivalently the closed embeddings
-
locally compact and second-countable spaces are sigma-compact
Theorems
Analysis Theorems
Contents
Idea
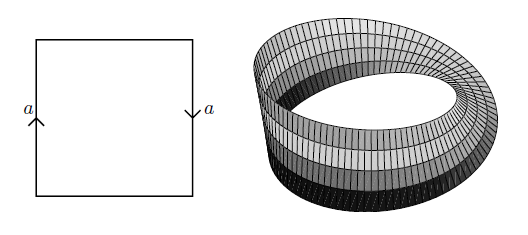
graphics grabbed from Lawson 03
The Möbius strip is the topological space obtained from the “open strip”, hence the square, by gluing two of its opposite sites but after applying half of a full rotation to one of them.
Realizations
As a quotient of the open strip
As a topological space, the Möbius strip is the quotient topological space obtained from the square by the equivalence relation which identifies two of the opposite sides, but with opposite orientation
As the tautological line bundle over
Regarded a vector bundle over the circle, the Möbius strip is the tautological line bundle over the 1-dimensional real projective space .
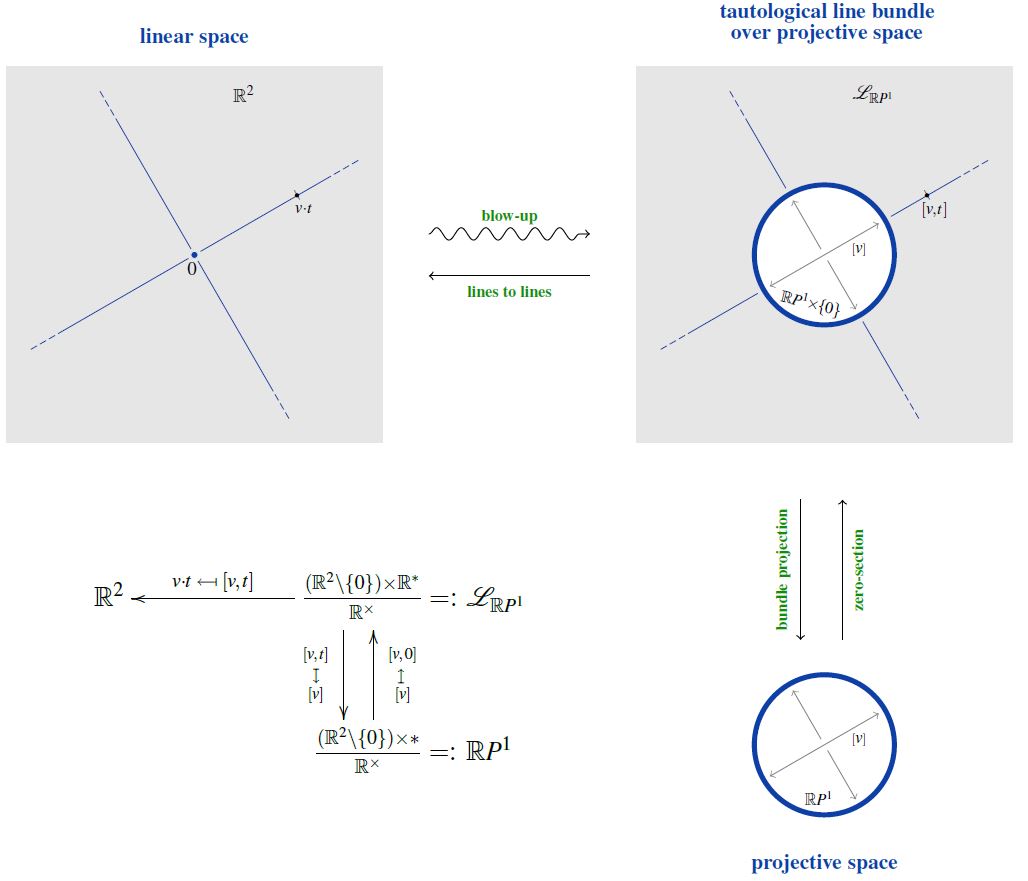
For more discussion of the topological vector bundle structure see this example and this prop.
Properties
Regarded as a manifold, the Möbius strip is among the simplest examples of a manifold that is not orientable.
Regarded as a real vector bundle over the circle, the Möbius strip is among the simplest examples of a non-trivial vector bundle.
Related concepts
References
Named after August Möbius.
- Terry Lawson, Topology: A Geometric Approach, Oxford University Press (2003) (pdf)
Last revised on November 22, 2020 at 19:54:02. See the history of this page for a list of all contributions to it.