nLab orbit category
Context
Homotopy theory
homotopy theory, (∞,1)-category theory, homotopy type theory
flavors: stable, equivariant, rational, p-adic, proper, geometric, cohesive, directed…
models: topological, simplicial, localic, …
see also algebraic topology
Introductions
Definitions
Paths and cylinders
Homotopy groups
Basic facts
Theorems
Representation theory
geometric representation theory
Ingredients
Definitions
representation, 2-representation, ∞-representation
Geometric representation theory
-
Grothendieck group, lambda-ring, symmetric function, formal group
-
principal bundle, torsor, vector bundle, Atiyah Lie algebroid
-
Eilenberg-Moore category, algebra over an operad, actegory, crossed module
Theorems
Cohomology
Special and general types
-
group cohomology, nonabelian group cohomology, Lie group cohomology
-
-
cohomology with constant coefficients / with a local system of coefficients
Special notions
Variants
-
differential cohomology
Extra structure
Operations
Theorems
Contents
- Idea
- Definition
- Equivariant Postnikov tower
- Equivariant set of connected components
- Equivariant fundamental groupoid
- Equivariant universal cover
- Examples
- Properties
- General properties
- Relation to -spaces and Elmendorf’s theorem
- Relation to global equivariant homotopy theory
- Relation to Mackey functors
- Relation to Bredon equivariant cohomology
- Base change of equivariance groups
- Related concepts
- References
Idea
The orbit category of a group is the category of “all kinds” of orbits of , namely of all suitable coset spaces regarded as G-spaces.
Definition
Definition
Given a topological group the orbit category (denoted also ) is the category whose
-
objects are the homogeneous spaces (coset spaces, -orbit types) , where is a closed subgroup of ,
-
morphismsare the -equivariant continuous functions.
(e.g. tom Dieck 1987, I (10.1))
Remark
Warning: This should not be confused with the situation where a group acts on a groupoid so that one obtains the orbit groupoid.
Remark
For suitable continuous actions of on a topological space , every orbit of the action is isomorphic to one of the coset spaces (the stabilizer group of any point in the orbit is conjugate to ). This is the sense in which def. gives “the category of all -orbits”.
Remark
Def. yields a small topologically enriched category (though of course if is a discrete group, the enrichment of is likewise discrete).
Of course, like any category, it has a skeleton, but as usually defined it is not itself skeletal, since there can exist distinct subgroups and such that .
Remark
(-sets are the free coproduct completion of -orbits)
Let be a discrete group. Since every G-set decomposes as a disjoint union of transitive actions, namely of orbits of elements of , the defining inclusion of the orbit category into exhibits the latter as its free coproduct completion (see also this Prop.).
Remark
(families of subgroups)
More generally, given a family of subgroups of which is closed under conjugation and taking subgroups one looks at the full subcategory whose objects are those for which . For such families, many of the considerations of results such as Elmendorf's theorem will still hold.
Equivariant Postnikov tower
Given a topological G-space, there is (following tom Dieck 1987, Sec. I.10) a sequence of variants (enhancements) of the -orbit category which mixes transitions between the fixed loci with n-truncations of their homotopy types.
Equivariant set of connected components
(…)
(…)
Equivariant fundamental groupoid
Definition
For a discrete group and a topological -space, its fundamental category (tom Dieck 1987 (10.7), Lück 1989, 8.15) or equivariant fundamental groupoid (Pronk & Scull 2021, Def. 3.1)
is the small category whose
-
objects are pairs consisting of a subgroup and a -equivariant function , hence, equivalently, a point in the fixed locus ;
-
morphisms are pairs consisting of a -equivariant function and a relative -homotopy class of a continuous path from to .
Example
For the point, its fundamental category (equivariant fundamental groupoid) according to Def. is the plain orbit category (Def. ).
Proposition
(equivariant fundamental groupoid under Grothendieck construction)
If we write
for the fundamental groupoid of in its incarnation as a presheaf of fixed loci over the orbit category, hence for the (2,1)-functor
which sends to the ordinary fundamental groupoid of the fixed locus , then the equivariant fundamental groupoid of (Def. ) is equivalently the Grothendieck construction
on (1).
This follows by direct unwinding of the definitions; see also Pronk & Scull 2021, below Def. 3.1.
Equivariant universal cover
Definition
(equivariant universal covering)
For a discrete group and a well-behaved topological -space, its equivariant universal cover (tom Dieck 1987 (10.13)) “is” the functor from the fundamental equivariant groupoid (2) of to TopSp
which sends to the universal covering of the connected component of given by relative-homotopy-equivalences classes of paths based at .
Write
for the -category of -presheaves on the -orbit category, hence for the proper -equivariant homotopy theory.
For a topological -space, its equivariant homotopy type is
Proposition
For a topological -space, its equivariant universal covering according to Def. is, under Prop. and the (infinity,1)-Grothendieck construction equivalent to the 1-truncation unit
in that
bottom right corner not quite right, will fix…
Examples
Example
(orbit category of Z/2Z)
For equivariance group the cyclic group of order 2:
the orbit category looks like this:
i.e.:
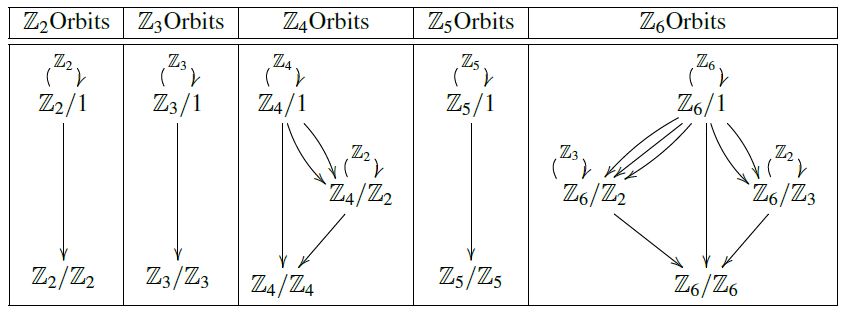
Properties
General properties
- Orbit categories as well as fundamental categories of -spaces are EI-categories.
Relation to -spaces and Elmendorf’s theorem
Elmendorf's theorem (see there for details) states that the (∞,1)-category of (∞,1)-presheaves on the orbit category are equivalent to the localization of topological spaces with -action at the weak homotopy equivalences on fixed point spaces.
Relation to global equivariant homotopy theory
The -orbit category is the slice (∞,1)-category of the global orbit category (the version with faithful functors as morphisms) over the delooping :
This means that in the general context of global equivariant homotopy theory, the orbit category appears as follows.
For more on this see also at cohesion of global- over G-equivariant homotopy theory the section the adjunction of sites.
Rezk-global equivariant homotopy theory:
Relation to Mackey functors
Orbit categories are used often in the treatment of Mackey functors from the theory of locally compact groups and in the definition of Bredon cohomology.
Relation to Bredon equivariant cohomology
It appears in equivariant stable homotopy theory, where the -fixed homotopy groups of a space form a presheaf on the homotopy category of the orbit category (e.g. page 8, 9 here).
Base change of equivariance groups
Example
(left base change along covering of equivariance group)
Let be a surjective homomorphism of discrete groups. For a subgroup, write for its pullback
Then there is a reflective subcategory-inclusion of orbit categories
where
-
.
A transparent way to see this is to identify (as above) -orbits with the 0-truncated objects in the -slice of :
Under this identification, the adjunction is the canonical left base change on slices composed with the 0-truncation reflection (here):
That the right adjoint is fully faithful is most readily seen by direct inspection: A map between -sets on both of which acts only through is evidently -equivariant if and only if it is -equivariant.
Related concepts
References
The notion of the orbit category (for use in equivariant cohomology/Bredon cohomology) is due to
- Glen Bredon, Section I.3. of Equivariant cohomology theories, Springer Lecture Notes in Mathematics Vol. 34. 1967 (doi:10.1007/BFb0082690)
announced in
- Glen Bredon, Equivariant cohomology theories, Bull. Amer. Math. Soc. Volume 73, Number 2 (1967), 266-268. (educlid:1183528794)
and there considered specifically for finite groups.
Discussion for any topological group (and further generalization) is considered (together with the model category theoretic proof of Elmendorf's theorem) in:
- William Dwyer, Daniel Kan, Sec. 2.1 and Thm. 3.1 of: Singular functors and realization functors, Indagationes Mathematicae (Proceedings) Volume 87, Issue 2, 1984, Pages 147-153 (doi:10.1016/1385-7258(84)90016-7)
Textbook accounts:
-
Tammo tom Dieck, Section I.10 of: Transformation Groups, de Gruyter 1987 (doi:10.1515/9783110858372)
-
Wolfgang Lück, (8.16) in Transformation Groups and Algebraic K-Theory, Lecture Notes in Mathematics 1408 (Springer 1989) (doi:10.1007/BFb0083681)
-
Peter May, Section I.4 of: Equivariant homotopy and cohomology theory CBMS Regional Conference Series in Mathematics, vol. 91, Published for the Conference Board of the Mathematical Sciences, Washington, DC, 1996 (ISBN:978-0-8218-0319-6, pdf, pdf)
Lecture notes:
- Tammo tom Dieck, Section 1.3 of: Representation theory, 2009 (pdf)
For more in relation to global equivariant homotopy theory see
The notion of fundamental category of a -space is due to
with further discussion in
- Dorette Pronk, Laura Scull, The Equivariant Fundamental Groupoid as an Orbifold Invariant, Homology, Homotopy and Applications 23 1 (2021) (arXiv:1908.01201, doi:10.4310/HHA.2021.v23.n1.a3)
Last revised on November 16, 2023 at 14:25:40. See the history of this page for a list of all contributions to it.