nLab pre-metric electromagnetism

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Discuss this page |
Redirected from "premetric electromagnetism".
Contents

Context

Physics

physics, mathematical physics, philosophy of physics

Surveys, textbooks and lecture notes

theory (physics), model (physics)

experiment, measurement, computable physics

Contents

Idea

Inspection of the equations of motion for classical electromagnetism (Maxwell's equations) readily reveals that — either in vacuum, in a dielectric medium or in a background field of gravity — they may equivalently be (re)written (in fact this is close to their original historical form) as

  1. a system of purely “topological”, “cohomological” or “exterior” partial differential equations

    on twice the number of physical fields,

  2. a linear “constitutive equation” imposing a “duality” relation on the fields

    reducing them to the physical degrees of freedom,

where it is only the second but not the first part that involves the “background” structure, namely the dielectric tensor characterizing the ambient dielectric medium and/or the pseudo-Riemannian metric characterizing the field of gravity.

This fact itself is fairly evident (certainly in modern formulations of Maxwell’s equations, see below), but some authors have highlighted it as possibly being of deeper relevance, starting with Kottler (1922a), (1922b), Cartan (1924) §80, Dantzig (1934) and more recently Hehl & Obukhov (2003), Delphenich (2005a), (2005b) (see the comprehensive account by Delphenich (202x)), the broad idea being that the second step above should be regarded as, indeed, secondary and possibly subject to conceptual re-evaluation (e.g. Delphenich (2015)).

We point out below (see also Freed (2002)) that, while the explicit perspective of “pre-metric electromagnetism” is maybe not widely appreciated under this name, in fact exactly the same idea – just with the electromagnetic field replaced by the (hypothetical) “RR-field” and then often called the “democratic” instead of “premetric” formulation – is secretly at the heart of the the widely recognized conjecture of K-theory classification of D-brane charge as well as of related conjectures, such as Hypothesis H.

Premetric electromagnetism

Electromagnetism in gravitational backgrounds

The equations of motion of classical electromagnetism (Maxwell's equations) on any spacetime manifold (X,g)(X,g) read, in modern differential form-formulation:

(1)dF = 0, d⋆ gF = j \begin{array}{rcl} \mathrm{d}\, F &=& 0 \mathrlap{\,,} \\ \mathrm{d} \, \star_g F &=& j \end{array}

where

and last not least

Often this is considered for X≃ℝ 3,1X \simeq \mathbb{R}^{3,1} being Minkowski spacetime, hence for g=ηg = \eta the Minkowski metric, in which case this describes pure “Maxwell theory”, but the exact same formulas apply in the generality that (X,g)(X,g) is any Lorentzian manifold, in which case they form the sector of the equations of motion of Einstein-Maxwell theory which involve the electromagnetic field and its coupling to background gravity. (The remaining sector are the Einstein equations for the metric field gg sourced by the stress-energy tensor of the Maxwell field.)

In order to bring out more manifestly that gravity (the pseudo-Riemannian metric) enters only through the Hodge star operator, we may evidently re-write the above pair of equations (1) equivalently as follows:

(2)dF = 0, dG = jandG=⋆ gF. \begin{array}{rcl} \mathrm{d}\, F &=& 0 \mathrlap{\,,} \\ \mathrm{d} \, G &=& j \end{array} \;\;\;\; \text{and} \;\;\;\; G \,=\, \star_g F \,.

Electromagnetism in dielectric media

In fact, this formulation (2) is closer to the (original) formulation of Maxwell’s equations used in the case that spacetime XX is thought of as possibly filled with a dielectric medium where, a priori, one considers two groups of fields:

  1. the electric field E→\vec E and magnetic flux density B→\vec B

    which jointly constitute the Faraday tensor, given (on a local coordinate chart (t,x 1,x 2,x 3)(t,x^1, x^2, x^3)) by (see there):

F=E idx i∧dt+ϵ ijkB idx jdx k F \,=\, E_i \mathrm{d}x^i \wedge \mathrm{d} t \,+\, \epsilon_{i j k} B^i \mathrm{d} x^j \mathrm{d} x^k

as well as

  1. the actual magnetic field H→\vec H and the dielectric displacement (or similar) D→\vec D, with

    G=H idx i∧dt−ϵ ijkD idx jdx k, G \,=\, H_i \mathrm{d}x^i \wedge \mathrm{d} t \,-\, \epsilon_{i j k} D^i \mathrm{d} x^j \mathrm{d} x^k \,,

and then imposes constitutive equations relating (E→,B→)(\vec E, \vec B) to (H→,D→)(\vec H, \vec D) and thereby expressing the dielectric-property of any electromagneric medium that one imagines filling the spacetime XX.

In general, constitutive equations can be nonlinear (and even multi-valued, reflecting hysteresis effects), but for sufficiently small field strengths they are of the form

G=⋆ ϵF G \,=\, \star_\epsilon F

for a linear map

⋆ ϵ:Ω 2(X)⟶Ω 2(X) \star_\epsilon \,\colon\, \Omega^2(X) \longrightarrow \Omega^2(X)

much like the Hodge star operator (whence here we use similar notation for both, which is non-standard).

Duality-symmetric higher gauge theory

While not traditionally discussed under this terminology in the literature, we may recognize (SS23) higher versions of “premetric” electromagnetism occuring in the supergravity- and string theory-literature as duality-symmetric or “democratic” formulations.

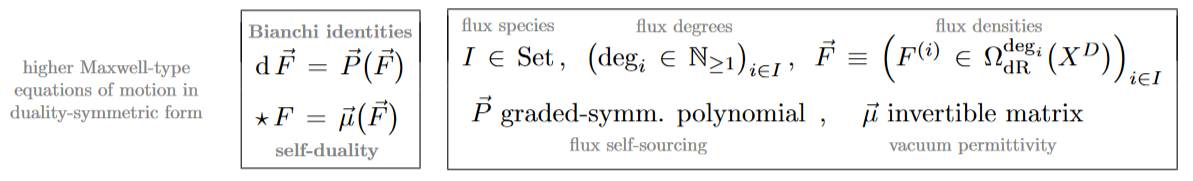
For more on the general picture see at Gauss law – In higher gauge theory.

RR-fields in gravitational backgrounds

The theory of type II D=10 supergravity famously contains higher gauge fields called the Ramond-Ramond fields or RR-fields for short, which may be understood as a certain higher-degree generalization of the electromagnetic field:

Where the electromagnetic field strength is a single differential 2-form F 2∈Ω 2(X)F_2 \,\in\, \Omega^2(X) (the Faraday tensor), the RR-fields have their field strengths encoded in a tuple of differential forms in every second degree up to half the spacetime dimension:

{F 2p+σ∈Ω 2p+σ(X)|0≤2p+κ≤5}, \Big\{ F_{2p + \sigma} \,\in\, \Omega^{2p+\sigma}(X) \;{\Big\vert}\; 0 \leq 2p+\kappa \leq 5 \Big\} \,,

where σ=0\sigma = 0 for type IIA supergravity and σ=1\sigma = 1 for type IIB supergravity.

Moreover, the equations of motion of these RR-fields are of the general form of Maxwell's equations (1), in fact when the Kalb-Ramond field and the spinor fields vanish, then the equations of motion are as before, up to form degree

(3)dF 2p+σ = 0 d⋆ gF 2p+σ = 0. \begin{array}{rcl} \mathrm{d} F_{2p + \sigma} &=& 0 \\ \mathrm{d} \star_g F_{2p + \sigma} &=& 0 \mathrlap{\,.} \end{array}

(In more generality the right-hand sides are non-vanishing combinations with the Kalb-Ramond field – already for the first equation! – and the spinor fields.)

But one may famously understand type II supergravity as the low-energy limit of type II string theory, in which case there are subtle flux quantization laws of the total flux of these RR-fields (in variation of the Dirac charge quantization-law imposed on the Maxwell field when regarding electromagnetism as the background field for quantum electrons).

A widely-considered conjecture (here) says that this flux quantization is realized by

  1. regarding the would-be Hodge duals ⋆ gF 2p+σ\star_g F_{2p + \sigma} of the RR-fields as independent fields F 10−2p−σF_{10-2p-\sigma}, so that the “pre-metric RR-field” now has about twice as many components:

    {F 2p+σ∈Ω 2p+σ(X)|0≤2p+κ≤5}, \Big\{ F_{2p + \sigma} \,\in\, \Omega^{2p+\sigma}(X) \;{\Big\vert}\; 0 \leq 2p+\kappa \leq 5 \Big\} \,,

    and then regarding/constraining such a tuple as the image under the Chern character of a class in (differentia) complex topological K-theory in addition to imposing the pre-metric equations of motion.

  2. afterwards imposing the Hodge-duality constraint, now thought of as a “self-duality” on K-theory and remaining subject to discussion in the literature: see at self-dual higher gauge field the section Examples – RR-Fields.

(4)dF 2•+σ=0 F D−2•−σ=⋆ gF 2•+σ \array{ \mathrm{d} \, F_{2\bullet + \sigma} \;=\; 0 \\ F_{D-2\bullet-\sigma} \;=\; \star_g F_{2\bullet+\sigma} }

(Again, this is stated so far for vanishing Kalb-Ramond field, for ease of exposition here. More generally the KR-field does not vanish and the above discussion applies for twisted K-theory.)

Clearly, this just the idea of “pre-metric electromagnetism” but now enacted in a variant situation. The string theory-literature refers to this mostly as the “democratic” formulation of the RR-fields, see Dall’Agata, Lechner & Tonin (1998) or Mkrtchyan & Valach (2023).

CC-Field in gravitational background

Somewhat similarly, the equations of motion of the supergravity C-field in D=11 supergravity are of the form

dG 4 = 0 d⋆ gG 4 = 12G 4∧G 4 \begin{array}{rcl} \mathrm{d} G_4 &=& 0 \\ \mathrm{d} \star_g G_4 &=& \tfrac{1}{2} G_4 \wedge G_4 \end{array}

where now G 4∈Ω 4(X)G_4 \,\in\, \Omega^4(X) is a differential 4-form. If we regard these equations in “pre-metric” form as in the above discussion of the RR-fields, by introducing an a priori independent 7-form G 7∈Ω 7(X)G_7 \,\in\, \Omega^7(X), then they read

(5)dG 4 = 0 dG 7 = −12G 4∧G 4andG 7=⋆ gG 4. \begin{array}{rcl} \mathrm{d} G_4 &=& 0 \\ \mathrm{d} G_7 &=& -\tfrac{1}{2} G_4 \wedge G_4 \end{array} \;\;\;\;\;\; \text{and} \;\;\;\;\;\; G_7 \,=\, \star_g G_4 \,.

As for the RR-field and its conjectured charge quantization in K-theory, in this “pre-metric” formulation it makes sense to ask which generalized cohomology theory yields the differential equations on the left, as those characterizing the image under its generalized Chern-Dold-like charatcer.

There is no Whitehead-generalized cohomology theory with this property, due to the non-linear quadratic function G 4↦12G 4∧G 4G_4 \mapsto \tfrac{1}{2} G_4 \wedge G_4 appearing in (5), but there is a non-abelian cohomology theory with the correct nonabelian character map: namely 4-Cohomotopy theory. This observation (due to Sati (2013,2018), §2.5) suggests (this is “Hypothesis H”) that the charge quantization of the supergravity C-field in M-theory may be in some form of Cohomotopy theory.

References

Premetric electromagnetic fields

The idea (if not the terminology) of pre-metric electromagnetism is attributed to:

  • Friedrich Kottler, Newtonsches Gesetz und Metrik, Sitzungsber. Akad. Wiss. Wien, Math.-Naturw. Klasse, Abt. IIa, 131 (1922) 1-14 [Engl. transl. by Delphenich: pdf, pdf]

  • Friedrich Kottler, Maxwell’sche Gleichungen und Metrik, Sitz. Akad. Wien IIa 131 (1922) 119-146 [Engl. transl. by Delphenich: pdf, pdf]

  • Élie Cartan, §80 in: Sur les variétés à connexion affine, et la théorie de la relativité généralisée (première partie) (Suite), Annales scientifiques de l’É.N.S. 3e série, tome 41 (1924) 1-25 [numdam:ASENS_1924_3_41__1_0]

  • David van Dantzig, The fundamental equations of electromagnetism, independent of metrical geometry, Mathematical Proceedings of the Cambridge Philosophical Society 30 4 (1934) 421-427 [doi:10.1017/S0305004100012664]

Early review:

  • Edmund T. Whittaker: pp. 192-196 of: A History of the Theories of Aether and Electricity – Vol. 2: The Modern Theories 1900-1926 (1953), reprinted by Humanities Press (1973) [pdf, Wikipedia entry]

    “[…] This chapter has been concerned, for the most part, with General Relativity, which is essentially a geometrisation of physics. It may be closed with some account of a movement in the opposite direction, seeking to abolish the priviledged position of geometry in physics, and indeed inquiring how far it may be possible to construct a physics independent of geometry. Since the notion of metric is a complicated one, which requires measurements with clocks and scales, generally with rigid bodies, which themselves are systems of great complexity, it seems undesirable to take metric as fundamental, particularly for phenomena which are simpler and actually independent of it.”

    “The movement was initiated by Friedrich Kottler of Vienna, who in 1922 published two papers [Kottler (1922a), (1922b)]”

Further amplification of the constitutive map that relates the pre-metrically independent fields:

The terminology “pre-metric electromagnetism” seems to be due to David H. Delphenich, who gives much further discussion:

Monographs:

The general principle of the pre-metric formulation, understood as a form of self-dual higher gauge theory, also appears in:

leading over to generalization of the idea such as to RR-fields.

Further discussion:

Duality-symmetric RR-fields

References which make the “democratic” (“pre-geometric”) formulation (4) of the RR-fields in type II supergravity manifest:

Discussion of (Lagrangian densities for) D=10 type II supergravity with “duality-symmetric”/“democratic”/“pregeometric” for of the RR-fields:

Enhancement of the self-duality constraint on pregeometric RR-fields from (twisted) de Rham cohomology to (twisted) topological K-theory (under the hypothesized K-theory classification of D-brane charge) in terms of a quadratic form on differential K-theory:

An indication of a more refined discussion in twisted differential KR-theory:

See at orientifold for more on this.

Expressing the self-duality of pregeometric RR-fields in terms of 11d Chern-Simons theory:

Some review:

  • Richard Szabo, section 3.6 and 4.6 of: Quantization of Higher Abelian Gauge Theory in Generalized Differential Cohomology, ESI 2385 (2012) [[arXiv:1209.2530, pdf]]

Discussion in the context of flux quantization (here: D-brane charge quantization in K-theory):

Duality-symmetric C-field

Formulation of the equations of motion of D=11 supergravity in superspace on fields including a flux density G 7G_7 a priori independent of the flux density G 4G_4 of the supergravity C-field:

Discussion of Lagrangian densities for D=11 supergravity with an a priori independent dual C-field field and introduction of the “duality-symmetric” terminology:

Discussion in the context of shifted C-field flux quantization:

General

Last revised on February 2, 2024 at 07:53:15. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (20 revisions) Cite Print Source