nLab homotopy

This page is about homotopy as a transformation. For homotopy sets in homotopy categories, see homotopy (as an operation).

---

Idea

In many categories $C$ in which one does homotopy theory, there is a notion of homotopy between morphisms, which is closely related to the 2-morphisms in higher category theory: a homotopy between two morphisms is a way in which they are equivalent.

If we regard such a category as a presentation of an $(\infty,1)$-category, then homotopies $f\sim g$ present the 2-cells $f\Rightarrow g$ in the resulting $(\infty,1)$-category.

Definition

In topological spaces

Definition

For $f,g\colon X \longrightarrow Y$ two continuous functions between topological spaces $X,Y$, then a left homotopy

$$\eta \colon f \,\Rightarrow_L\, g$$

is a continuous function

$$\eta \;\colon\; X \times I \longrightarrow Y$$

out of the standard cylinder object over $X$: the product space of $X$ with the Euclidean closed interval $I \coloneqq [0,1]$, such that this fits into a commuting diagram of the form

$$\array{ X \\ {}^{\mathllap{(id,\delta_0)}}\downarrow & \searrow^{\mathrlap{f}} \\ X \times I &\stackrel{\eta}{\longrightarrow}& Y \\ {}^{\mathllap{(id,\delta_1)}}\uparrow & \nearrow_{\mathrlap{g}} \\ X } \,.$$

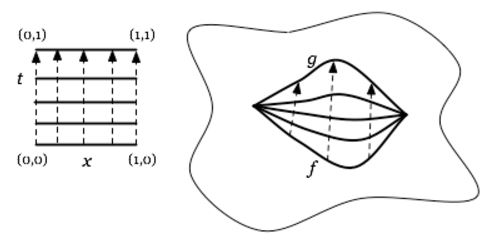
(graphics grabbed from J. Tauber here)

Example

Let $X$ be a topological space and let $x,y \in X$ be two of its points, regarded as functions $x,y \colon \ast \longrightarrow X$ from the point to $X$. Then a left homotopy, def. , between these two functions is a commuting diagram of the form

$$\array{ \ast \\ {}^{\mathllap{\delta_0}}\downarrow & \searrow^{\mathrlap{x}} \\ I &\stackrel{\eta}{\longrightarrow}& X \\ {}^{\mathllap{\delta_1}}\uparrow & \nearrow_{\mathrlap{y}} \\ \ast } \,.$$

This is simply a continuous path in $X$ whose endpoints are $x$ and $y$.

In enriched categories

If $C$ is enriched over Top, then a homotopy in $C$ between maps $f,g:X\,\rightrightarrows \,Y$ is a map $H:[0,1] \to C(X,Y)$ in $Top$ such that $H(0)=f$ and $H(1)=g$. In $Top$ itself this is the classical notion.

If $C$ has copowers, then an equivalent definition is a map $[0,1]\odot X\to Y$, while if it has powers, an equivalent definition is a map $X\to \pitchfork([0,1],Y)$.

There is a similar definition in a simplicially enriched category, replacing $[0,1]$ with the 1-simplex $\Delta^1$, with the caveat that in this case not all simplicial homotopies need be composable even if they match correctly. (This depends on whether or not all (2,1)-horns in the simplicial set, $C(X,Y)$, have fillers.) Likewise in a dg-category we can use the “chain complex interval” to get a notion of chain homotopy.

In model categories

If $\mathcal{C}$ is a model category, it has an intrinsic notion of homotopy determined by its factorizations. For more on the following see at homotopy in a model category.

Definition

Let $\mathcal{C}$ be a model category and $X \in \mathcal{C}$ an object.

• A path object $Path(X)$ for $X$ is a factorization of the diagonal $\nabla_X \colon X \to X \times X$ as

$$\nabla_X \;\colon\; X \underoverset{\in W}{i}{\longrightarrow} Path(X) \overset{(p_0,p_1)}{\longrightarrow} X \times X \,.$$

where $X\to Path(X)$ is a weak equivalence. This is called a good path object if in addition $Path(X) \to X \times X$ is a fibration.

• A cylinder object $Cyl(X)$ for $X$ is a factorization of the codiagonal (or “fold map”) $\Delta_X: X \sqcup X \to X$ as

$$\Delta_X \;\colon\; X \sqcup X \overset{(i_0,i_1)}{\longrightarrow} Cyl(X) \underoverset{p}{\in W}{\longrightarrow} X \,.$$

where $Cyl(X) \to X$ is a weak equivalence. This is called a good cylinder object if in addition $X \sqcup X \to Cyl(X)$ is a cofibration.

Remark

By the factorization axioms every object in a model category has both a good path object and as well as a good cylinder object according to def. . But in some situations one is genuinely interested in using non-good such objects.

For instance in the classical model structure on topological spaces, the obvious object $X\times [0,1]$ is a cylinder object, but not a good cylinder unless $X$ itself is cofibrant (a cell complex in this case).

More generally, the path object $Path(X)$ of def. is analogous to the powering $\pitchfork(I,X)$ with an interval object and the cylinder object $Cyl(X)$ is analogous to the tensoring $I\odot X$ with an interval object. In fact, if $\mathcal{C}$ is a $V$-enriched model category and $X$ is fibrant/cofibrant, then these powers and copowers are in fact examples of (good) path and cylinder objects if the interval object is sufficiently good.

Definition

Let $f,g \colon X \longrightarrow Y$ be two parallel morphisms in a model category.

• A left homotopy $\eta \colon f \Rightarrow_L g$ is a morphism $\eta \colon Cyl(X) \longrightarrow Y$ from a cylinder object of $X$, def. , such that it makes this diagram commute:

$$\array{ X &\longrightarrow& Cyl(X) &\longleftarrow& X \\ & {}_{\mathllap{f}}\searrow &\downarrow^{\mathrlap{\eta}}& \swarrow_{\mathrlap{g}} \\ && Y } \,.$$

• A right homotopy $\eta \colon f \Rightarrow_R g$ is a morphism $\eta \colon X \to Path(Y)$ to some path object of $X$, def. , such that this diagram commutes:

$$\array{ && X \\ & {}^{\mathllap{f}}\swarrow & \downarrow^{\mathrlap{\eta}} & \searrow^{\mathrlap{g}} \\ Y &\longleftarrow& Path(Y) &\longrightarrow& Y } \,.$$

Remark

By remark it follows that in a $Top$-enriched model category, any enriched homotopy between maps $X\to Y$ is a left homotopy if $X$ is cofibrant and a right homotopy if $Y$ is fibrant. Similar remarks hold for other enrichments.

For more see at homotopy in a model category.

In (co-)fibration categories

Clearly the concept of left homotopy in def. only needs part of the model category axioms and thus makes sense more generally in suitable cofibration categories. Dually, the concept of path objects in def. makes sense more generally in suitable fibration categories such as categories of fibrant objects in the sense of Brown.

Likewise if there is a cylinder functor, one gets functorially defined cylinder objects, etc.

In dependent type theory

In dependent type theory, let $A$ be a type and let $P$ be a type family indexed by $A$, and let $f,g:\prod_{x:A} P(x)$ be two elements of a dependent product type of a type family $P$. The type of homotopies between $f$ and $g$ is the type

$$f \sim g \equiv \prod_{x:A} (f(x) =_{B(x)} g(x))$$

A homotopy between $f$ and $g$ is simply an element $H:f \sim g$.

Note that a homotopy is not the same as an identification $f = g$. However this can be made so if one assumes function extensionality.

Related concepts

	homotopy	cohomology	homology
	$[S^n,-]$	$[-,A]$	$(-) \otimes A$
category theory	covariant hom	contravariant hom	tensor product
homological algebra	Ext	Ext	Tor
enriched category theory	end	end	coend
homotopy theory	derived hom space $\mathbb{R}Hom(S^n,-)$	cocycles $\mathbb{R}Hom(-,A)$	derived tensor product $(-) \otimes^{\mathbb{L}} A$

• homotopy theory

• left homotopy, right homotopy

• homotopy relative boundary

• smooth homotopy

• isotopy, smooth isotopy

• higher homotopy

• homotopy class

• transfor

  • natural transformation

  • pseudonatural transformation

  • lax natural transformation

• function extensionality

• whiskering

• homotopy analysis method

References

See the references at homotopy theory and at model category.

Discussion in computational topology:

• Marek Filakovský, Lukáš Vokřínek, Are two given maps homotopic? An algorithmic viewpoint, Found Comput Math (2019) (arXiv:1312.2337, doi:10.1007/s10208-019-09419-x)

For homotopies in Martin-Löf dependent type theory:

• Univalent Foundations Project, Homotopy Type Theory – Univalent Foundations of Mathematics (2013)