nLab glueball

Redirected from "glue-balls".

Contents

Context

Fields and quanta

fields and particles in particle physics

and in the standard model of particle physics:

force field gauge bosons

photon - electromagnetic field (abelian Yang-Mills field)
W, Z, B-boson - electroweak field (Yang-Mills field)
gluon - strong nuclear force (Yang-Mills field)
graviton - field of gravity
infraparticle

scalar bosons

Higgs boson, inflaton (scalar field)

matter field fermions (spinors, Dirac fields)

flavors of fundamental fermions in the standard model of particle physics:
generation of fermions	1st generation	2nd generation	3d generation
quarks ( $q$ )
up-type	up quark ( $u$ )	charm quark ( $c$ )	top quark ( $t$ )
down-type	down quark ( $d$ )	strange quark ( $s$ )	bottom quark ( $b$ )
leptons
charged	electron	muon	tauon
neutral	electron neutrino	muon neutrino	tau neutrino

bound states:
mesons	light mesons: pion ( $u d$ ) ρ-meson ( $u d$ ) ω-meson ( $u d$ ) f1-meson a1-meson	strange-mesons: ϕ-meson ( $s \bar s$ ), kaon, K-meson ( $u s$ , $d s$ ) eta-meson ( $u u + d d + s s$ ) charmed heavy mesons*: D-meson ( $u c$ , $d c$ , $s c$ ) J/ψ-meson ( $c \bar c$ )	bottom heavy mesons: B-meson ( $q b$ ) ϒ-meson ( $b \bar b$ )
baryons	nucleons: proton $(u u d)$ neutron $(u d d)$

(also: antiparticles)

effective particles

Goldstone bosons

hadrons (bound states of the above quarks)

meson
- scalar meson
  - σ-meson
  - pion
  - kaon
  - D-meson
  - B-meson
- vector meson
  - ω-meson
  - ρ-meson
  - f1-meson
  - a1-meson
  - b1-meson
  - h1-meson
  - K*-meson
- tensor meson
- quarkonium
  - charmonium
  - ϒ-meson
exotic meson
- XYZ meson
- tetraquark
baryon
- nucleon
  - proton
  - neutron
  - chemical element
    - carbon
    - nitrogen
- Lambda baryon
- pentaquark

solitons

in grand unified theory

minimally extended supersymmetric standard model

superpartners

bosinos:

gravitino - field of supergravity (Rarita-Schwinger field)
gaugino - super Yang-Mills field
gluino

sfermions:

squark

dark matter candidates

WIMP
axion

Exotica

auxiliary fields

Idea
Properties

In holographic QCD

References

General
In holographic QCD

Idea

In quantum chromodynamics, in fact already in pure SU(3) Yang-Mills theory, a glueball is a bound state of (just) gluons (a kind of confinement).

Glueballs are hard to detect in experiment but their existence is confirmed by lattice QCD, also by the AdS-QCD correspondence (see there). According to Greensite 11, section 8.5:

The caloron idea is probably the most promising current version of monopole confinement in pure non-abelian gauge theories, but it is basically (in certain gauges) a superposition of monopoles with spherically symmetric abelian fields, and this leads to the same questions raised in connection with monopole Coulomb gases.

Properties

In holographic QCD

In holographic QCD, glueballs are dual to massless scalar fields in the $\sim$ AdS-bulk spacetime (for instance, but not necessarily, the dilaton).

The following graphics shows predictions of glueball spectra from a simple AdS/QCD-model compared to lattice QCD-computations

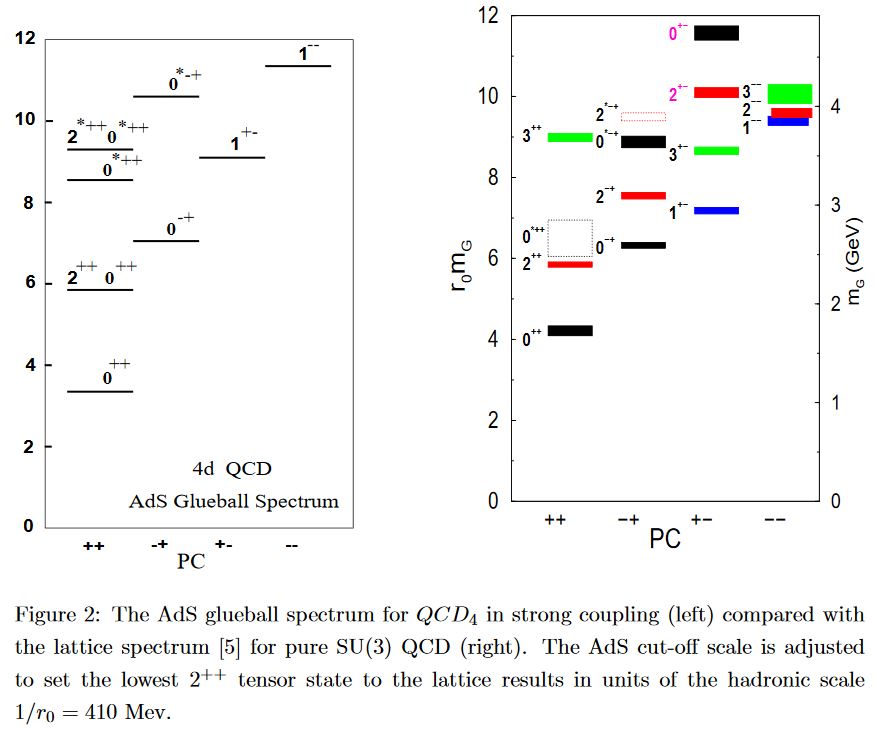
Apparently the spin structure of type IIA supergravity does resemble the low mass glueball spin splitting. (Brower-Mathur-Tan 00, p. 22)

References

General

The first article discussing glueballs is apparently

Harald Fritzsch, Peter Minkowski, Ψ-resonances, gluons and the Zweig rule, Nuov. Cim. A, 1975,30: 393 (doi:10.1007/BF02730295)

Review:

Vincent Mathieu, The Physics of Glueballs, Zakopane, February 2009 (pdf, pdf)
Jeff Greensite, An Introduction to the Confinement Problem, Lecture Notes in Physics, Volume 821, 2011 (doi:10.1007/978-3-642-14382-3)

In holographic QCD

Discussion of glueballs in holographic QCD (AdS/QCD):

Richard C. Brower, Samir Mathur, Chung-I Tan, Glueball Spectrum for QCD from AdS Supergravity Duality, Nucl. Phys. B587:249-276, 2000 (arXiv:hep-th/0003115)
Alex S. Miranda, C. A. Ballon Bayona, Henrique Boschi-Filho, Nelson R. F. Braga, Glueballs at finite temperature from AdS/QCD, Nucl. Phys. Proc. Suppl. 199 (2010) 107-112 [arXiv:0910.4319, doi:10.1016/j.nuclphysbps.2010.02.013]
Xue-Feng Li, Ailin Zhang, Scalar glueball in a soft-wall model of AdS/QCD, Chinese Physics C, Volume 38, Number 1 (arXiv:1309.7154)
Cornélio Rodrigues Filho, Glueballs in the Klebanov-Strassler Theory: Pseudoscalars vs Scalars (arXiv:2011.12689)

Last revised on July 1, 2024 at 16:41:12. See the history of this page for a list of all contributions to it.