nLab orientifold plane

Idea

In type II string theory on orientifolds (Dai-Leigh-Polchinski 89), one says O-plane for the fixed point locus of the $\mathbb{Z}_2$-involution (see at real space).

O-planes carry D-brane charges in KR-theory (Witten 98), see (DMR 13) for a mathematical account. They serve RR-field tadpole cancellation and as such play a key role in the construction of intersecting D-brane models for string phenomenology.

Properties

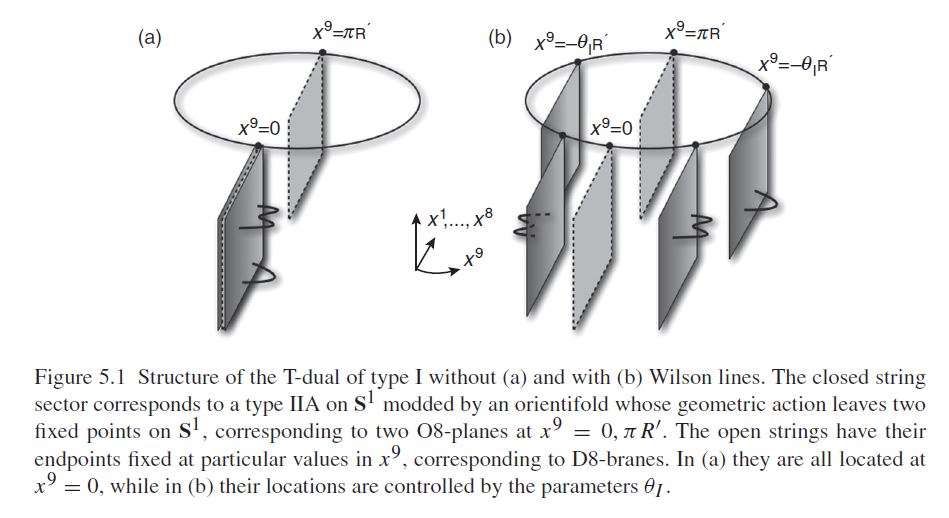
T-Duality with type I string theory

Under T-duality, type I string theory is dual to type II string theory with orientifold planes (reviewed e.g. in Ibanez-Uranga 12, section 5.3.2 - 5.3.4):

O-Plane charge

O-planes carry effective negative RR-charge which may (must) cancel against the actual RR- D-brane charge via RR-field tadpole cancellation.

For flat orientifolds

The charge of the spacetime-filling $O9$-plane of plain type I string theory (type II string theory on the orientifold $\mathbb{R}^{9,1}\sslash \mathbb{Z}_2$ with trival spacetime $\mathbb{Z}_2$-action) is found by worldsheet-computation to be $-32$ in units of D9-brane charge:

(1)$$q_{O9^-} \;=\; -32 \, q_{D9}$$

(e.g. Blumenhagen-Lüst-Theisen 13 (9.83)).

Remark

counting of D-branes on orientifolds

Beware that there is some convention involved in assigning an absolute value of unit D-brane charge $q_{D9}$. A common choice in the literature is to mean by “one D-brane” one of the two pre-images on the covering space, in which case its obsolute charge is to be

(2)$$q_{Dp} \;=\; 1/2$$

(e.g. BDHKMMS 01, p. 46-47). From BLT 13, p. 318:

This means that RR-field tadpole cancellation here requires the presence of 32 D-branes (or rather, by Remark : 16 and their $\mathbb{Z}_2$-mirror images), hence a space-filling D9-brane with Chan-Paton bundle of rank $32$, corresponding to a gauge group SO(32). For more on this see at type I string theory – Tadpole cancellation and SO(32)-GUT.

From this the O$p^-$-brane charge for $p \leq n$ follows from T-duality (as above) with respect to KK-compactification on a d-torus $\mathbb{T}^d$ with $\mathbb{Z}_2$-action given by canonical coordinate reflection

$$\array{ \mathbb{Z}_2 \times \mathbb{R}^{10-d-1,1} \times \mathbb{T}^d &\longrightarrow& \mathbb{R}^{10-d-1,1} \times \mathbb{T}^d \\ (\sigma, (\vec x, \vec y)) &\mapsto& (\vec x, - \vec y) } \,.$$

This results in $O(9-d)^-$-planes with worldvolume $\mathbb{R}^{10-d-1,1}$. But since the orbifold $\mathbb{T}^d\sslash \mathbb{Z}_2$ now has $2^d$ singularities /fixed points (this Example) there are now $2^d$ such $O(9-d)^-$-planes.

Since the number of D-branes does not change under T-duality, the total O-plane charge should be the same as before

$$2^d \cdot q_{O(9-d)} \;=\; 1 \cdot q_{O9} \;=\; -32 \cdot q_{D9} \;=\; - 2^5 \cdot q_{D9}$$

which means that the $O(9-d)^-$-plane charge is

$$q_{O(9-d)^-} \;=\; - 2^{5-d} \cdot q_{D(p-d)}$$

or equivalently

(3)$$q_{O p^-} \;=\; - 2^{ p - 4 } \cdot q_{D p}$$

(e.g. Ibáñez-Uranga 12 (5.52), Blumenhagen-Lüst-Theisen 13 (10.212))

In summary, we have the following table of O-plane charges on flat orbifolds:

O-plane species	charge $q_{O p^-}/q_{D p}$	transverse d-torus	fixed points $\left\vert\left( \mathbb{T}^d\right)^{\mathbb{Z}_2}\right\vert$
$O9^-$	$-32$	$\mathbb{T}^0$	$\phantom{1}1$
$O8^-$	$-16$	$\mathbb{T}^1$	$\phantom{1}2$
$O7^-$	$-\phantom{1}8$	$\mathbb{T}^2$	$\phantom{1}4$
$O6^-$	$-\phantom{1}4$	$\mathbb{T}^3$	$\phantom{1}8$
$O5^-$	$-\phantom{1}2$	$\mathbb{T}^4$	$16$
$O4^-$	$-\phantom{1}1$	$\mathbb{T}^5$	$32$

In particular the O4-plane has negative unit charge (in units of D4-brane charge $q_{D4}$), so that the total charge of $-32$ here comes entirely from the number $32 = 2^5$ of fixed points of the $\mathbb{Z}_2$-action on $\mathbb{T}^5$.

O-plane charges of different dimension may be present

graphics grabbed from Johnson 97

In the presence of discrete torsion

In the presence of discrete torsion in the B-field and/or the RR-fields, this charge structure of orientifold planes on flat orbifolds gets further modified (Hanany-Kol 00, Sec. 2.1, see Bergman-Gimon-Sugimoto 01, Sec. 1):

graphics grabbed from Bergman-Gimon-Sugimoto 01

(In comparing this last table with the above table, notice that this shows the Op-plane charge in units of $q_{Dq} \coloneqq 1/2$ as in (2).)

In differential equivariant KR-theory

A proposal for a formalization of a much more general formula for O-plane charge, regarded in differential equivariant KR-theory is briefly in Distler-Freed-Moore 09, p. 6.

Duality with M-Theory

The possible O-planes in M-theory are $MO1$ ($\leftrightarrow$M-wave), MO5 ($\leftrightarrow$M5-brane) and MO9 (Hanany-Kol 00 around (3.2), HSS 18, Prop. 4.7).

Under the duality between M-theory and type IIA string theory the O8-plane is identified with the MO9 of Horava-Witten theory:

graphics grabbed from GKSTY 02, section 3

while the O4-plane is dual to the MO5 (Hori 98, Gimon 98, Sec. III, AKY 98, Sec. II B, Hanany-Kol 00, 3.1.1)

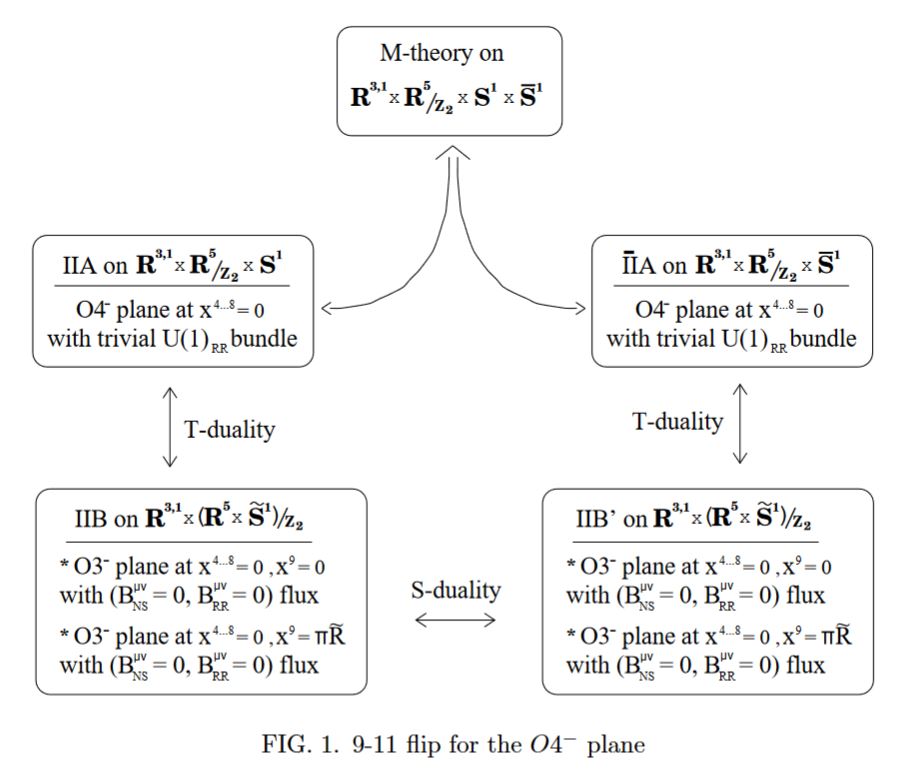
graphics grabbed from Gimon 98

and the $O0$ to the MO1 (Hanany-Kol 00 3.3)

Fractional branes at O-planes

By the discussion at D-branes ending on NS5 branes, a black D6-brane may end on a black NS5-brane, and in fact a priori each brane NS5-brane has to be the junction of two black D6-branes.

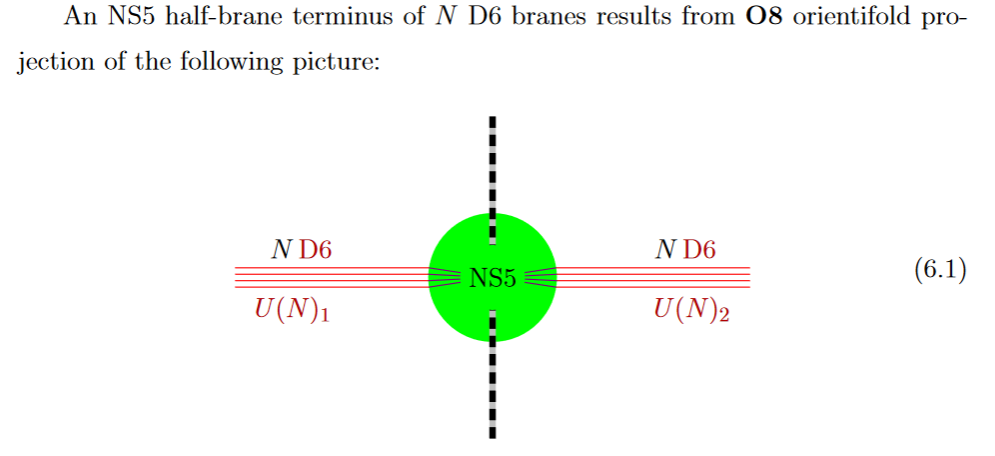
from GKSTY 02

If in addition the black NS5-brane sits at an O8-plane, hence at the orientifold fixed point-locus, then in the ordinary $\mathbb{Z}/2$-quotient it appears as a “half-brane” with only one copy of D6-branes ending on it:

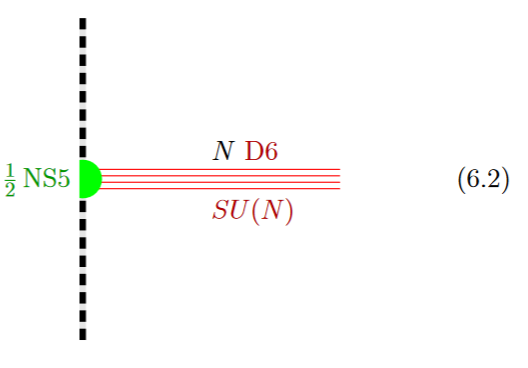
from GKSTY 02

(In Hanany-Zaffaroni 99 this is interpreted in terms of the 't Hooft-Polyakov monopole.)

The lift to M-theory of this situation is an M5-brane intersecting an M9-brane:

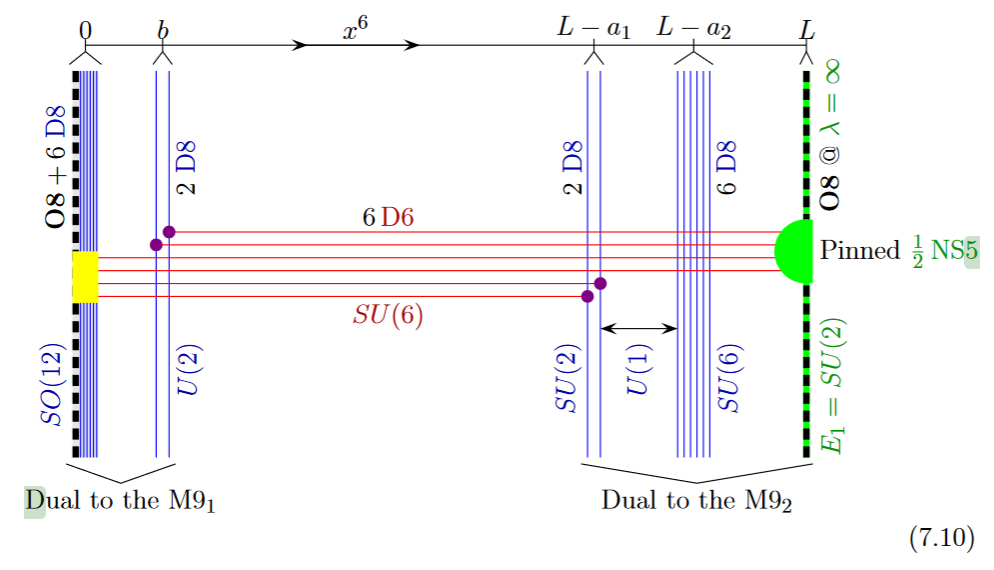
from GKSTY 02

Alternatively the O8-plane may intersect the black D6-branes away from the black NS5-brane:

from HKLY 15

In general, some of the NS5 sit away from the O8-plane, while some sit on top of it:

from Hanany-Zaffaroni 98

See also at intersecting D-brane models the section Intersection of D6s with O8s.

Related concepts

• D-brane

• M-theory lift of gauge enhancement on D6-branes

• NS5 half-branes

References

General

The term “orientifold” originates around

• Jin Dai, R.G. Leigh, Joseph Polchinski, p. 12 of New Connections Between String Theories, Mod.Phys.Lett. A4 (1989) 2073-2083 (spire:25758)

Other early accounts include

• Clifford Johnson, Anatomy of a Duality, Nucl.Phys. B521 (1998) 71-116 (arXiv:hep-th/9711082)

• Amihay Hanany, Alberto Zaffaroni, Branes and Six Dimensional Supersymmetric Theories, Nucl.Phys. B529 (1998) 180-206 (arXiv:hep-th/9712145)

• Edward Witten, section 5 of D-branes and K-theory, J. High Energy Phys., 1998(12):019, 1998 (arXiv:hep-th/9810188)

• Sunil Mukhi, Nemani V. Suryanarayana, Gravitational Couplings, Orientifolds and M-Planes, JHEP 9909 (1999) 017 (arXiv:hep-th/9907215)

• Yoshifumi Hyakutake, Yosuke Imamura, Shigeki Sugimoto, Orientifold Planes, Type I Wilson Lines and Non-BPS D-branes, JHEP 0008 (2000) 043 (arXiv:hep-th/0007012)

• Jan de Boer, Robbert Dijkgraaf, Kentaro Hori, Arjan Keurentjes, John Morgan, David Morrison, Savdeep Sethi, section 3 of Triples, Fluxes, and Strings, Adv.Theor.Math.Phys. 4 (2002) 995-1186 (arXiv:hep-th/0103170)

Textbook accounts:

• Luis Ibáñez, Angel Uranga, section 10 of String Theory and Particle Physics – An Introduction to String Phenomenology, Cambridge 2012

• Ralph Blumenhagen, Dieter Lüst, Stefan Theisen, Section 9.4 and 10.6 of Basic Concepts of String Theory Part of the series Theoretical and Mathematical Physics, Springer 2013

See also

• Wikipedia, Orientifold

With discrete torsion

O-Plane charge in the presence of discrete torsion:

• Hanany-Kol 00

• Oren Bergman, Eric Gimon, Shigeki Sugimoto, Orientifolds, RR Torsion, and K-theory, JHEP 0105:047, 2001 (arXiv:hep-th/0103183)

• Atish Dabholkar, Jaemo Park, Strings on Orientifolds, Nucl. Phys. B477 (1996) 701-714 (arXiv:hep-th/9604178)

In terms of KO-theory

O-Plane charge in differential equivariant KR-theory:

• Charles Doran, Kevin Iga, Greg Landweber, Stefan Méndez-Diez, Geometrization of $\mathcal{N}$-Extended 1-Dimensional Supersymmetry Algebras, Adv. Theor. Math. Phys. 19 5 (2015) 1043-1113 [arXiv:1311.3736, doi:10.4310/ATMP.2015.v19.n5.a4, pdf]

• Charles Doran, Kevin Iga, Jordan Kostiuk, Stefan Méndez-Diez, Geometrization of $\mathcal{N}$-Extended 1-Dimensional Supersymmetry Algebras II, Adv. Theor. Math> Physics 22 3 (2018) [arXiv:1610.09983]

• Jacques Distler, Dan Freed, Greg Moore, Orientifold Précis in: Hisham Sati, Urs Schreiber (eds.) Mathematical Foundations of Quantum Field and Perturbative String Theory Proceedings of Symposia in Pure Mathematics, AMS (2011) (arXiv:0906.0795, slides)

• Jacques Distler, Dan Freed, Greg Moore, Spin structures and superstrings (arXiv:1007.4581)

reviewed/surveyed in

• Daniel Freed, Dirac charge quantiation, K-theory, and orientifolds, talk at a workshop Mathematical methods in general relativity and quantum field theories, Paris, November 2009 (pdf, pdf)

• Greg Moore, The RR-charge of an orientifold, Oberwolfach talk 2010 (pdf, pdf, ppt)

• Daniel Freed, Lectures on twisted K-theory and orientifolds, lecures at K-Theory and Quantum Fields, ESI 2012 (pdf)

Actual construction of twisted differential orthogonal K-theory and its relation to D-brane charge in type I string theory (on orientifolds):

• Daniel Grady, Hisham Sati, Twisted differential KO-theory (arXiv:1905.09085)

Examples / Models

The Witten-Sakai-Sugimoto model for QCD on O-planes:

• Toshiya Imoto, Tadakatsu Sakai, Shigeki Sugimoto, $O(N)$ and $USp(N)$ QCD from String Theory, Prog.Theor.Phys.122:1433-1453, 2010 (arXiv:0907.2968)

Lift to M-theory

Lift to M-theory (MO5, MO9):

• Kentaro Hori, Consistency Conditions for Fivebrane in M Theory on $\mathbb{R}^5/\mathbb{Z}_2$ Orbifold, Nucl.Phys.B539:35-78, 1999 (arXiv:hep-th/9805141)

• Eric Gimon, On the M-theory Interpretation of Orientifold Planes (arXiv:hep-th/9806226, spire:472499)

• Changhyun Ahn, Hoil Kim, Hyun Seok Yang, $SO(2N)$ $(0,2)$ SCFT and M Theory on $AdS_7 \times \mathbb{R}P^4$, Phys.Rev. D59 (1999) 106002 (arXiv:hep-th/9808182)

• E. Gorbatov, V.S. Kaplunovsky, J. Sonnenschein, Stefan Theisen, S. Yankielowicz, section 3 of On Heterotic Orbifolds, M Theory and Type I’ Brane Engineering, JHEP 0205:015, 2002 (arXiv:hep-th/0108135)

• Amihay Hanany, Barak Kol, On Orientifolds, Discrete Torsion, Branes and M Theory, JHEP 0006 (2000) 013 (arXiv:hep-th/0003025)

• Hirotaka Hayashi, Sung-Soo Kim, Kimyeong Lee, Futoshi Yagi, 6d SCFTs, 5d Dualities and Tao Web Diagrams (arXiv:1509.03300)

• John Huerta, Hisham Sati, Urs Schreiber, Real ADE-equivariant (co)homotopy and Super M-branes (arXiv:1805.05987)

The intersection with (p,q)5-brane webs:

• Hirotaka Hayashi, Sung-Soo Kim, Kimyeong Lee, Masato Taki, Futoshi Yagi, More on 5d descriptions of 6d SCFTs, JHEP10 (2016) 126 (arXiv:1512.08239)

• Amihay Hanany, Alberto Zaffaroni, Issues on Orientifolds: On the brane construction of gauge theories with $SO(2n)$ global symmetry, JHEP 9907 (1999) 009 (arXiv:hep-th/9903242)

• Gabi Zafrir, Brane webs in the presence of an $O5^-$-plane and 4d class S theories of type D, JHEP07 (2016) 035 (arXiv:1602.00130)