nLab D6-brane
Context
String theory
Ingredients
Critical string models
Extended objects
Topological strings
Backgrounds
Phenomenology
Contents
Idea
The D-brane of dimension 6+1 in type IIA string theory.
Relation to other branes
To the KK-monopole in M-theory
The lift of the D6 to M-theory is the Kaluza-Klein monopole there (Townsend 95).
One way to understand this is as follows: the first Chern class of the M-theory circle bundle is the D0-brane degree-2 RR-field strength . By the self-duality of the RR-fields this is the Hodge dual of the D6-brane degree-8 field strength. Now given a Kaluza-Klein monopole after removing the locus of the monopole where the circle-fiber degenerates (as in the original Dirac charge quantization argument) it is a non-degenerated circle bundle such that the integral of the 2-form over any 2-sphere surrounding the singular locus is non-trivial. By the usual yoga of electric-magnetic duality this integral measures the magnetic flux of a D6-brane sitting at the singular locus. For more on this see at Kaluza-Klein monopole – Relation to the D6-brane, and see at M-theory lift of gauge enhancement on D6-branes.
To D7-branes
The T-dual of the D6 branes is the D7-brane in type IIB string theory.
Bound states
bound states/brane intersections involving D6-branes:
Table of branes
from M-branes to F-branes: superstrings, D-branes and NS5-branes
(e.g. Johnson 97, Blumenhagen 10)
Phenomenology – Intersecting D-brane models
D6-branes are the main ingredient in type IIA string phenomenology in the guise of intersecting D-brane models, see also at string phenomenology the section Models in type II with intersecting branes.
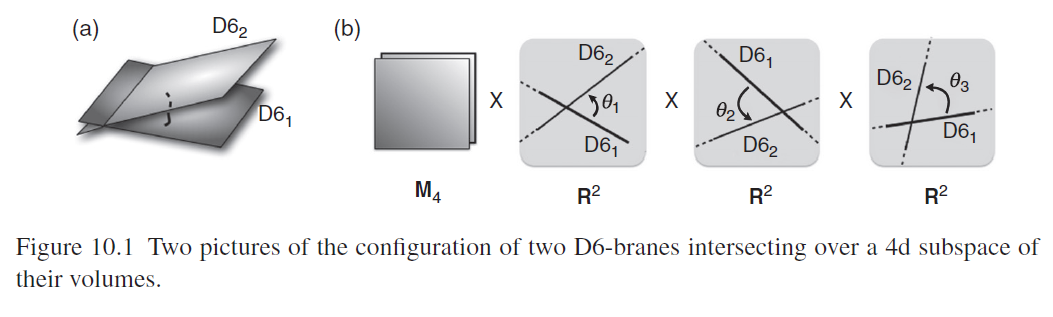
graphics grabbed from Ibáñez-Uranga 12
Related concept
Table of branes appearing in supergravity/string theory (for classification see at brane scan).
References
The relation to the Kaluza-Klein monopole in M-theory is due to
- Paul Townsend, The eleven-dimensional supermembrane revisited, Phys.Lett.B350:184-187,1995 (arXiv:hep-th/9501068)
Further discussion and derivation of the Chan-Paton gauge field content on the D6 from the KK-monopole is discussed in
-
César Gómez, Juan José Manjarín, A note on the dyonic D6-brane, 6th International Workshop on Conformal Field Theory and Integrable Models, Landau Institute, Sept. 2002 (arXiv:hep-th/0302096)
-
Juan José Manjarín, Topics on D-brane charges with B-fields, Int. J. Geom. Meth. Mod. Phys. 1 (2004) (arXiv:hep-th/0405074)
Discussion of D6 intersecting branes for intersecting D-brane models:
-
Luis Ibáñez, Angel Uranga, String Theory and Particle Physics – An Introduction to String Phenomenology, Cambridge 2012
-
Angel Uranga, Model building in IIA: Intersecting brane worlds, 2012 (pdf)
Last revised on November 26, 2019 at 15:34:06. See the history of this page for a list of all contributions to it.
 (
(