nLab moduli space of monopoles

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Discuss this page |
Redirected from "monopoles in Yang-Mills theory".
Contents

Context

Chern-Weil theory

Quantum Field Theory

algebraic quantum field theory (perturbative, on curved spacetimes, homotopical)

Introduction

Concepts

field theory:

Lagrangian field theory

quantization

quantum mechanical system, quantum probability

free field quantization

gauge theories

interacting field quantization

renormalization

Theorems

States and observables

Operator algebra

Local QFT

Perturbative QFT

Contents

Idea

By the Nahm transform, the moduli space of x 4x^4-translation invariant self-dual Yang-Mills theory solitons on 4d Euclidean space ℝ 4\mathbb{R}^{4} is equivalently the space of solutions to the Bogomolny equations on 3d Euclidean space, which in turn may be thought of as magnetic monopoles in 3d Euclidean Yang-Mills theory coupled to a charged scalar field (a “Higgs field”). Therefore this moduli space is traditionally referred to simply as the moduli space of magnetic monopoles (e.g. Atiyah-Hitchin 88) or just the moduli space of monopoles.

Definition

The moduli space

(1)ℳ k≔⋯ \mathcal{M}_k \;\coloneqq\; \cdots

of kk monopoles is … (Atiyah-Hitchin 88, p. 15-16).

Properties

Scattering amplitudes of monopoles

Write

(2)Maps cplxrtnl */(ℂP 1,ℂP 1) k⊂Maps cplxrtnl */(ℂP 1,ℂP 1)⊂Maps */(S 2,S 2) Maps_{cplx\,rtnl}^{\ast/}\big( \mathbb{C}P^1, \mathbb{C}P^1 \big)_k \;\subset\; Maps_{cplx\,rtnl}^{\ast/}\big( \mathbb{C}P^1, \mathbb{C}P^1 \big) \;\subset\; Maps^{\ast/}\big( S^2, S^2 \big)

for the space of pointed rational functions from the Riemann sphere to itself, of degree k∈ℕk \in \mathbb{N}, inside the full Cohomotopy cocycle space. The homotopy type of this mapping space is discussed in Segal 79, see homotopy of rational maps.

To each configuration c∈ℳ k c \in \mathcal{M}_k of k∈ℕk \in \mathbb{N} magnetic monopoles is associated a scattering amplitude

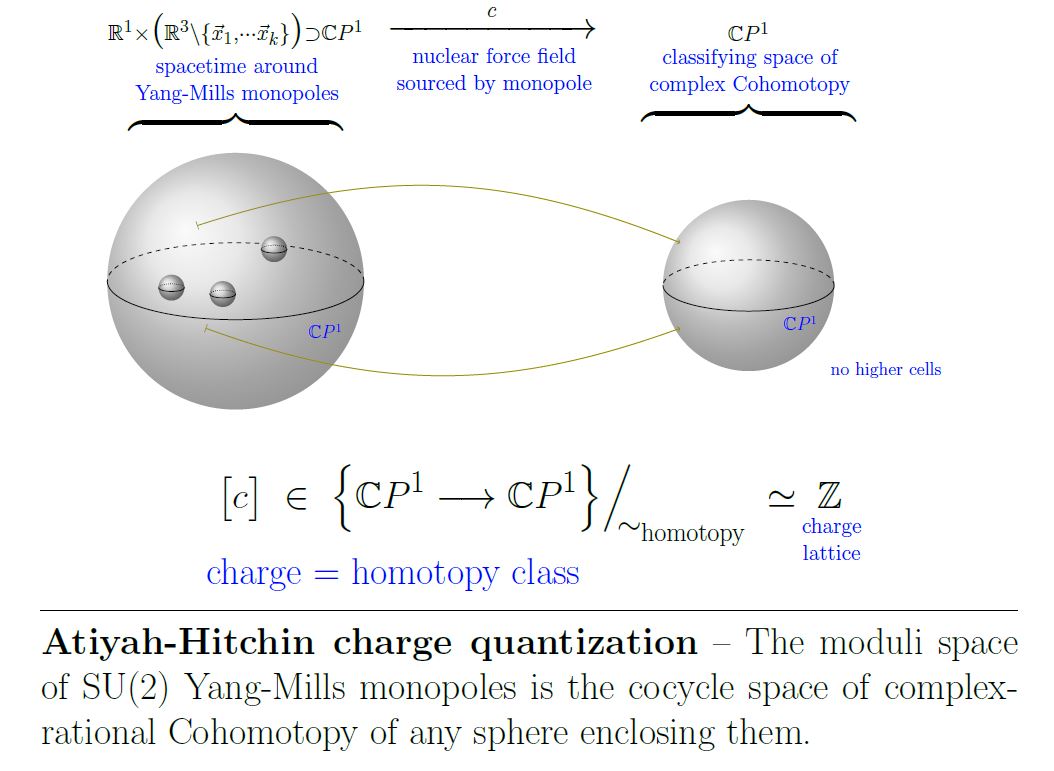
(3)S(c)∈Maps cplxrtnl */(ℂP 1,ℂP 1) k S(c) \in Maps_{cplx\,rtnl}^{\ast/}\big( \mathbb{C}P^1, \mathbb{C}P^1 \big)_k

(Atiyah-Hitchin 88 (2.8))


Charge quantization in Cohomotopy

Proposition

(moduli space of k monopoles is space of degree kk complex-rational functions from Riemann sphere to itself)

The assignment (3) is a diffeomorphism identifying the moduli space (1) of kk magnetic monopoles with the space (2) of complex-rational functions from the Riemann sphere to itself, of degree kk (hence the cocycle space of complex-rational 2-Cohomotopy)

ℳ k⟶≃ diffSMaps cplxrtnl */(ℂP 1,ℂP 1) k \mathcal{M}_k \; \underoverset{ \simeq_{diff} }{ S }{ \;\;\longrightarrow\;\; } \; Maps_{cplx\,rtnl}^{\ast/} \big( \mathbb{C}P^1, \mathbb{C}P^1 \big)_k

(due to Donaldson 84, see also Atiyah-Hitchin 88, Theorem 2.10).

Proposition

(space of degree kk complex-rational functions from Riemann sphere to itself is k-equivalent to Cohomotopy cocycle space in degree kk)

The inclusion of the complex rational self-maps maps of degree kk into the full based space of maps of degree kk (hence the kk-component of the second iterated loop space of the 2-sphere, and hence the plain Cohomotopy cocycle space) induces an isomorphism of homotopy groups in degrees ≤k\leq k (in particular a k-equivalence):

Maps cplxrtnl */(ℂP 1,ℂP 1) k↪≃ ≤kMaps */(S 2,S 2) k Maps_{cplx\,rtnl}^{\ast/} \big( \mathbb{C}P^1, \mathbb{C}P^1 \big)_k \; \underset{ \simeq_{\leq k} }{ \;\;\hookrightarrow\;\; } \; Maps^{\ast/}\big( S^2, S^2 \big)_k

(Segal 79, Prop. 1.1, see at homotopy of rational maps)

Hence, Prop. and Prop. together say that the moduli space of kk-monopoles is kk-equivalent to the Cohomotopy cocycle space π 2(S 2) k\mathbf{\pi}^2\big( S^2 \big)_k.

ℳ k⟶≃ diffSMaps cplxrtnl */(ℂP 1,ℂP 1) k↪≃ ≤kMaps */(S 2,S 2) k \mathcal{M}_k \; \underoverset{ \simeq_{diff} }{ S }{ \;\;\longrightarrow\;\; } \; Maps_{cplx\,rtnl}^{\ast/} \big( \mathbb{C}P^1, \mathbb{C}P^1 \big)_k \; \underset{ \simeq_{\leq k} }{ \;\;\hookrightarrow\;\; } \; Maps^{\ast/}\big( S^2, S^2 \big)_k

This is a non-abelian analog of the Dirac charge quantization of the electromagnetic field, with ordinary cohomology replaced by Cohomotopy cohomology theory:

\,


Relation to braid groups

Proposition

(moduli space of monopoles is stably weak homotopy equivalent to classifying space of braid group)

For k∈ℕk \in \mathbb{N} there is a stable weak homotopy equivalence between the moduli space of k monopoles (?) and the classifying space of the braid group Braids 2kBraids_{2k} on 2k2 k strands:

Σ ∞ℳ k≃Σ ∞Braids 2k \Sigma^\infty \mathcal{M}_k \;\simeq\; \Sigma^\infty Braids_{2k}

(Cohen-Cohen-Mann-Milgram 91)


Geometric engineering by Dpp-D(p+2)(p+2)-brane intersections

Generally Dp-D(p+2)-brane intersections geometrically engineer Yang-Mills monopoles in the worldvolume of the higher dimensional D(p+2)(p+2)-branes.

Specifically for p=6p = 6, i.e. for D6-D8-brane intersections, this fits with the Witten-Sakai-Sugimoto model geometrically engineering quantum chromodynamics, and then gives a geometric engineering of the Yang-Mills monopoles in actual QCD (HLPY 08, p. 16).

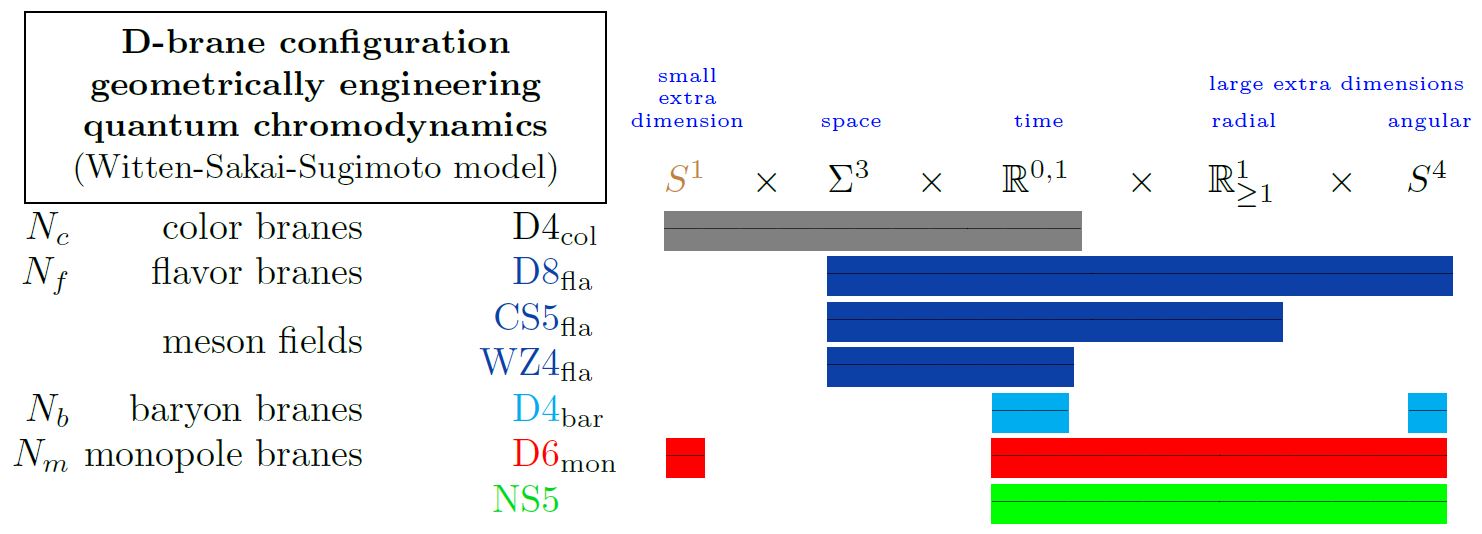
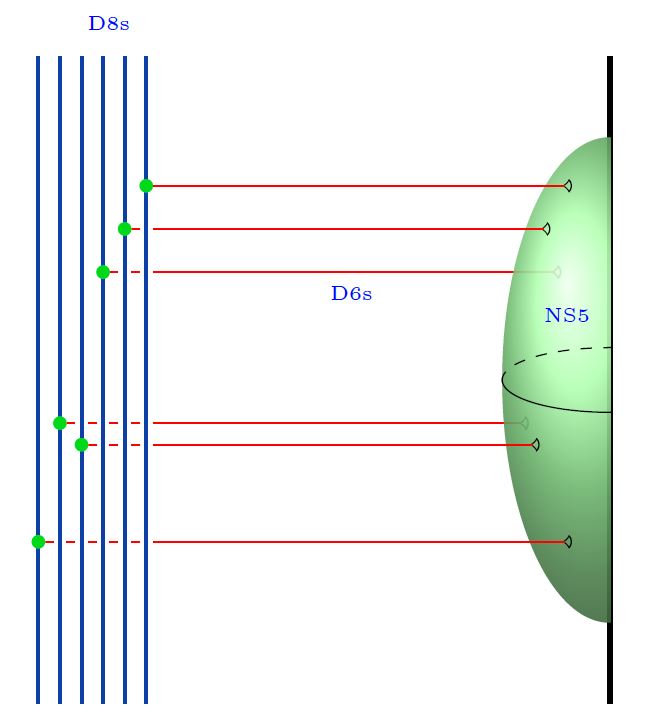
graphics from Sati-Schreiber 19c

Here we are showing

  1. the color D4-branes;

  2. the flavor D8-branes;

    with

    1. the 5d Chern-Simons theory on their worldvolume

    2. the corresponding 4d WZW model on the boundary

    both exhibiting the meson fields

  3. the baryon D4-branes

    (see below at WSS – Baryons)

  4. the Yang-Mills monopole D6-branes

    (see at D6-D8-brane bound state)

  5. the NS5-branes.


moduli spaces

References

General

See also:

On the ordinary cohomology of the moduli space of YM-monopoles:

The special case of gauge group SU(3):

Identification of Yang-Mills monopoles with rational maps

The following lists references concerned with the identification of the (extended) moduli space of Yang-Mills monopoles (in the BPS limit, i.e. for vanishing Higgs potential) with a mapping space of complex rational maps from the complex plane, equivalently holomorphic maps from the Riemann sphere ℂP 1\mathbb{C}P^1 (at infinity in ℝ 3\mathbb{R}^3) to itself (for gauge group SU(2)) or generally to a complex flag variety such as (see Ionnadou & Sutcliffe 1999a for review) to a coset space by the maximal torus (for maximal symmetry breaking) or to complex projective space ℂP n−1\mathbb{C}P^{n-1} (for gauge group SU(n) and minimal symmetry breaking).

The identification was conjectured (following an analogous result for Yang-Mills instantons) in:

Full understanding of the rational map involved as “scattering data” of the monopole is due to:

The identification with (pointed) holomorphic functions out of ℂ P 1 \mathbb{C}P^1 was proven…

…for the case of gauge group SU ( 2 ) SU(2) (maps to ℂ P 1 \mathbb{C}P^1 itself) in

…for the more general case of classical gauge group with maximal symmetry breaking (maps to the coset space by the maximal torus) in:

… for the fully general case of semisimple gauge groups with any symmetry breaking (maps to any flag varieties) in

and for un-pointed maps in

Further discussion:

Review:

  • Alexander B. Atanasov, Magnetic monopoles and the equations of Bogomolny and Nahm (pdf), chapter 5 in: Magnetic Monopoles, ‘t Hooft Lines, and the Geometric Langlands Correspondence, 2018 (pdf, slides)

On the relevant homotopy of rational maps (see there for more references):

As transversal Dpp/D(p+2)(p+2)-brane intersections

In string theory Yang-Mills monopoles are geometrically engineeted as transversally intersecting Dp-D(p+2)-brane bound states:

For transversal D1-D3-brane bound states:

For transversal D2-D4 brane intersections (with an eye towards AdS/QCD):

  • Alexander Gorsky, Valentin Zakharov, Ariel Zhitnitsky, On Classification of QCD defects via holography, Phys. Rev. D79:106003, 2009 (arxiv:0902.1842)

For transversal D3-D5 brane intersections:

For transversal D6-D8-brane intersections (with an eye towards AdS/QCD):

  • Deog Ki Hong, Ki-Myeong Lee, Cheonsoo Park, Ho-Ung Yee, Section V of: Holographic Monopole Catalysis of Baryon Decay, JHEP 0808:018, 2008 (https:arXiv:0804.1326)

With emphasis on half NS5-branes in type I' string theory:

The moduli space of monopoles appears also in the KK-compactification of the M5-brane on a complex surface (AGT-correspondence):

  • Benjamin Assel, Sakura Schafer-Nameki, Jin-Mann Wong, M5-branes on S 2×M 4S^2 \times M_4: Nahm’s Equations and 4d Topological Sigma-models, J. High Energ. Phys. (2016) 2016: 120 (arxiv:1604.03606)

As Coulomb branches of D=3D=3 𝒩=4\mathcal{N}=4 SYM

Identification of the Coulomb branch of D=3 N=4 super Yang-Mills theory with the moduli space of monopoles in Yang-Mills theory:

Rozansky-Witten invariants

Discussion of Rozansky-Witten invariants of moduli spaces of monopoles:

Relation to braids

Relation to braid groups:

Relation of Dp-D(p+2)-brane bound states (hence Yang-Mills monopoles) to Vassiliev braid invariants via chord diagrams computing radii of fuzzy spheres:

Last revised on September 4, 2021 at 10:24:01. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (35 revisions) Cite Print Source