nLab Spin(6)
Context
Group Theory
- group, ∞-group
- group object, group object in an (∞,1)-category
- abelian group, spectrum
- super abelian group
- group action, ∞-action
- representation, ∞-representation
- progroup
- homogeneous space
Classical groups
Finite groups
Group schemes
Topological groups
Lie groups
Super-Lie groups
Higher groups
Cohomology and Extensions
Related concepts
Spin geometry
spin geometry, string geometry, fivebrane geometry …
Ingredients
Spin geometry
rotation groups in low dimensions:
see also
String geometry
Fivebrane geometry
Ninebrane geometry
Contents
Idea
The spin group in dimension 6.
Properties
Exceptional isomorphism
Proposition
There is an exceptional isomorphism
between Spin(6) and SU(4), reflecting, under the classification of simple Lie groups, the coincidence of the Dynkin diagrams “D3” and A3.
(e.g. Figueroa-O’Farrill 10, Lemma 8.1)
One way to see the isomorphism is as follows. Let be a 4-dimensional complex vector space with an inner product and a compatible complex volume form, meaning an element of the exterior product whose norm is 1 in the norm coming from the inner product on . The inner product defines a conjugate-linear isomorphism (with the complex dual vector space) that together with the complex volume form can be used to define a conjugate-linear Hodge star operator on . This Hodge star operator squares to the identity, and its and eigenspaces, say , each become 6-dimensional real inner product spaces in a natural way. Thus, the group , consisting of all complex-linear transformations of that preserve the inner product and complex volume form, acts as linear transformations of that preserve the inner product, giving a homomorphism . Since is connected we in fact have .
Specializing to the case we get a Lie group homomorphism . Since is nonzero and is simple, must be injective. Since
must also be surjective. Since is connected and is a bijection, must be a covering map. Since , exhibits as a connected cover of that is at least a double cover. But the universal cover of , namely , is only a double cover. Thus is a double cover of , and .
Coset spaces
coset space-structures on n-spheres:
| standard: | |
|---|---|
| this Prop. | |
| this Prop. | |
| this Prop. | |
| exceptional: | |
| Spin(7)/G₂ is the 7-sphere | |
| since Spin(6) SU(4) | |
| since Sp(2) is Spin(5) and Sp(1) is SU(2), see Spin(5)/SU(2) is the 7-sphere | |
| G₂/SU(3) is the 6-sphere | |
| Spin(9)/Spin(7) is the 15-sphere |
see also Spin(8)-subgroups and reductions
homotopy fibers of homotopy pullbacks of classifying spaces:
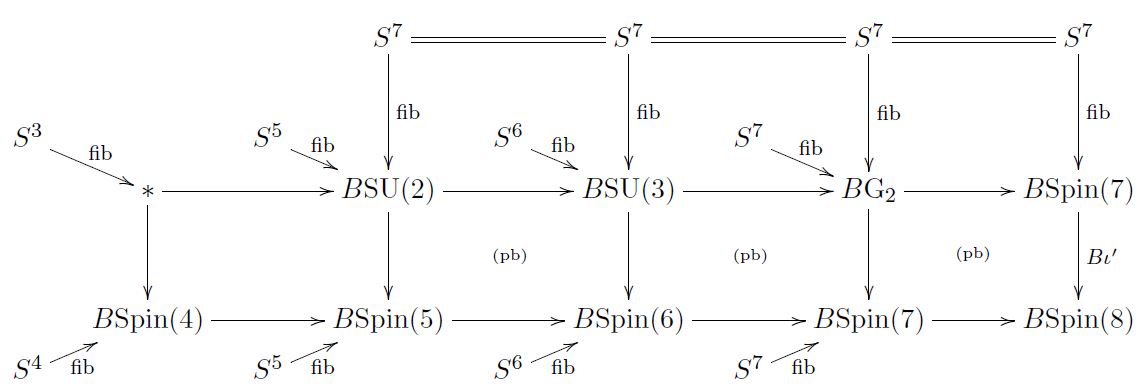
(from FSS 19, 3.4)
-Structure and exceptional geometry
Spin(8)-subgroups and reductions to exceptional geometry
see also: coset space structure on n-spheres
Related concepts
rotation groups in low dimensions:
see also
References
- José Figueroa-O'Farrill, PG course on Spin Geometry lecture 8: Parallel and Killing spinors, 2010 (pdf)
Last revised on August 17, 2021 at 06:19:55. See the history of this page for a list of all contributions to it.