nLab Dyson formula
Context
Quantum systems
-
quantum algorithms:
Contents
In quantum mechanics
In quantum mechanics what is called the Dyson formula is what in mathematics is called the iterated integral-expression for parallel transport: It an expression for the solution to a differential equation of the form
where is a one-parameter family of elements of some Hilbert space and is a one-parameter family of linear operators on this Hilbert space.
This appears prominently in the expression of the Schrödinger equation in the interaction picture, in which case is the interaction Hamiltonian in the Heisenberg picture of the free theory. In this case the Dyson series gives the S-matrix. This is the context in which the term “Dyson formula” orginates. But of course also the plain Schrödinger equation (“in the Schrödinger picture”) is already of this form if the Hamiltonian is time-dependent.
The idea is to think of the solution as given by the limit
and to think of this as the result of forming the exponential expression and then re-ordering in the resulting sum of products all the factors of such that they are time ordered with larger values of ordered to the left of smaller values.
Accordingly, in the physics literature solutions to this equation are written
with the time ordering operator producing time-ordered products. The corresponding series of iterated integrals
is called the Dyson series.
In quantum field theory
The idea generalizes to relativistic field theory perturbative quantum field theory on Lorentzian spacetimes if due care is exercised (including adiabatic switching and point extension of operator-valued distributions). Here the “time ordering” is generalized to a causal ordering, this is the content of the construction of the S-matrix and the interacting field algebra in causal perturbation theory.
See at time-ordered product and S-matrix for details.
Examples
Kontsevich integral is Dyson formula of KZ-connection
For the Definition of the Knizhnik-Zamolodchikov connection we need the following notation:
-
configuration spaces of points
For write
(1)for the ordered configuration space of n points in the plane, regarded as a smooth manifold.
Identifying the plane with the complex plane , we have canonical holomorphic coordinate functions
(2) -
(3)
for the quotient vector space of the linear span of horizontal chord diagrams on strands by the 4T relations (infinitesimal braid relations), regarded as an associative algebra under concatenation of strands (here).
Definition
The universal Knizhnik-Zamolodchikov form is the horizontal chord diagram-algebra valued differential form (3) on the configuration space of points (1)
given in the canonical coordinates (2) by:
where
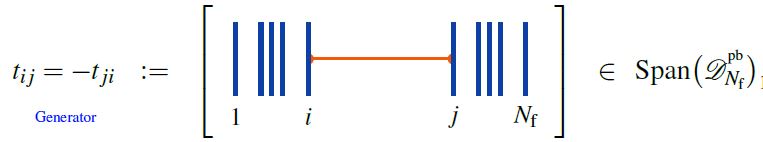
is the horizontal chord diagram with exactly one chord, which stretches between the th and the th strand.
Regarded as a connection form for a connection on a vector bundle, this defines the universal Knizhnik-Zamolodchikov connection , with covariant derivative
for any smooth function
with values in modules over the algebra of horizontal chord diagrams modulo 4T relations.
The condition of covariant constancy
is called the Knizhnik-Zamolodchikov equation.
Finally, given a metric Lie algebra and a tuple of Lie algebra representations
the corresponding endomorphism-valued Lie algebra weight system
turns the universal Knizhnik-Zamolodchikov form (4) into a endomorphism ring-valued differential form
The universal formulation (4) is highlighted for instance in Bat-Natan 95, Section 4.2, Lescop 00, p. 7. Most authors state the version after evaluation in a Lie algebra weight system, e.g. Kohno 14, Section 5.
Proposition
(Knizhnik-Zamolodchikov connection is flat)
The Knizhnik-Zamolodchikov connection (Def. ) is flat:
Proposition
(Kontsevich integral for braids)
The Dyson formula for the holonomy of the Knizhnik-Zamolodchikov connection (Def. ) is called the Kontsevich integral on braids.
(e.g. Lescop 00, side-remark 1.14)
Related concepts
References
Relation to the Magnus expansion:
- Michel Bauer, Raphael Chetrite, Kurusch Ebrahimi-Fard, Frederic Patras: Time-ordering and a generalized Magnus expansion, Letters in Mathematical Physics 103 (2013) 331 [doi:10.1007/s11005-012-0596-z, arXiv:1206.3990]
Last revised on August 30, 2024 at 12:15:19. See the history of this page for a list of all contributions to it.