nLab tree tensor network state

Idea

What are called tree tensor network states (TTN states) in quantum physics (specifically in solid state physics and in AdS/CFT) are those tensor network states of the form of tree, often considered of fixed valence.

For example, given a metric Lie algebra $\mathfrak{g}$ (with string diagram-notation as discussed there) its Lie bracket-tensor $f \coloneqq [-,-] \in \mathfrak{g}^\ast \otimes \mathfrak{g}^\ast \otimes \mathfrak{g}$ gives rise to tree tensor network of the following form:

graphics from Sati-Schreiber 19c

The tree tensor network states in the form of Bruhat-Tits trees play a special role in the AdS/CFT correspondence, either as

1. a kind of lattice QFT-approximation to an actual boundary CFT quantum state,

2. as the p-adic geometric incarnation of anti de Sitter spacetime in the p-adic AdS/CFT correspondence,

3. as a reflection of actual crystal-site quantum states in AdS/CFT in solid state physics:

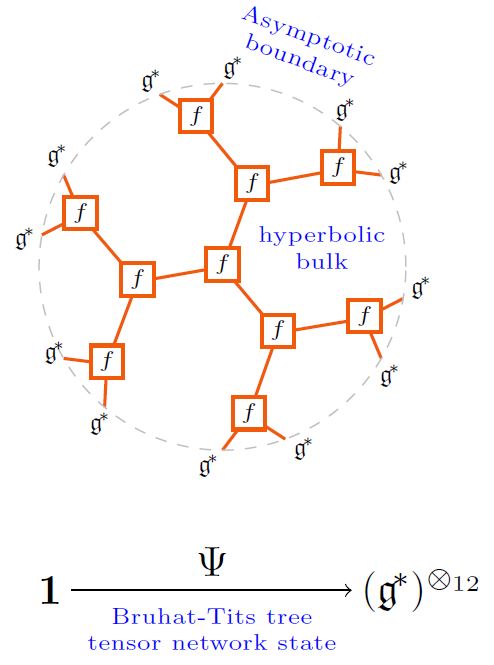
graphics from Sati-Schreiber 19c

(As in HMSS 16, HLM 19. But maybe one wants the Poincaré-dual networks, instead, as in HMPS 18?)

Related concepts

quantum probability theory – observables and states

• states

  • classical state

  • quantum state

    • space of states (in geometric quantization)

    • state on a star-algebra, quasi-state

    • qbit, Bell state

      dimer, tensor network state

    • quantum state preparation

    • probability amplitude, quantum fluctuation

    • pure state

      • wave function

      • bra-ket

      • Bell state

    • quantum superposition, quantum interference

    • quantum entanglement

    • quantum measurement

      wave function collapse

      Born rule

      deferred measurement principle

      quantum reader monad

      measurement problem

    • superselection sector

    • mixed state, density matrix

      entanglement entropy

      holographic entanglement entropy

    • coherent quantum state

  • ground state, excited state

  • quasi-free state

  • Fock space, second quantization

  • vacuum, vacuum state

    • Hadamard state

    • vacuum diagram

    • vacuum expectation value, vacuum amplitude, vacuum fluctuation

    • vacuum energy

    • vacuum polarization

    • interacting vacuum

    • thermal vacuum, KMS state

    • vacuum stability

      • false vacuum, tachyon, Coleman-De Luccia instanton

    • theta vacuum

    • perturbative string theory vacuum

      • non-geometric string theory vacuum

      • landscape of string theory vacua

  • entangled state

  • tensor network state

    • matrix product state

    • tree tensor network state

• observables

  • quantum observable, beable

  • algebra of observables, star-algebra

  • Bohr topos

  • quantum operator (in geometric quantization)

  • quantum operation, quantum effect, effect algebra

  • in quantum field theory

    • local observable

    • polynomial observable

      • linear observable

        • field observable

    • regular observable

    • microcausal observable

    • normal-ordered product, time-ordered products, retarded product

    • Wick algebra

    • scattering amplitude

    • interacting field algebra of observables, Bogoliubov's formula

• GNS construction

• theorems

  • order-theoretic structure in quantum mechanics

    • Gleason's theorem

    • Alfsen-Shultz theorem

    • Harding-Döring-Hamhalter theorem

    • Kochen-Specker theorem

    • Nuiten's lemma

  • Wigner's theorem

  • no-cloning theorem

  • Bell's theorem

References

General

For more see the references at tensor network state.

• Shi-Ju Ran, Emanuele Tirrito, Cheng Peng, Xi Chen, Luca Tagliacozzo, Gang Su, Maciej Lewenstein, Section 2.3.3 of: Tensor Network Contractions, Lecture Notes in Physics, Springer (2020) (arXiv:1708.09213, doi:10.1007/978-3-030-34489-4)

Application to entanglement entropy

Application to entanglement entropy;

• Luca Tagliacozzo, G. Evenbly, Guifre Vidal,

  Simulation of two-dimensional quantum systems using a tree tensor network that exploits the entropic area law, Phys. Rev. B 80, 235127 (2009) (arXiv:0903.5017)

Application in solid state physics

Application in solid state physics:

• Valentin Murg, Örs Legeza, Reinhard M. Noack, Frank Verstraete, Simulating Strongly Correlated Quantum Systems with Tree Tensor Networks, Phys. Rev. B 82, 205105 (2010) (arXiv:1006.3095)

Application in quantum chemistry

For application in quantum chemistry:

• Naoki Nakatani, Garnet Kin-Lic Chan, Efficient Tree Tensor Network States (TTNS) for Quantum Chemistry: Generalizations of the Density Matrix Renormalization Group Algorithm, J. Chem. Phys. 138, 134113 (2013) (arXiv:1302.2298)

• Klaas Gunst, Frank Verstraete, Sebastian Wouters, Örs Legeza, Dimitri Van Neck, T3NS: three-legged tree tensor network states, Chem. Theory Comput. 2018, 14, 4, 2026-2033 (arXiv:1801.09998)

• Henrik R. Larsson, Computing vibrational eigenstates with tree tensor network states (TTNS), J. Chem. Phys. 151, 204102 (2019) (arXiv:1909.13831)

Application to $p$-adic AdS/CFT correspondence:

Discussion of Bruhat-Tits tree-tensor networks in the context of the p-adic AdS/CFT correspondence:

• Arpan Bhattacharyya, Ling-Yan Hung, Yang Lei, Wei Li, Tensor network and ($p$-adic) AdS/CFT, JHEP 1801 (2018) 139 (arXiv:1703.05445)

• Ling-Yan Hung, Wei Li, Charles M. Melby-Thompson, $p$-adic CFT is a holographic tensor network (arXiv:1902.01411)

Application in machine learning

Application in machine learning:

• Ding Liu, Shi-Ju Ran, Peter Wittek, Cheng Peng, Raul Blázquez García, Gang Su, Maciej Lewenstein, Machine Learning by Unitary Tensor Network of Hierarchical Tree Structure, New Journal of Physics, 21, 073059 (2019) (arXiv:1710.04833)

• Song Cheng, Lei Wang, Tao Xiang, Pan Zhang, Tree Tensor Networks for Generative Modeling, Phys. Rev. B 99, 155131 (2019) (arXiv:1901.02217)