nLab twisted cohomotopy
Context
Homotopy theory
homotopy theory, (∞,1)-category theory, homotopy type theory
flavors: stable, equivariant, rational, p-adic, proper, geometric, cohesive, directed…
models: topological, simplicial, localic, …
see also algebraic topology
Introductions
Definitions
Paths and cylinders
Homotopy groups
Basic facts
Theorems
Cohomology
Special and general types
-
group cohomology, nonabelian group cohomology, Lie group cohomology
-
-
cohomology with constant coefficients / with a local system of coefficients
Special notions
Variants
-
differential cohomology
Extra structure
Operations
Theorems
Manifolds and cobordisms
manifolds and cobordisms
cobordism theory, Introduction
Definitions
Genera and invariants
Classification
Theorems
Spheres
Contents
Idea
Twisted cohomotopy is the twisted cohomology-variant of the the non-abelian cohomology-theory cohomotopy, represented by homotopy types of n-spheres.
The coefficients/twist for twisted cohomotopy are spherical fibrations, and cocycles are sections of these. For those spherical fibrations arising as unit sphere bundles of real vector bundles the twist may be understood as given by the J-homomorphism.
Properties
Various classical theorems of differential topology are secretly theorems about twisted cohomotopy
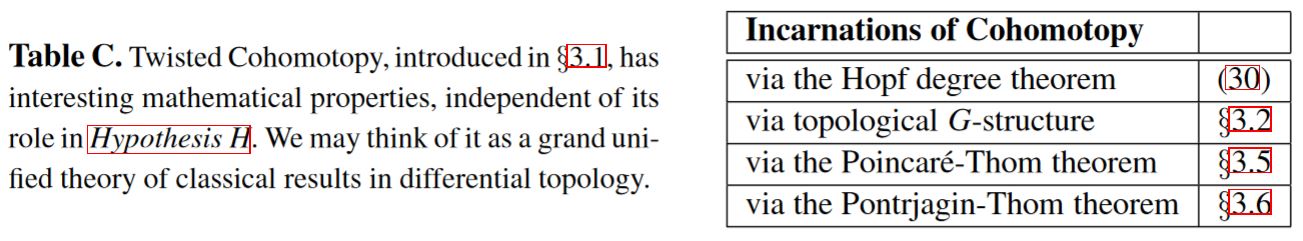
table grabbed from FSS 19b
Twisted Pontrjagin theorem
The twisted Pontrjagin theorem relates twisted cohomotopy with cobordism classes of normally twisted-framed submanifolds.
Twisted May-Segal theorem
The May-Segal theorem has a twisted generalization:
Proposition
Let
-
be a smooth closed manifold of dimension ;
Then the scanning map constitutes a weak homotopy equivalence
between
-
the J-twisted (n+k)-cohomotopy space of , hence the space of sections of the -spherical fibration over which is associated via the tangent bundle by the O(n)-action on
-
the configuration space of points on with labels in .
(Bödigheimer 87, Prop. 2, following McDuff 75)
Remark
In the special case that the closed manifold in Prop. is parallelizable, hence that its tangent bundle is trivializable, the statement of Prop. reduces to this:
Let
-
be a parallelizable closed manifold of dimension ;
Then the scanning map constitutes a weak homotopy equivalence
between
-
-cohomotopy space of , hence the space of maps from to the (n+k)-sphere
-
the configuration space of points on with labels in .
Poincaré–Hopf theorem
See at
Equivariant Hopf degree theorem
On flat orbifolds, twisted cohomotopy becomes equivariant cohomotopy and the twisted Hopf degree theorem becomes the
Related concepts
| flavours of Cohomotopy cohomology theory | cohomology (full or rational) | equivariant cohomology (full or rational) |
|---|---|---|
| non-abelian cohomology | Cohomotopy (full or rational) | equivariant Cohomotopy |
| twisted cohomology (full or rational) | twisted Cohomotopy | twisted equivariant Cohomotopy |
| stable cohomology (full or rational) | stable Cohomotopy | equivariant stable Cohomotopy |
| differential cohomology | differential Cohomotopy | equivariant differential cohomotopy |
| persistent cohomology | persistent Cohomotopy | persistent equivariant Cohomotopy |
References
The concept is implicit in classical texts on differential topology, for instance
-
Dusa McDuff, Configuration spaces of positive and negative particles, Topology Volume 14, Issue 1, March 1975, Pages 91-107 (doi:10.1016/0040-9383(75)90038-5)
-
Carl-Friedrich Bödigheimer, Section 2 of: Stable splittings of mapping spaces, Algebraic topology. Springer 1987. 174-187 (pdf, pdf)
Discussion of the twisted Pontrjagin theorem and of twisted stable cohomotopy (framed cobordism cohomology theory):
- James Cruickshank, around Lemma 5.2 of: Twisted homotopy theory and the geometric equivariant 1-stem, Topology and its Applications 129 3 (2003) 251-271 [doi:10.1016/S0166-8641(02)00183-9]
Discussion of unstable twisted Cohomotopy, with application to flux quantization of supergravity C-field (“Hypothesis H”):
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber, Twisted Cohomotopy implies M-theory anomaly cancellation on 8-manifolds, Comm Math. Phys. 377 (2020) 1961-2025 [arXiv:1904.10207, doi:10.1007/s00220-020-03707-2]
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber, Twisted Cohomotopy implies level quantization of the full 6d Wess-Zumino-term of the M5-brane (arXiv:1906.07417)
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber, Twisted Cohomotopy implies M5-brane anomaly cancellation (arXiv:2002.07737)
-
Domenico Fiorenza, Hisham Sati, Urs Schreiber, Twisted Cohomotopy implies twisted String structure on M5-branes (arXiv:2002.11093)
Last revised on June 23, 2025 at 09:50:14. See the history of this page for a list of all contributions to it.