nLab Kontsevich integral
Context
Knot theory
Examples/classes:
Types
Related concepts:
Integration theory
Analytic integration
Riemann integration, Lebesgue integration
line integral/contour integration
integration of differential forms
integration over supermanifolds, Berezin integral, fermionic path integral
Kontsevich integral, Selberg integral, elliptic Selberg integral
Cohomological integration
integration in ordinary differential cohomology
integration in differential K-theory
Variants
Topology
topology (point-set topology, point-free topology)
see also differential topology, algebraic topology, functional analysis and topological homotopy theory
Basic concepts
-
fiber space, space attachment
Extra stuff, structure, properties
-
Kolmogorov space, Hausdorff space, regular space, normal space
-
sequentially compact, countably compact, locally compact, sigma-compact, paracompact, countably paracompact, strongly compact
Examples
Basic statements
-
closed subspaces of compact Hausdorff spaces are equivalently compact subspaces
-
open subspaces of compact Hausdorff spaces are locally compact
-
compact spaces equivalently have converging subnet of every net
-
continuous metric space valued function on compact metric space is uniformly continuous
-
paracompact Hausdorff spaces equivalently admit subordinate partitions of unity
-
injective proper maps to locally compact spaces are equivalently the closed embeddings
-
locally compact and second-countable spaces are sigma-compact
Theorems
Analysis Theorems
The Kontsevich Integral
Idea
The Kontsevich integral is the Dyson formula for the parallel transport or holonomy of the Knizhnik-Zamolodchikov connection on ordered configuration spaces of points in the plane. As such it is a (universal) Vassiliev invariant of braids and, with due care, of knots and links, essentially coinciding with the Wilson loop observable of perturbatively quantized Chern-Simons theory.
The Kontsevich integral generalises the Gauss integral formula? which computes the linking number of two embedded circles via integration.
Definition
On braids
For the Definition of the Knizhnik-Zamolodchikov connection we need the following notation:
-
configuration spaces of points
For write
(1)for the ordered configuration space of n points in the plane, regarded as a smooth manifold.
Identifying the plane with the complex plane , we have canonical holomorphic coordinate functions
(2) -
(3)
for the quotient vector space of the linear span of horizontal chord diagrams on strands by the 4T relations (infinitesimal braid relations), regarded as an associative algebra under concatenation of strands (here).
Definition
The universal Knizhnik-Zamolodchikov form is the horizontal chord diagram-algebra valued differential form (3) on the configuration space of points (1)
given in the canonical coordinates (2) by:
where
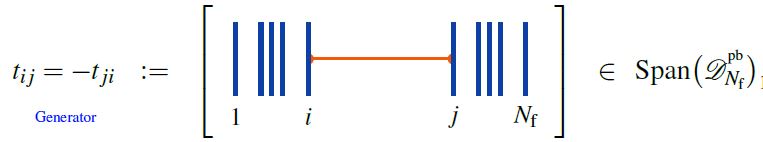
is the horizontal chord diagram with exactly one chord, which stretches between the th and the th strand.
Regarded as a connection form for a connection on a vector bundle, this defines the universal Knizhnik-Zamolodchikov connection , with covariant derivative
for any smooth function
with values in modules over the algebra of horizontal chord diagrams modulo 4T relations.
The condition of covariant constancy
is called the Knizhnik-Zamolodchikov equation.
Finally, given a metric Lie algebra and a tuple of Lie algebra representations
the corresponding endomorphism-valued Lie algebra weight system
turns the universal Knizhnik-Zamolodchikov form (4) into a endomorphism ring-valued differential form
The universal formulation (4) is highlighted for instance in Bat-Natan 95, Section 4.2, Lescop 00, p. 7. Most authors state the version after evaluation in a Lie algebra weight system, e.g. Kohno 14, Section 5.
Proposition
(Knizhnik-Zamolodchikov connection is flat)
The Knizhnik-Zamolodchikov connection (Def. ) is flat:
Proposition
(Kontsevich integral for braids)
The Dyson formula for the holonomy of the Knizhnik-Zamolodchikov connection (Def. ) is called the Kontsevich integral on braids.
(e.g. Lescop 00, side-remark 1.14)
On knots
Definition
Let be a strict Morse knot?. Let be the graded completion? of the algebra of chord diagrams with -term relations. The Kontsevich integral of is given by:
In this definition:
- and are the minimum and maximum of the -coordinate in the Morse knot? .
- The integration is over the points in the simplex of points in the interval where no coordinate is critical on .
- Upon removing the critical values (note: values not points, so we remove a point if it is on the same level as a critical point), the knot decomposes into a set of arcs which can be parametrised by height. Each arc therefore defines a function where is the corresponding interval of height values. In fact, must be the open interval between two successive critical values of the height function. For a particular such interval, there must be an even number of arcs with that domain. Given a point in the simplex (with no critical values), each coordinate in that point lies in an interval between critical values, and then for that interval we choose an unordered pair of arcs. A choice of pair for each coordinate is called a pairing, and is written .
- For a pairing, , the symbol denotes the number of arcs that are oriented downwards when equipped with the inherited orientation from .
- Putting the knot back together as a circle, we join the ends of the pairing to make a chord diagram with chords. This defines an element in the algebra of chord diagrams which we denote by .
Invariance
The Kontsevich integral is an invariant of Morse knots? but is not quite a knot invariant. When a “hump” is introduced to the knot then it is multiplied by where is the “humped” unknot. Therefore, it can be made in to a genuine knot invariant via the formula
where is the number of critical points of . To distinguish this from the Kontsevich integral, it is sometimes called the final Kontsevich integral (and the other the preliminary one).
Related concepts
References
General
Review:
-
Christine Lescop, Introduction to the Kontsevich Integral of Framed Tangles, 2000 (pdf)
-
Toshitake Kohno, Section 5 of: Local Systems on Configuration Spaces, KZ Connections and Conformal Blocks, Acta Math Vietnam 39, 575–598 (2014) (doI;10.1007/s40306-014-0088-6, pdf)
Textbook account:
- Christine Lescop, Invariants of links and 3-manifolds from graph configurations (arXiv:2001.09929)
Perturbative quantum Chern-Simons theory
Discussion of perturbative quantization of Chern-Simons theory (via Kontsevich integrals/knot graph cohomology on Jacobi diagrams, regarding Feynman amplitudes as differential forms on configuration spaces of points and yielding universalVassiliev invariants):
-
Dror Bar-Natan, Perturbative aspects of the Chern-Simons topological quantum field theory, thesis 1991 (spire:323500, proquest:303979053, BarNatanPerturbativeCS91.pdf)
-
Scott Axelrod, Isadore Singer, Chern-Simons Perturbation Theory, in S. Catto, A. Rocha (eds.) Proc. XXthe DGM Conf. World Scientific Singapore, 1992, 3-45; (arXiv:hep-th/9110056)
-
Scott Axelrod, Isadore Singer, Chern–Simons Perturbation Theory II, J. Diff. Geom. 39 (1994) 173-213 (arXiv:hep-th/9304087)
-
Maxim Kontsevich, Vassiliev’s knot invariants, Advances in Soviet Mathematics, Volume 16, Part 2, 1993 (pdf)
-
Maxim Kontsevich, Feynman diagrams and low-dimensional topology, in First European Congress of Mathematics, Vol. II (Paris, 1992), volume 120 of Progr. Math., pages 97–121, Birkhäuser, Basel, 1994. (pdf)
-
Dror Bar-Natan, Perturbative Chern-Simons theory, Journal of Knot Theory and Its RamificationsVol. 04, No. 04, pp. 503-547 (1995) (doi:10.1142/S0218216595000247)
-
Daniel Altschuler, Laurent Freidel, Vassiliev knot invariants and Chern-Simons perturbation theory to all orders, Commun. Math. Phys. 187 (1997) 261-287 arXiv:q-alg/9603010, doi:10.1007/s002200050136
-
Pascal Lambrechts, Ismar Volić, sections 6 and 7 of Formality of the little N-disks operad, Memoirs of the American Mathematical Society ; no. 1079, 2014 (arXiv:0808.0457, doi:10.1090/memo/1079)
Review:
-
Robbert Dijkgraaf, Perturbative topological field theory, In: Trieste 1993, Proceedings, String theory, gauge theory and quantum gravity ‘93 189-227 (spire:399223, pdf)
-
Sergei Chmutov, Sergei Duzhin, Jacob Mostovoy, Section 4 of: Introduction to Vassiliev knot invariants, Cambridge University Press, 2012 (arxiv/1103.5628, doi:10.1017/CBO9781139107846)
See also at correlator as differential form on configuration space of points and see at graph complex as a model for the spaces of knots.
The “Wheels theorem”, saying that the perturbative Chern-Simons Wilson loop observable of the unknot is, as a universal Vassiliev invariant, a series of wheel-shaped Jacobi diagrams with coefficients the modified Bernoulli numbers, is due to
- Dror Bar-Natan, Thang T Q Le, Dylan Thurston, Two applications of elementary knot theory to Lie algebras and Vassiliev invariants, Geom. Topol. Volume 7, Number 1 (2003), 1-31 (euclid.gt/1513883092)
following
- Dror Bar-Natan, Stavros Garoufalidis, Lev Rozansky, Dylan Thurston, Wheels, wheeling, and the Kontsevich integral of the unknot (q-alg/9703025)
Last revised on January 28, 2020 at 02:59:40. See the history of this page for a list of all contributions to it.