nLab M-theory lift of gauge enhancement on D6-branes
Context
String theory
Ingredients
Critical string models
Extended objects
Topological strings
Backgrounds
Phenomenology
Contents
Idea
It is well understood that in type IIA string theory there appears
-
enhanced gauge symmetry on -coincident D-branes;
-
enhanced gauge symmetry on -coincident D-branes at an orientifold plane.
The physical picture of this effect is that the gauge bosons of these gauge fields are the modes of the open strings stretching between these D-branes which become massless as the branes coincide.
Now, as the situation is lifted to M-theory, the D0-branes, D2-branes and D4-branes lift to M2-branes and M5-branes, and the gauge enhancement is thought to be similarly reflected on these M-branes (as exhibited for the M2-branes by the BLG-model and ABJM-model).
But for the D6-brane the situation is different: the D6-brane lifts not to an M-brane, but to a configuration of the field of 11-dimensional supergravity: the 11d Kaluza-Klein monopole.
Here we discuss the picture of how gauge enhancement on D6-branes in type IIA string theory is reflected on Kaluza-Klein monopoles at ADE-singularities. The explicit realization reviewed below is due to (Sen 97).
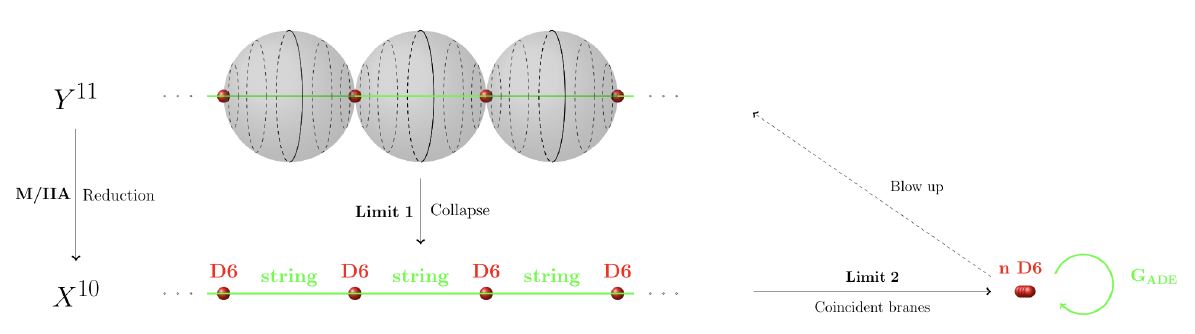
graphics grabbed from HSS18
Construction
-singularity and -gauge enhancement
The 11d multi-centered Kaluza-Klein monopole spacetime has metric tensor of the form
where
is the metric tensor of the multi-centered Taub-NUT space, with the canonical coordinate on a circle and with
and
for the set of positions of the KK-monopoles of mass .
Notice that the radius of the -circle vanishes precisely at the positions . Nevertheless, as long as all the are distinct, then the above is a smooth spacetime also at the positions of the – if the periodicity of is taken to be . But with this periodicity fixed, then as the monopole positions coincide, then the resulting metric has a conical singularity at that point, of ADE-singularity type .
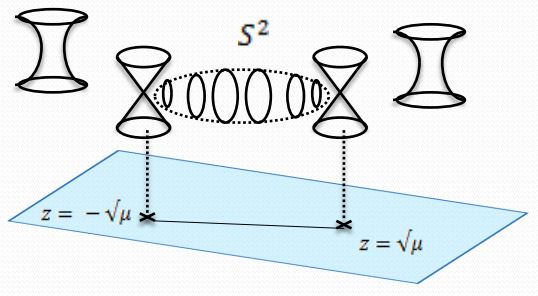
To see where the gauge enhancement arises from in this singular case, observe that in the non-singular configuration there are linearly indepent 2-cycles in the multi-center KK-monopole spacetime, represented by the the 2-spheres that are swept out by the circle fiber as it moves from to (remembering that the circle fiber radius vanishes precisely at the positions ).
For the canonical choice of straight path between and (and arbitrary fixed position in the remaining 7 dimensions) then the surface area of these 2-spheres is
For any other choice of path the surface area will be larger. Hence an M2-brane with tension swrapping the 2-cycle has minimal tension energy when in the configuration of these spheres, namely
The type IIA limit is given by . In this limit the M2-branes wrapping the above cycles become the type IIA superstring by double dimensional reduction, the KK-monopoles become the D6-branes, and it is evident from the geometry that the membrane warpping becomes an open string strentching between the th and the th D6-brane.
In the limit the D6-branes coincide,the strings stretching between them become massless (in accord with the above formula for the wrapped M2-brane mass), and become the gauge bosons of an Chan-Paton gauge field.
-singularity and -gauge enhancement
Now consider the above setupmodified by replacing the Taub-NUT space with coordinates by its -orbifold given by the -action with nontrivial operation given by
and in the Taub-NUT metric replace by
The type IIA image of the origin of this configuration is an orientifold plane.
Now as the all approach 0 we get an 11d spacetime with a -type ADE-singularity, whose type IIA image is D6-branes coincident on an orientifold plane.
Related concepts
from M-branes to F-branes: superstrings, D-branes and NS5-branes
(e.g. Johnson 97, Blumenhagen 10)
Refrences
- Ashoke Sen, A Note on Enhanced Gauge Symmetries in M- and String Theory, JHEP 9709:001,1997 (arXiv:hep-th/9707123)
Last revised on September 9, 2019 at 19:35:03. See the history of this page for a list of all contributions to it.