nLab Poincaré–Hopf theorem

Idea

The Poincaré–Hopf theorem says that for any vector field $v \in \Gamma(T X)$ with a finite set of isolated vanishing points $\{x_i\}$ on an orientable compact differential manifold $X$, the sum over the $x_i \in X$ of the degrees of the vector in the vicinity of these points, regarded as cohomotopy classes

$$v/{\vert v\vert}_{\vert x_i} \;\colon\; \partial D_{x_i} \longrightarrow S(T_{x_i} X)$$

and called the Poincaré–Hopf index of $f$ at $x_i$

$$ind_{x_i}(v) \;\coloneqq\; deg\big( v/{\vert v\vert}_{\vert {x_i} } \big)$$

is given by the Euler characteristic, hence by the value of the Euler class on the tangent bundle:

$$\chi(X) \coloneqq \chi[T X] \;=\; \underset{ {\text{isolated zero}} \atop { x_i } }{\sum} ind_{x_i}(v) \,.$$

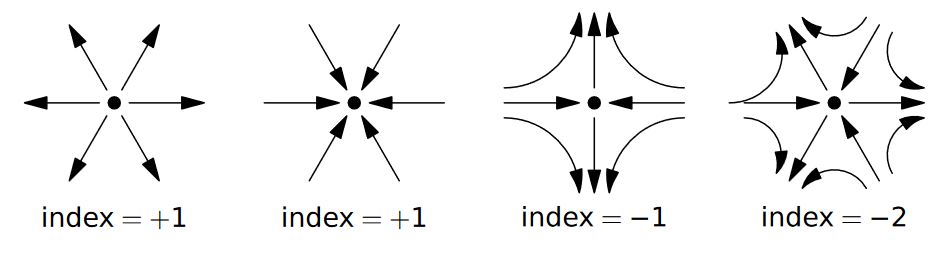
In particular, the existence of a nowhere vanishing vector field (for which the above sum is empty) implies that the Euler characteristic vanishes.

The converse also holds: If the Euler characteristic of a closed manifold vanishes, then a nowhere vanishing vector field exists (e.g. MO:a/47553/58526).

Also: Given any connected closed manifold, the complement of any positive number of disjoint open balls carries a nowhere vanishing vector field (namely: choose any vector field with isolated zeros on the original manifold and then deform continuously to push all these zeros into inside the open balls). Smoothly scaling this vector field to zero towards the centers of these open balls gives a vector field on the original manifold which may be used in the PH-theorem.

Related concepts

• Hopf degree theorem, equivariant Hopf degree theorem

• Pontrjagin theorem, equivariant Pontrjagin theorem

• Gauss-Bonnet theorem

• twisted cohomotopy

References

Named after Henri Poincaré and Heinz Hopf.

Textbook accounts:

• Raoul Bott, Loring Tu, Chapter 11 of Differential Forms in Algebraic Topology, Graduate Texts in Mathematics 82, Springer 1982 (doi:10.1007/BFb0063500)

• B. A. Dubrovin, S. P. Novikov, A. T. Fomenko, section 15.2 of Modern Geometry — Methods and Applications: Part II: The Geometry and Topology of Manifolds, Graduate Texts in Mathematics 104, Springer-Verlag New York, 1985 (doi:10.1007/978-1-4612-1100-6)

• John Milnor, Chapter 6 of: Topology from the differential viewpoint, Princeton University Press, 1997. (ISBN:9780691048338, pdf)

• Gerard Walschap, chapter 6.7 of Metric Structures in Differential Geometry, Graduate Texts in Mathematics, Springer 2004

Review:

• Alex Wright, Kael Dixon, The Poincaré–Hopf theorem (pdf)

• Ariel Hafftka, Differential topology and the Poincaré–Hopf theorem (pdf)

See also:

• Wikipedia, Poincaré–Hopf theorem

Discussion in a broader perspective of K-theory and index theorems:

• Omar Mohsen, Poincaré–Hopf theorem and groupoids (pdf, pdf)

A comment on the version for complex vector fields is in

• Howard Jacobowitz, Non-vanishing complex vector fields and the Euler characteristic (arXiv:0901.0893)

Generalization to orbifolds:

• Christopher Seaton, Two Gauss–Bonnet and Poincaré–Hopf theorems for orbifolds with boundary, Differential Geometry and its Applications Volume 26, Issue 1, February 2008, Pages 42-51 (doi:10.1016/j.difgeo.2007.11.002)