nLab Kaluza-Klein monopole

Idea

General

In the context of Kaluza-Klein theory where an electromagnetic field in Einstein-Maxwell theory in dimension $d$ is modeled by a configuration of pure Einstein gravity in dimension $d+1$, a Kaluza-Klein monopole is a configuration of gravity in dimension $d+1$ which in dimension $d$ looks like a magnetic monopole (Sorkin 83, Gross-Perry 83).

In supergravity

This situation is of particular interest in the reduction of 11-dimensional supergravity (or M-theory, where one also speaks of the MK6-brane) where the Kaluza-Klein magnetic monopole charge is interpreted as D6-brane charge under duality between M-theory and type IIA string theory.

The Kaluza-Klein monopole (Han-Koh 85) is one type of solution of the equations of motion of 11-dimensional supergravity. It is given by the product $N_4\times \mathbb{R}^{11-5,1}$ of Euclidean Taub-NUT spacetime with Minkowski spacetime. Upon Kaluza-Klein compactification this looks like a monopole, whence the name. (For discussion as an ADE-singularity see IMSY 98, section 9, Asano 00, section 3.)

Upon KK-compactification on a 6-dimensional fiber, with the 11d KK-monopole / D6-brane completely wrapping the fiber, the KK-monopole in 11d supergravity becomes the KK-monopole in 5d supergravity. Further compactifying on a circle leads to a black hole in 4d, incarnated as a D0/D6 bound state (e.g. Nelson 93).

geometry transverse to KK-monopoles	Riemannian metric	remarks
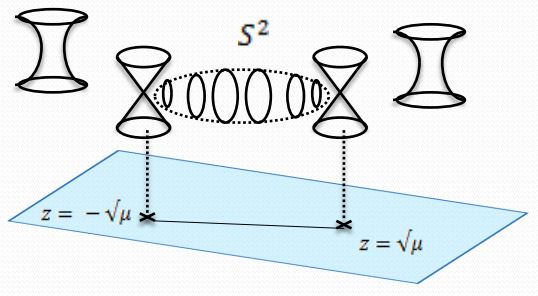
Taub-NUT space: geometry transverse to $N+1$ distinct KK-monopoles at $\vec r_i \in \mathbb{R}^3 \;\; i \in \{1, \cdots, N+1\}$	$\array{d s^2_{TaubNUT} \coloneqq U^{-1}(d x^4 + \vec \omega \cdot d \vec r)^2 + U (d \vec r)^2 \,, \\ \vec r \in \mathbb{R}^3,\, x^4 \in \mathbb{R}/(2 \pi R\mathbb{Z}) \\ U \coloneqq 1 + \underoverset{i = 1}{N+1}{\sum} U_i\,, \phantom{AA} \vec \omega \coloneqq \underoverset{i = 1}{N+1}{\sum} \vec \omega_i \\ U_i \coloneqq \frac{R/2}{ {\vert \vec r - \vec r_i\vert} }\,, \phantom{AA} \vec \nabla \times \vec \omega= \vec \nabla U_i}$	(e.g. Sen 97b, Sect. 2)
ALE space Taub-NUT close to $N$ close-by KK-monopoles e.g. close to $\vec r = 0$: $\frac{{\vert \vec r_i\vert}}{R/2}, \frac{{\vert \vec r\vert}}{R/2} \ll 1$	$\array{d s^2_{ALE} \coloneqq U'^{-1}(d x^4 + \vec \omega \cdot d \vec r)^2 + U' (d \vec r)^2 \,, \\ \vec r \in \mathbb{R}^3,\, x^4 \in \mathbb{R}/(2 \pi R\mathbb{Z}) \\ U' \coloneqq \underoverset{i = 1}{N+1}{\sum} U'_i\,, \phantom{AA} \vec \omega \coloneqq \underoverset{i = 1}{N+1}{\sum} \vec \omega_i \\ U'_i \coloneqq \frac{R/2}{ {\vert \vec r - \vec r_i\vert} }\,, \phantom{AA} \vec \nabla \times \vec \omega= \vec \nabla U_i}$	e.g. via Euler angles: $\vec \omega = (N+1)R/2(\cos(\theta)-1) d\psi$ (e.g. Asano 00, Sect. 2)
$A_N$-type ADE singularity: ALE space in the limit where all $N+1$ KK-monopoles coincide at $vec r_i = 0$	$\array{d s^2_{A_N Sing} \coloneqq \frac{\vert\vec r\vert }{(N+1)R/2}(d x^4 + \vec \omega \cdot d \vec r)^2 + \frac{ (N+1)R/2}{\vert \vec r\vert} (d \vec r)^2 \,, \\ \vec r \in \mathbb{R}^3,\, x^4 \in \mathbb{R}/(2 \pi R\mathbb{Z}) }$	(e.g. Asano 00, Sect. 3)

Properties

(For the moment the following is all about the KK-monopoles in 11d supergravity/M-theory.)

Far-horizon geometry

We discuss the far horizon geometry of coincident MK6-branes.

The metric tensor of $N$ coincident KK-monopoles in 11-dimensional supergravity in the limit that $\ell_{th} \coloneqq N \ell_P \to 0$ is

(1)$$g_{MK6} \;=\; g_{\mathbb{R}^{6,1}} + (d y)^2 + y^2 \big( (d \theta)^2 + (\sin \theta)^2 (d \varphi)^2 + (\cos \theta)^2 (d \phi)^2 \big)$$

subject to the identification

(2)$$(\varphi, \phi) \;\sim\; (\varphi, \phi) + (2\pi/N ,2\pi/N) \,.$$

This is equation (47) in IMSY 98, which applies subject to the condition

$$U/\left(\frac{N}{g^{2/3}_{YM}}\right) \;=\; U/\left(\frac{N}{(2\pi)^{4/3} \ell_P}\right) \;\gg\; 1$$

from a few lines above. Inserting this condition into the definition $y^2 \coloneqq 2 N \ell^3_P U$ right above (47) shows that

$$\begin{aligned} y^2 & = 2 N \ell^3_P U \\ & = 2(2\pi)^{-4/3} N^2 \ell_P^2 \; \underset{ \gg 1 }{ \underbrace{ \left(U/\left(\frac{N}{ (2 \pi)^{4/3} \ell_P}\right)\right) }} \end{aligned}$$

hence that the distance $y$ from the locus of the MK6-brane is large in units of

$$\ell_{th} \;=\; \sqrt{2} (2\pi)^{-2/3} N \ell_P \,.$$

The identification (2) means that this is the orbifold metric cone $\mathbb{R}^{6,1} \times \left( \mathbb{R}^4/(\mathbb{Z}_N)\right)$, hence an A-type ADE-singularity. To make this more explicit, introduce the complex coordinates

$$v \;\coloneqq\; y \, e^{i \varphi} \sin \theta \;\;\; w \;\coloneqq\; y \, e^{i \phi} \cos \theta$$

on $\mathbb{R}^4 \simeq \mathbb{C}^2$, in terms of which (1) becomes

$$g_{MK6} \;\coloneqq\; d v d \overline v + d w d \overline w$$

and which exhibit the identification (2) as indeed that of the A-type $\mathbb{Z}_N$-action (Asano 00, around (18)).

Relation to the D6-brane in type IIA string theory

Under the relation between M-theory and type IIA superstring theory an ADE orbifold of the 11d KK-monopole corresponds to D6-branes combined with O6-planes (Townsend 95, p. 6, Atiyah-Witten 01, p. 17-18 see also e.g. Berglund-Brandhuber 02, around p. 15).

By (Townsend 95, (1), Sen 97c (1)-(4)) the 11d spacetime describing the KK-monopole lift of a plain single D6 brane is $\mathbb{R}^{6,1}\times \mathbb{R}^3\times S^1$ with metric tensor away from the origin of the $\mathbb{R}^3$-factor (which is the locus of the lifted D6/monopole) being

$$d s_{11}^2 = - d t^2 + d s_{\mathbb{R}^6}^2 + (1+\mu/r) d s_{\mathbb{R}^3}^2 + (1+ \mu/r)^{-1} (d x^{11} - A_i d x^i)^2 \,,$$

where

• $A = A_i d x^i$ is any 3-form on $\mathbb{R}^3$ satisfying $d_{\mathbb{R}^3} A = \star d (1-\mu/r)$;

• $r$ denotes the distance in $\mathbb{R}^3$ from the origin.

• $\mu$ is the charge of the monopole.

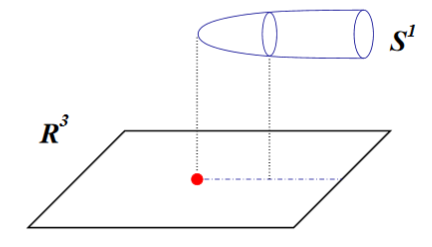
Away from $\{0\} \in \mathbb{R}^3$ this gives a circle bundle with first Chern class measured by the integral of $R_0 \coloneqq d A$ (the RR-field of the D0-brane) over any sphere surrounding the singular locus in $\mathbb{R}^3 - \{0\}$. By the electric-magnetic duality between D0-branes and D6-branes (due to the self-duality of the RR-field) this means, from the 10-dimensional perspective, that at $0 \in \mathbb{R}^3$ a D6-brane is located.

graphics grabbed from Acharya-Gukov 04

Notice that the circle bundle away from its degeneration locus as a bundle over $S^2 \hookrightarrow \mathbb{R}^3 -\{0\}$ is necessarily of the form $S^3 \to S^2$, a multiple of the complex Hopf fibration (see also Atiyah-Maldacena-Vafa 00, p. 10).

Discussion as an A-type ADE singularity is in (Sen 97c, section 2). Generalization to D-type singularities and hence D6-branes in orientifolds is in (Sen 97c ,section 3). Discussion as the fixed point of the circle group-action on the M-theory circle fibers is in (Townsend 95, p. 6, Atiyah-Witten 01, pages 17-18). Witten emphasizes that it is important that the location of the D6 is not just a cyclic group orbifold singularity but really a circle group-action fixed point conical singularity:

Chiral fermions arise when the locus of A−D−E singularities passes through isolated points at which X has an isolated conical singularity that is not just an orbifold singularity (Witten 01, p. 2).

Discussion dealing carefully with the perspective where the locus of the D6-brane is not remived: Gaillard & Schmude 09.

For more on this see at D6-brane – Relation to other branes and at M-theory lift of gauge enhancement on D6-branes.

Relation to the D7-brane in type IIB string theory/F-theory

Under further T-duality to type IIB superstring theory/F-theory these D6-branes become the D7-branes.

from M-branes to F-branes: superstrings, D-branes and NS5-branes

M-theory on $S^1_A \times S^1_B$-elliptic fibration	KK-compactification on $S^1_A$	type IIA string theory	T-dual KK-compactification on $S^1_B$	type IIB string theory	geometrize the axio-dilaton	F-theory on elliptically fibered-K3 fibration	duality between F-theory and heterotic string theory	heterotic string theory on elliptic fibration
M2-brane wrapping $S_A^1$	double dimensional reduction $\mapsto$	type IIA superstring	$\mapsto$	type IIB superstring	$\mapsto$	“	$\mapsto$	heterotic superstring
M2-brane wrapping $S_B^1$	$\mapsto$	D2-brane	$\mapsto$	D1-brane	$\mapsto$	“
M2-brane wrapping $p$ times around $S_A^1$ and $q$ times around $S_B^1$	$\mapsto$	$p$ strings and $q$ D2-branes	$\mapsto$	(p,q)-string	$\mapsto$	“
M5-brane wrapping $S_A^1$	double dimensional reduction $\mapsto$	D4-brane	$\mapsto$	D5-brane	$\mapsto$	“
M5-brane wrapping $S_B^1$	$\mapsto$	NS5-brane	$\mapsto$	NS5-brane	$\mapsto$	“	$\mapsto$	NS5-brane
M5-brane wrapping $p$ times around $S_A^1$ and $q$ times around $S_B^1$	$\mapsto$	$p$ D4-brane and $q$ NS5-branes	$\mapsto$	(p,q)5-brane	$\mapsto$	“
M5-brane wrapping $S_A^1 \times S_B^1$	$\mapsto$		$\mapsto$	D3-brane	$\mapsto$	“
KK-monopole/A-type ADE singularity (degeneration locus of $S^1_A$-circle fibration, Sen limit of $S^1_A \times S^1_B$ elliptic fibration)	$\mapsto$	D6-brane	$\mapsto$	D7-branes	$\mapsto$	A-type nodal curve cycle degeneration locus of elliptic fibration (Sen 97, section 2)		SU-gauge enhancement
KK-monopole orientifold/D-type ADE singularity	$\mapsto$	D6-brane with O6-planes	$\mapsto$	D7-branes with O7-planes	$\mapsto$	D-type nodal curve cycle degeneration locus of elliptic fibration (Sen 97, section 3)		SO-gauge enhancement
exceptional ADE-singularity	$\mapsto$		$\mapsto$		$\mapsto$	exceptional ADE-singularity of elliptic fibration	$\mapsto$	E6-, E7-, E8-gauge enhancement

(e.g. Johnson 97, Blumenhagen 10)

Other Brane charges (?)

Hull 1997 argued (around equation 2.12) that the KK-monopole in 11-dimensional supergravity is the object which carries the 6-form charge Poincaré dual to the time-component of the 5-form charge of the M5-brane as appearing in the M-theory super Lie algebra, via

$$\wedge^5 (\mathbb{R}^{10,1})^\ast \simeq \wedge^5 (\mathbb{R}^{10})^\ast \oplus \wedge^6 \mathbb{R}^{10} \,.$$

(An analogous relation identifies the time-component of the M2-brane charge with the charge of the M9-brane, see there.)

Related concepts

• M-brane

• Taub-NUT spacetime

• gravitational instanton

References

In 5d gravity

Original articles:

• Rafael Sorkin, Kaluza-Klein monopole, Phys. Rev. Lett. 51 (1983) 87 (doi:10.1103/PhysRevLett.51.87)

• David Gross, Malcolm Perry, Magnetic Monopoles in Kaluza-Klein Theories, Nucl. Phys. B226 (1983) 29 (doi:10.1016/0550-3213(83)90462-5)

• P. J. Ruback, The motion of Kaluza-Klein monopoles, Comm. Math. Phys. Volume 107, Number 1 (1986), 93-102 (euclid:1104115933)

• A. L. Cavalcanti de Oliveira, E. R. Bezerra de Mello, Kaluza-Klein Magnetic Monopole in Five-Dimensional Global Monopole Spacetime, Class. Quant. Grav. 21 (2004) 1685-1694 (arXiv:hep-th/0309189)

Review:

• Emre Sakarya, Kaluza-Klein monopole (2007) [pdf]

• Tomás Ortín: §15 in: Gravity and Strings, Cambridge University Press (2010, 2015) [doi:10.1017/CBO9780511616563, doi:10.1017/CBO9781139019750]

Discussion of topological T-duality for KK-monopoles is in

• Ashwin S. Pande, Topological T-duality and Kaluza-Klein Monopoles, Adv. Theor. Math. Phys., 12, (2007), pp 185-215 (arXiv:math-ph/0612034)

In 11d supergravity

Original articles

• Seung Kee Han, I. G. Koh: $N = 4$ Remaining Supersymmetry in Kaluza-Klein Monopole Background in $D=11$ Supergravity Theory, Phys. Rev. D 31 (1985) 2503 [doi:10.1103/PhysRevD.31.2503, spire:204521]

  also in: Michael Duff (ed.): The World in Eleven Dimensions 57–60

• Paul Townsend, The eleven-dimensional supermembrane revisited, Phys. Lett. B 350 (1995) 184-187 [arXiv:hep-th/9501068]

• Chris Hull: Gravitational Duality, Branes and Charges, Nucl. Phys. B 509 (1998) 216-251 [arXiv:hep-th/9705162, doi:10.1016/S0550-3213(97)00501-4]

• Ashoke Sen, Kaluza-Klein Dyons in String Theory, Phys. Rev. Lett. 79:1619-1621, 1997 (arXiv:hep-th/9705212)

• Ashoke Sen, Dynamics of Multiple Kaluza-Klein Monopoles in M- and String Theory, Adv. Theor. Math. Phys. 1:115-126, 1998 (arXiv:hep-th/9707042)

• Ashoke Sen, A Note on Enhanced Gauge Symmetries in M- and String Theory, JHEP 9709:001,1997 (arXiv:hep-th/9707123)

• Nissan Itzhaki, Juan Maldacena, Jacob Sonnenschein, Shimon Yankielowicz, Supergravity and The Large $N$ Limit of Theories With Sixteen Supercharges, Phys. Rev. D 58, 046004 1998 (arXiv:hep-th/9802042)

• Masako Asano, Compactification and Identification of Branes in the Kaluza-Klein monopole backgrounds (arXiv:hep-th/0003241)

• Michael Atiyah, Juan Maldacena, Cumrun Vafa, An M-theory Flop as a Large N Duality, J.Math.Phys.42:3209-3220,2001 (arXiv:hep-th/0011256)

• Michael Atiyah, Edward Witten $M$-Theory dynamics on a manifold of $G_2$-holonomy, Adv. Theor. Math. Phys. 6 (2001) (arXiv:hep-th/0107177)

• Edward Witten, Anomaly Cancellation On Manifolds Of $G_2$ Holonomy (arXiv:hep-th/0108165)

• Per Berglund, Andreas Brandhuber, Matter From $G_2$ Manifolds, Nucl.Phys. B641 (2002) 351-375 (arXivLhep-th/0205184)

• Jérôme Gaillard, Johannes Schmude, The lift of type IIA supergravity with D6 sources: M-theory with torsion, JHEP 1002:032,2010 (arXiv:0908.0305)

Discussion for the massive case, reducing to the D6-brane in massive type IIA string theory:

• Eric Bergshoeff, Eduardo Eyras, Yolanda Lozano, The massive Kaluza-Klein monopole, Physics Letters B

  430 1–2 (1998) 77-86 [doi:10.1016/S0370-2693(98)00501-2, arXiv:hep-th/9802199]

Discussion in terms of G-structures:

• Ulf Danielsson, Giuseppe Dibitetto, Adolfo Guarino, KK-monopoles and $G$-structures in M-theory/type IIA reductions, JHEP 1502 (2015) 096 (arXiv:1411.0575)

Discussion of a BFSS-like matrix model for MK6-branes:

• Amihay Hanany, Gilad Lifschytz, M(atrix) Theory on $T^6$ and a m(atrix) Theory Description of KK Monopoles, Nucl. Phys. B519:195-213, 1998 (arXiv:hep-th/9708037)

Relation to massive type IIA string theory:

• Eduardo Eyras, Yolanda Lozano, The Kaluza-Klein Monopole in a Massive IIA Background, Nucl. Phys. B546 (1999) 197-218 [arXiv:hep-th/9812188, doi:10.1016/S0550-3213(99)00098-X]

Review

• Clifford Johnson, section 10.5 of D-brane primer (arXiv:hep-th/0007170)

• Katrin Becker, Melanie Becker, John Schwarz, p. 333 of String Theory and M-Theory: A Modern Introduction, 2007

• Luis Ibáñez, Angel Uranga, section 6.3.3 of String Theory and Particle Physics – An Introduction to String Phenomenology, Cambridge University Press 2012

• Bobby Acharya, Sergei Gukov, p. 45 of: M theory and Singularities of Exceptional Holonomy Manifolds, Phys. Rept. 392 (2004) 121-189 [arXiv:hep-th/0409191]

Relation to black holes

Relation to black holes in string theory

• William Nelson, Kaluza-Klein Black Holes in String Theory, Phys. Rev. D49:5302-5306, 1994 (arXiv:hep-th/9312058)