nLab double dimensional reduction
Context
String theory
Ingredients
Critical string models
Extended objects
Topological strings
Backgrounds
Phenomenology
Contents
Idea
What is called double dimensional reduction is a variant of Kaluza-Klein mechanism combined with fiber integration in the presence of branes: given a spacetime of dimension in which a -brane propagates, its KK-reduction results in a -dimensional effective spacetime containing a -brane together with a “doubly reduced” -brane, which is the reduction of those original -brane configurations that wrapped the cycle along which the KK-reduction takes place.
Definition
Via fiber integration in ordinary differential cohomology
Let be the smooth topos. For write for the universal moduli stack of circle n-bundles with connection (given by the Deligne complex).
Notice that fiber integration in ordinary differential cohomology has the following stacky incarnation (see here):
Proposition
For an oriented closed manifold of dimension , then fiber integration in ordinary differential cohomology is reflected by a morphism of the form
where the vertical morphisms are the curvature maps and the bottom morphisms reflects ordinary fiber integration of differential forms.
Definition
Given a cocycle
on the Cartesian product of some smooth space with , then its double dimensional reduction is the cocycle on which is given by the composite
where the first morphism is the unit of the (Cartesian product internal hom)-adjunction.
Via cyclic loop spaces
We discuss here a formalization of double dimensional reduction via cyclification adjunction (FSS 16, section 3, BMSS 18, section 2.2). For more see at geometry of physics – fundamental super p-branes the section on double dimensional reduction.
Proposition
Let be any (∞,1)-topos and let be an ∞-group in . Then the right base change/dependent product along the canonical point inclusion into the delooping of takes the following form: There is a pair of adjoint ∞-functors of the form
where
-
denotes the internal hom in ,
-
denotes the homotopy quotient by the conjugation ∞-action for equipped with its canonical ∞-action by left multiplication and the argument regarded as equipped with its trivial --action (for the circle group this is the cyclic loop space construction).
Hence for
-
a coefficient object, such as for some differential generalized cohomology theory
then there is a natural equivalence
given by
Proof
First observe that the conjugation action on is the internal hom in the (∞,1)-category of -∞-actions . Under the equivalence of (∞,1)-categories
(from NSS 12) then with its canonical ∞-action is and with the trivial action is .
Hence
Actually, this is the very definition of what is to mean in the first place, abstractly.
But now since the slice (∞,1)-topos is itself cartesian closed, via
it is immediate that there is the following sequence of natural equivalences
Here denotes the terminal morphism and denotes the base change along it.
Examples
Reduction on the circle
Example
When is the circle, and we think of as a spacetime of 11-dimensional supergravity, then may represent the supergravity C-field as a cocycle in ordinary differential cohomology. Then its double dimensional reduction in the sense of def. is the differential cocycle representing the B-field on , in the sense of string theory.
Remark
For a circle as in example , then the morphism in def. sends each point of to the loop in that winds identically around the copy of at that point. Hence in this case it would make sense to consider, more generally, for each the “order ” double dimensional reduction, given by the operation where one instead considers the map that lets the loop wind times around the .
The resulting double dimensional reduction is just -times the original one, so in a sense nothing much is changed, but maybe it is suggestive that now we are looking at the space of -fixed points of the free loop space (for the cyclic group of order ). In E-infinity geometry this fixed-point structure on the free loop spaces makes the derived function algebras – the topological Hochschild homology of the original function algebras – be cyclotomic spectra.
For general super -branes
Double dimensional reduction for the super--branes in dimensions which are described by the Green-Schwarz action functional corresponds to moving down and left the diagonals in the brane scan table of consistent such branes:
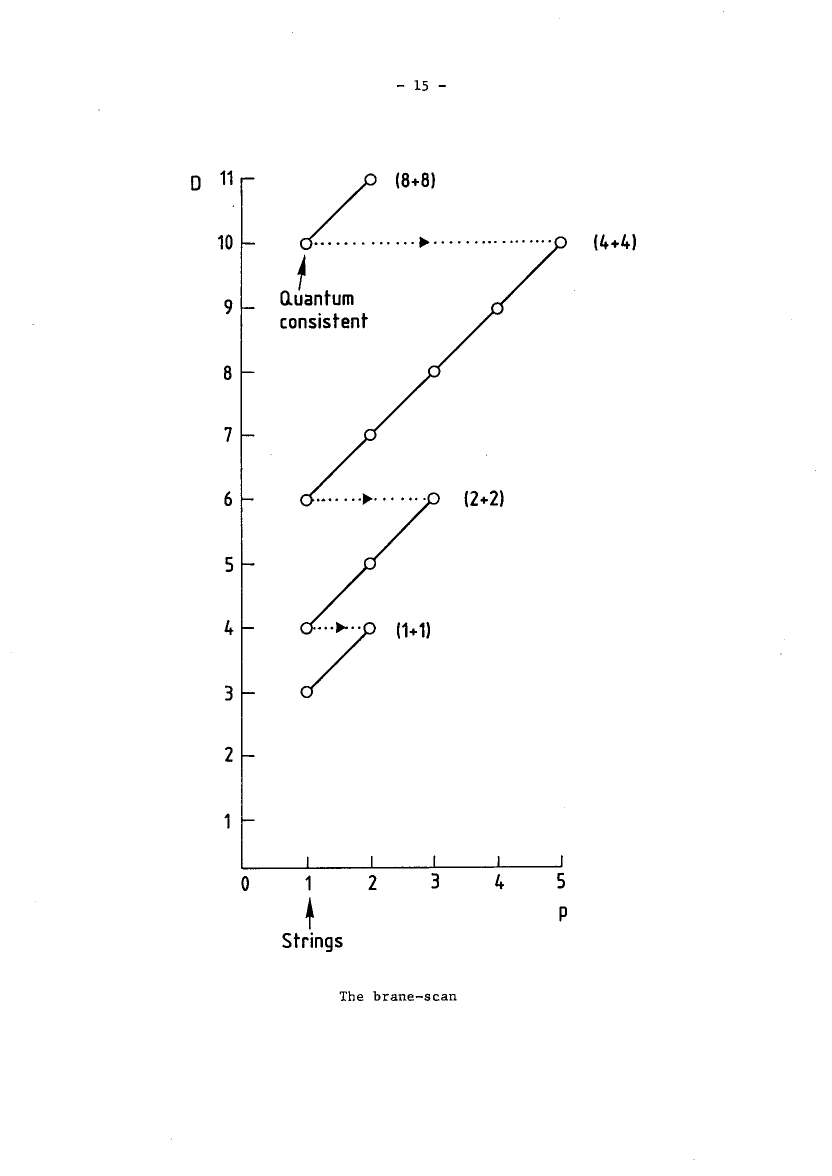
In particular
-
the superstring of type IIA string theory appears as the double dimensional reduction of the M2-brane in the KK-compactification from 11-dimensional supergravity/M-theory down to 10-dimensional type II supergravity/type II string theory.
-
the D4-brane appears as the double dimensional reduction of the M5-brane under this process;
-
the double dimensional reduction of the super 2-brane in 4d is super 1-brane in 3d (see there).
From M-branes to F-branes
from M-branes to F-branes: superstrings, D-branes and NS5-branes
(e.g. Johnson 97, Blumenhagen 10)
Related concepts
References
Formalization of double dimensional reduction is discussed in rational homotopy theory in
- Domenico Fiorenza, Hisham Sati, Urs Schreiber, Section 3 of T-Duality from super Lie n-algebra cocycles for super p-branes, ATMP Volume 22 (2018) Number 5 (arXiv:1611.06536)
and in full homotopy theory in
-
Vincent Braunack-Mayer, Hisham Sati, Urs Schreiber, Section 2.2 of: Gauge enhancement of Super M-Branes via rational parameterized stable homotopy theory, Communications in Mathematical Physics 371: 197 (2019) (arXiv:1806.01115, doi:10.1007/s00220-019-03441-4)
-
Hisham Sati, Urs Schreiber: Cyclification of Orbifolds, Comm. Math. Phys. 405 67 (2024) [doi:10.1007/s00220-023-04929-w, arXiv:2212.13836, talk]
Exposition is in
- Urs Schreiber, Section 4 of Super Lie n-algebra of Super p-branes, talks at Fields, Strings, and Geometry Seminar, Surrey, 5th-9th Dec. 2016
and further discussion in
-
Luigi Alfonsi, Global Double Field Theory is Higher Kaluza-Klein Theory, Fortsch. d. Phys. 68 3-4 (2020) [arXiv:1912.07089, doi:10.1002/prop.202000010]
-
Luigi Alfonsi: The puzzle of global Double Field Theory: open problems and the case for a Higher Kaluza-Klein perspective, Fortschr. Phys. 69 7 (2021) [arXiv:2007.04969, doi:10.1002/prop.202000102]
Reduction of membrane to string
The concept of double dimensional reduction was introduced, for the case of the reduction of the supermembrane in 11d to the Green-Schwarz superstring in 10d, in
- Michael Duff, Paul Howe, T. Inami, Kellogg Stelle, Superstrings in from Supermembranes in , Phys. Lett. B191 (1987) 70 and in Michael Duff (ed.) The World in Eleven Dimensions 205-206 (1987) [spire:245249, doi:10.1016/0370-2693(87)91323-2]
The above “brane scan” table showing the double dimensional reduction pattern of the super--branes given by the Green-Schwarz action functional (see there for more references on this) is taken from
- Michael Duff: Supermembranes: the first fifteen weeks, Class. Quantum Grav. 5 189 (1988) [doi:10.1088/0264-9381/5/1/023]
Reduction of M5-brane to D4-brane
The double dimensional reduction of the M5-brane to the D4-brane:
-
Paul Townsend, D-branes from M-branes, Phys. Lett. B373 (1996) 68-75 (arXiv:hep-th/9512062)
-
Mina Aganagic, Jaemo Park, Costin Popescu, John Schwarz, Section 6 of: World-Volume Action of the M Theory Five-Brane, Nucl. Phys. B 496 (1997) 191-214 [arXiv:hep-th/9701166, doi:10.1016/S0550-3213(97)00227-7]
-
Mina Aganagic, Jaemo Park, Costin Popescu, John Schwarz, Section 6 of: Dual D-Brane Actions, Nucl. Phys. B 496 (1997) 215-230 [arXiv:hep-th/9702133, doi:10.1016/S0550-3213(97)00257-5]
-
Neil Lambert, Constantinos Papageorgakis, Maximilian Schmidt-Sommerfeld, M5-Branes, D4-Branes and Quantum 5D super-Yang-Mills, JHEP 1101:083 (2011) (arXiv:1012.2882)
-
Edward Witten, Fivebranes and Knots (arXiv:1101.3216)
-
Anajak Phonchantuek, Pichet Vanichchapongjaroen: Double dimensional reduction of M5-brane action in Sen formalism, Eur. Phys. J. C 83 (2023) 721 [arXiv:2305.04861, doi:10.1140/epjc/s10052-023-11892-2]
Reduction of black M2s and black M5s
The differential geometry of the double dimensional reduction of the M2-brane- and M5-brane-charges was maybe first clearly written out in:
- Varghese Mathai, Hisham Sati, §4 in: Some Relations between Twisted K-theory and Gauge Theory, J. High Energ. Phys. 2004 03 (2004) 016 [arXiv:hep-th/0312033, doi:10.1088/1126-6708/2004/03/016]
For their black brane-reductions see:
- José Figueroa-O'Farrill, Joan Simón, Supersymmetric Kaluza-Klein reductions of M2 and M5-branes, Adv. Theor. Math. Phys. 6:703-793, 2003 (arXiv:hep-th/0208107)
Reduction of M-Waves and MK6s
- José Figueroa-O'Farrill, Joan Simón, Supersymmetric Kaluza-Klein reductions of M-waves and MKK-monopoles, Class. Quant. Grav.19:6147-6174, 2002 (arXiv:hep-th/0208108)
Last revised on August 12, 2025 at 07:03:29. See the history of this page for a list of all contributions to it.
 (
(