nLab orbifold

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Discuss this page |
Redirected from "effective orbifold".

Context

Higher Geometry

higher geometry / derived geometry

Ingredients

Concepts

Constructions

Examples

Theorems

Higher Lie theory

∞-Lie theory (higher geometry)

Background

Smooth structure

Higher groupoids

Lie theory

∞-Lie groupoids

∞-Lie algebroids

Formal Lie groupoids

Cohomology

Homotopy

Related topics

Examples

∞\infty-Lie groupoids

∞\infty-Lie groups

∞\infty-Lie algebroids

∞\infty-Lie algebras

Contents

Idea

An orbifold is much like a smooth manifold but possibly with singularities of the form of fixed points of finite group-actions.

(from Hyde-Ramsden-Robins 14)

Where a smooth manifold is a space locally modeled on Cartesian spaces/Euclidean spaces ℝ n\mathbb{R}^n, an orbifold is, more generally, a space that is locally modeled on quotients of ℝ n\mathbb{R}^ns, suitably understood, by actions of finite groups of diffeomorphisms. Here fixed points of the group action become singular points in the quotient, like the tip of a cone, which are not allowed in mere manifolds:

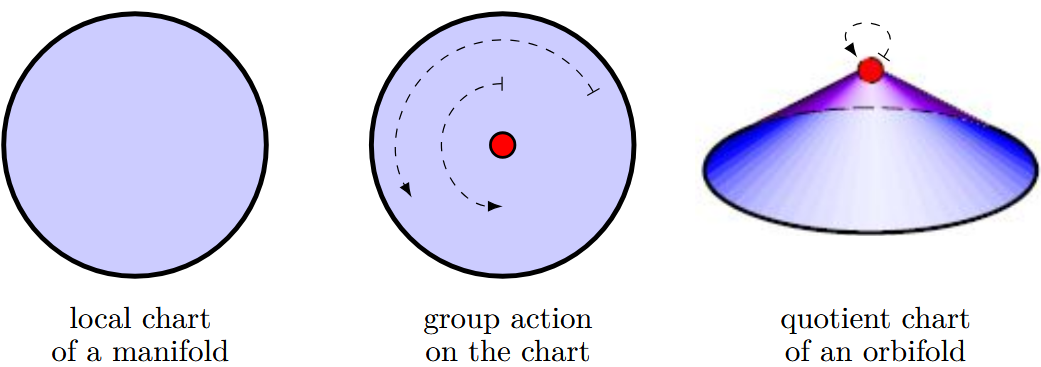
One way to make precise the nature of these quotients is as stacky homotopy quotients, hence as smooth action groupoids ℝ n⫽G\mathbb{R}^n\sslash G. The global structure of an orbifold is then captured (Moerdijk & Pronk 1997, Moerdijk 2002) by saying that orbifolds are proper étale Lie groupoids, and with Morita equivalent Lie groupoids understood to give the same orbifold this means that orbifolds are (étale) smooth stacks.

The word orbifold was introduced by Thurston 1992, while the concept goes back to Satake 1956, who originally spoke of VV-manifolds and took them in a more restrictive sense, assuming that the actions of finite groups on the charts are always effective. Nowadays these are called effective orbifolds.

There is also a notion of finite stabilizers in algebraic geometry. A singular variety is called an (algebraic) orbifold if it has only so-called orbifold singularities.


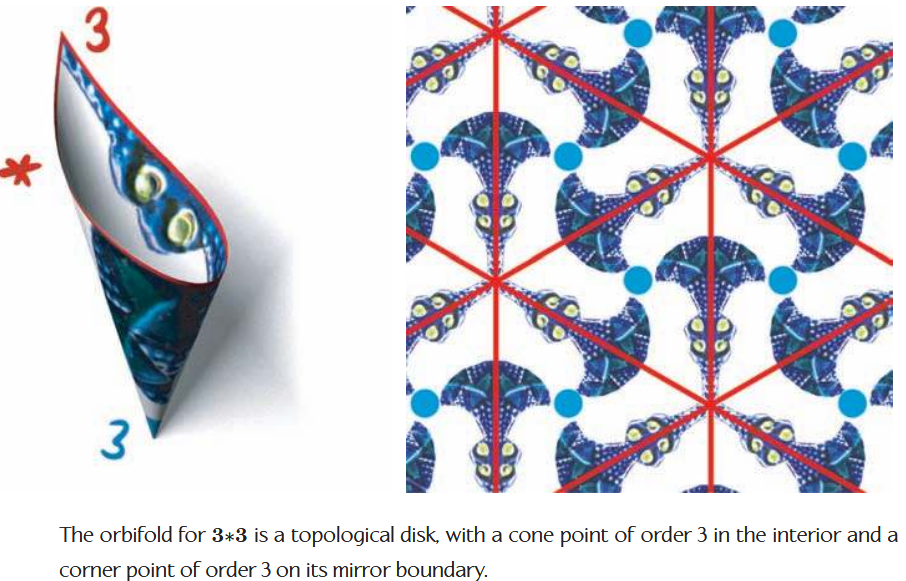
Artistic illustrations of 2-dimensional flat orbifolds (arising as quotients of the plane by wallpaper groups) are presented by Conway, Burgiel & Goodman-Strauss 2008:

(from CBG 2008 p. 114)


(from CBG 2008 p. 116)


Definition

Via orbifold atlases

The traditional definition of orbifolds, going back to Satake 1956:

Definition

(effective orbifold structure via orbifold atlas)
Let XX be a paracompact Hausdorff space.

  1. An orbifold chart (G↷U^,ϕ)\big(G \curvearrowright \widehat{U}, \phi\big) for XX is:

    1. n∈ℕn \in \mathbb{N}, the dimension,

    2. U^⊂ℝ n\widehat U \subset \mathbb{R}^n, a connected open subset,

    3. G⊂Diff(U^)G \subset Diff(\widehat{U}), a finite group of diffeomorphisms of U^\widehat{U},

    4. ϕ:U^⟶X\phi : \widehat{U} \longrightarrow X, a GG-invariant continuous function such that:

      1. its image U≔ϕ(U^)⊂XU \coloneqq \phi\big(\widehat{U}\big) \subset X is an open subset,

      2. its quotient map U^/G⟶∼ϕ(U^)⊂X\widehat{U}/G \overset{\sim}{\longrightarrow} \phi\big(\widehat{U}\big) \subset X is a homeomorphism onto this image.

  2. An embedding

    (G 1↷U^ 1,ϕ 1)↪ι(G 2↷U^ 2,ϕ 2) \big(G_1 \curvearrowright \widehat{U}_1, \phi_1\big) \overset{\iota}{\hookrightarrow} \big(G_2 \curvearrowright \widehat{U}_2, \phi_2\big)

    between a pair of such orbifold charts is:

    • a smooth embedding ι:U^ 1↪U^ 2\iota \colon \widehat{U}_1 \hookrightarrow \widehat{U}_2

    such that:

    • ϕ 2∘ι=ϕ 1\phi_2 \circ \iota = \phi_1.

  3. An orbifold atlas for XX is:

    • a set {(G i↷U^ i,ϕ i)} i∈I\Big\{\big(G_i \curvearrowright \widehat{U}_i, \phi_i\big)\Big\}_{i \in I} of orbifold charts

    such that:

    1. the images {U i↪ϕX} i∈I\Big\{ U_i \xhookrightarrow{\phi} X \Big\}_{i \in I} form an open cover of XX,

    2. for all pairs of charts (G 1↷U^ 1,ϕ 1)\big(G_1 \curvearrowright \widehat{U}_1, \phi_1\big), (G 2↷U^ 2,ϕ 2)\big(G_2 \curvearrowright \widehat{U}_2, \phi_2\big) in the set, and all x∈U 1∩U 2x \in U_1 \cap U_2, there exists:

      1. an open neighbourhood U 12⊂U 1∩U 2U_{12} \subset U_1 \cap U_2 of xx,

      2. an orbifold chart (G 12↷U^ 12,ϕ 12)\big(G_{12} \curvearrowright \widehat{U}_{12}, \phi_{12}\big) of U 12U_{12},

      3. embeddings (G 12↷U^ 12,ϕ 12)↪(G i↷U^ i,ϕ i)\big(G_{12} \curvearrowright \widehat{U}_{12}, \phi_{12}\big) \hookrightarrow \big(G_{i} \curvearrowright \widehat{U}_{i}, \phi_{i}\big) for i∈{1,2}i \in \{1,2\}.

  4. An equivalence of a pair of such orbifold atlases is a third atlas which refines both via embeddings.

  5. An orbifold structure on XX is a choice of equivalence class of orbifold atlases on XX.

(cf. Moerdijk & Pronk 1999 §1.1)

Remark

The assumption in Def. that the orbi-chart groups GG be subgroups of the diffeomorphism group implies that their action on the chart is effective. One also speaks of effective orbifolds to distinguish this original definition from the more relaxed one where the chart actions are only required to be through any group homomorphism G⟶Diff(U^)G \longrightarrow Diff\big(\widehat{U}\big).

The above definition does not explicitly require that chart embeddings be equivariant, but this is implied (cf. MP99 §1.1.1(i)):

Lemma

For

ι i:(G 1↷U^ 1,ϕ 1)↪(G 2↷U^ 2,ϕ 2) \iota_i \,\colon\, \big(G_1 \curvearrowright \widehat{U}_1, \phi_1\big) \hookrightarrow \big(G_2 \curvearrowright \widehat{U}_2, \phi_2\big)

a pair, i∈{1,2}i \in \{1,2\}, of embeddings of orbifold charts (Def. ), there exists a unique g 2∈G 2g_2 \in G_2 such that

ι 2=g 2∘ι 1. \iota_2 = g_2 \circ \iota_1 \mathrlap{\,.}

Proof

By definition of chart embeddings, we have

ϕ 2∘ι 1=ϕ 1=ϕ 2∘ι 2. \phi_2 \circ \iota_1 = \phi_1 = \phi_2 \circ \iota_2 \mathrlap{\,.}

By the orbi-chart property of ϕ 2\phi_2, this means that for each point x∈U^ 1x \in \widehat{U}_1 there exists g 2(x)g_2(x) such that

ι 2(x)=g 2(x)∘ι 1(x), \iota_2(x) = g_2(x) \circ \iota_1(x) \,,

with U^⟶g 2G 2:x→g 2(x)\widehat{U} \overset{g_2}{\longrightarrow} G_2 \colon x \to g_2(x) a continuous function.

Finally, by the assumption that G 2G_2 is finite, hence discrete, and that U^ 1\widehat{U}_1 is connected, this function must be constant.

Proposition

Given an embedding of orbifold charts (Def. )

ι:(G 1↷U^ 1,ϕ 1)↪(G 2↷U^ 2,ϕ 2) \iota \,\colon\, \big(G_1 \curvearrowright \widehat{U}_1, \phi_1\big) \hookrightarrow \big(G_2 \curvearrowright \widehat{U}_2, \phi_2\big)

there exists a unique group homomorphism

ι:G 1⟶G 2 \iota \colon G_1 \longrightarrow G_2

such that for all g 1∈G 1g_1 \in G_1 and x∈U^ 1x \in \widehat{U}_1

(1)ι(g 1⋅x)=ι(g 1)⋅ι(x). \iota( g_1 \cdot x ) \;=\; \iota(g_1) \cdot \iota(x) \,.

Moreover, if the orbifold is effective (Rem. ), the this group homomorphism is injective.

Proof

Noting that with λ\lambda also λ∘g 1\lambda \circ g_1 is am embedding, Lem. implies a unique g 2g_2 with g 2∘λ=λ∘g 1g_2 \circ \lambda = \lambda \circ g_1. This gives the group homomorphism.

To see that this homomorphism is injective of the chart actions are effective group action, assume that g 1g_1 is such that that ι(g 1)=e\iota(g_1) = \mathrm{e}. We need to see that then already g 1=eg_1 = \mathrm{e}. But the equivariance property (1) just proven states that, for all x∈U^ 1x \in \widehat{U}_1,

ι(g 1⋅x)=ι(x). \iota(g_1 \cdot x) = \iota(x) \,.

Since ι\iota is an embedding of smooth manifolds, this implies that g 1⋅x=xg_1 \cdot x = x for all x∈U^ 1x \in \widehat{U}_1, for all xx. By effectiveness, this implies that g 1=eg_1 = \mathrm{e}.

Remark

Every orbifold (Def. ) may be given by an orbi-atlas all whose orbi-charts (G,U^,ϕ)\big(G, \widehat{U}, \phi\big) are open balls U^≃𝔻 n⊂ℝ n\widehat{U} \simeq \mathbb{D}^n \subset \mathbb{R}^n equipped with an orthogonal linear group action.This follows by the existence of invariant Riemannian metrics and the differential version of the slice theorem,

(cf. MP99 §1.1.1(ii))

Via proper étale groupoids

Equivalently, an orbifold is a smooth stack presented by an orbifold groupoid [Moerdijk & Pronk 1997, 1999].

Definition

An orbifold is a Lie groupoid 𝒢\mathcal{G} which is

  1. étale:

    the source map s:Mor(𝒢)⟶Obj(𝒢)s \colon Mor(\mathcal{G}) \longrightarrow Obj(\mathcal{G}) is a local diffeomorphisms (as are then all the other structure maps),

  2. proper:

    the pair of the source and target maps (s,t):Mor(𝒢)⟶Obj(𝒢)(s,t) \colon Mor(\mathcal{G}) \longrightarrow Obj(\mathcal{G}) is a proper map.

Remark

From the perspective of the traditional Definition one may rather want to say that (cf. Moerdijk 2002 §3.2): An orbifold structure on a paracompact Hausdorff space XX is a choice of Lie groupoid 𝒢\mathcal{G} as in Def. , together with a homeomorphism from its space of isomorphism classes, Obj(𝒢)/Mor(𝒢)⟶∼XObj(\mathcal{G})/\mathrm{Mor}(\mathcal{G}) \overset{\sim}{\longrightarrow} X.

But since XX is determined, up to homeomorphism, from the groupoid 𝒢\mathcal{G}, the evident category of orbifold structures in this sense is equivalent to the homotopy category of the corresponding Lie groupoids/differentiable stacks (cf. Moerdijk 2002 §3.3)

Remark

The main ingredient in the construction (cf. Amenta 2012, Cor. 2.4.13) of an orbifold atlas (Def. ) from an effective proper étale Lie groupoid (Def. ) is the fact that every point xx in the object space of a proper étale Lie groupoid has an open neighbourhood U⊂Obj(𝒢)U \subset Obj(\mathcal{G}) on which the groupoid structure restricts to an action groupoid U⫽G xU \sslash G_x of the finite automorphism group/isotropy group G x≔Hom 𝒢(x,x)G_x \coloneqq Hom_{\mathcal{G}}(x,x) of xx. (cf. Moerdijk & Pronk 1997, pp. 15, Amenta 2012, Thm. 2.4.11)

Conversely, the construction of a proper étale Lie groupoid (Def. ) from an orbifold atlas (Def. ) is indicated in Moerdijk & Pronk 1999, inside proof of Thm. 4.1.1.

Properties

General

Global quotient orbifolds

In (ALR 07, theorem 1.23) it is asserted that every effective orbifold XX (paracompact, Hausdorff) is isomorphic to a global quotient orbifold, specifically to a global quotient of O(n)O(n) (where nn is the dimension of XX) acting on the frame bundle of XX (which is a manifold).

(Co)homology

It has been noticed that the topological invariants of the underlying topological space of an orbifold as a topological space with an orbifold structure are not appropriate, but have to be corrected leading to orbifold Euler characteristics, orbifold cohomology etc. One of the constructions which is useful in this respect is the inertia orbifold (the inertia stack of the original orbifold) which gives rise to “twisted sectors” in Hilbert space of a quantum field theory on the orbifold, and also to twisted sectors in the appropriate cohomology spaces. A further generalization gives multitwisted sectors.

Examples

Example

(sign involution orbifold of the nn-torus)
A basic class of examples of closed flat orbifolds are the orbifold quotients 𝕋 n⫽C 2\mathbb{T}^n \sslash C_2 of the n n -torus 𝕋 n≔ℝ n/ℤ n\mathbb{T}^n \,\coloneqq\, \mathbb{R}^n/\mathbb{Z}^n by the involution-action inverting the sign of the canonical coordinates:

Further examples:

Orbifolds are in differential geometry what Deligne-Mumford stacks are in algebraic geometry. See also at geometric invariant theory and GIT-stable point.

Orbifolds may be regarded as a kind of stratified spaces.

See also:

Orbifolds in string theory:

References

General

Essentially the modern definition of orbifolds via orbifold atlases was first given by:

under the name “V-manifolds”. (Satake’s motivation for this terminology has not survived. One claim [MO:q/97901] is that: According to a colleague who asked Satake about it, the “V” in “V-manifold” stands for the German word ‘Verzweigung, which means “branching” or “ramification”, as in ramified cover.)

The term “orbifold” was introduced by:

the first three chapters of which are published in expanded form as:

Further early discussion:

and specifically for orbifolds in complex geometry:

For careful comparative review of the definitions in these original articles see also IKZ 10.

Survey of basic orbifold theory:

See also:

  • Wikipedia: Orbifolds

  • Alanod Sibih: Orbifold Atlas Groupoids, PhD thesis, Dalhousie (2013) [pdf]

On good orbifolds:

Textbook accounts:

Application to moduli spaces of curves and moduli spaces of Riemann surfaces:

On Riemannian orbifolds:

  • Christian Lange, Orbifolds from a metric viewpoint (arXiv:1801.03472)

  • Renato G. Bettiol, Andrzej Derdzinski, Paolo Piccione, Teichmüller theory and collapse of flat manifolds, Annali di Matematica (2018) 197: 1247 (arXiv:1705.08431, doi:10.1007/s10231-017-0723-7)

  • S. T. Hyde, S. J. Ramsden and V. Robins, Unification and classification of two-dimensional crystalline patterns using orbifolds, Acta Cryst. (2014). A70, 319-337 (doi:10.1107/S205327331400549X)

Assorted applications in mathematical physics and notably in string theory:

Orbifolds often appear as moduli spaces in differential geometry:

The generalization of orbifolds to weighted branched manifolds:

On orbifolds, orbifold cohomology and specifically on Chen-Ruan cohomology and orbifold K-theory:

As proper étale Lie groupoids

The understanding of orbifolds as proper étale Lie groupoids/differentiable stacks is due to

See also:

Review:

  • Olivier Brahic: Orbifolds via Lie Groupoids (2009) [pdf]

and further details:

  • Alexander Amenta: The Geometry of Orbifolds via Lie Groupoids, Honours Thesis, Australian National University (2012) [arXiv:1309.6367]

Analogous discussion for topological orbifolds as topological stacks:

Discussion of the corresponding perspective in algebraic geometry, via Deligne-Mumford stacks:

  • Andrew Kresch, On the geometry of Deligne-Mumford stacks (doi:10.5167/uzh-21342, pdf), in: D. Abramovich, A. Bertram, L. Katzarkov, R. Pandharipande, M. Thaddeus (eds.) Algebraic Geometry: Seattle 2005, Proceedings of Symposia in Pure Mathematics 80, American Mathematical Society (2009) 259-271 [pspum-80-1]

On mapping stacks of orbifolds:

Discussion of principal bundles and fiber bundles over orbifolds:

An expected relation of orbifolds (orbispaces) to global equivariant homotopy theory:

As diffeological spaces

On orbifolds regarded as naive local quotient spaces (instead of homotopy quotients/Lie groupoids/differentiable stacks) but as such formed in diffeological spaces:

and as stratified diffeological spaces:

On this approach seen in the broader context of cohesive higher differential geometry:

Orbifold cobordism

Orbifold cobordisms are discussed in

  • K. S. Druschel, Oriented Orbifold Cobordism, Pacific J. Math., 164(2) (1994), 299-319 (doi:10.2140/pjm.1994.164.299, pdf)

  • K. S. Druschel, The Cobordism of Oriented Three Dimensional Orbifolds, Pacific J. Math., bf 193(1) (2000), 45-55.

  • Andres Angel, Orbifold cobordism (pdf)

See also at orbifold cobordism.

tangential structure on orbifolds (in the context of factorization homology):

In string theory

(from Green 86)

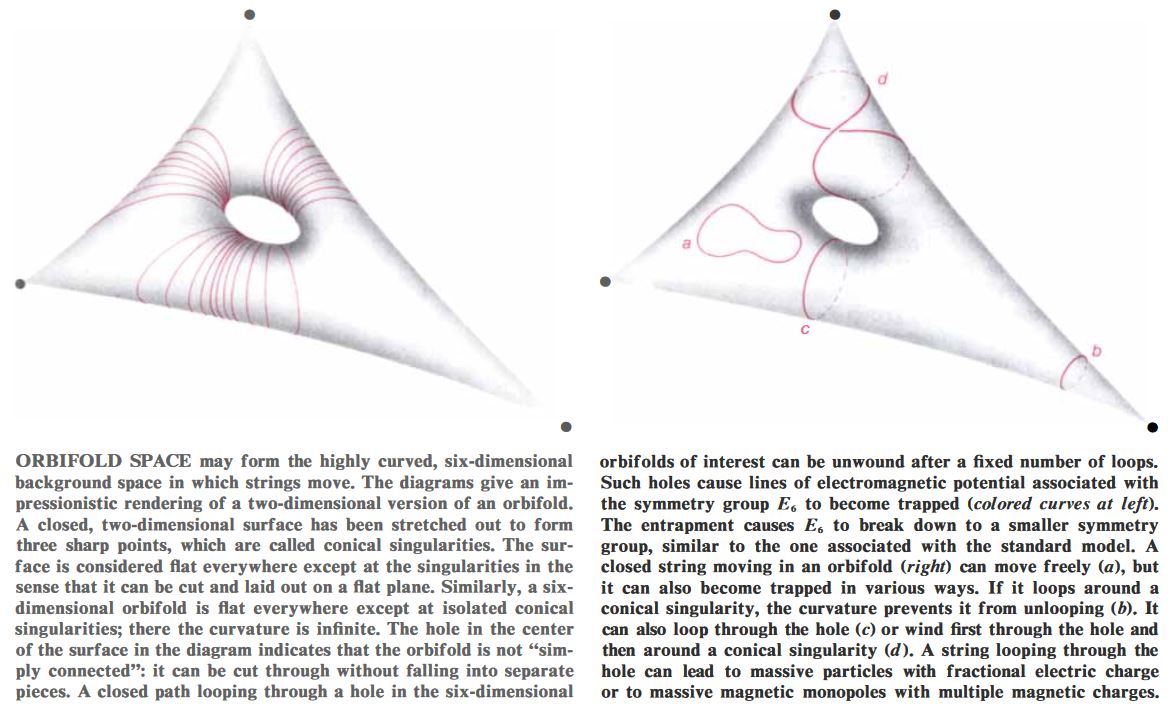
In perturbative string theory, orbifolds as target spaces for a string sigma-model were first considered in

and then further developed notably in

See also:

For more references on orbifolds in string theory see also at

Review of heterotic string phenomenology on orbifolds:

Discussion of blow-up of orbifold singularities in string theory:

In terms of vertex operator algebras:

Review of orbifolds in the context of string KK-compactifications and intersecting D-brane models:

and for orbifolds of G₂-manifolds for M-theory on G₂-manifolds:

and for heterotic string phenomenology:

For topological strings the path integral as a pull-push transform for target orbifolds – in analogy to what Gromov-Witten theory is for Deligne-Mumford stacks – has first been considered in

Review with further pointers:

On non-supersymmetric flat orbifolds of supergravity theories:

  • Anamaria Font, Alexis Hernandez, Non-Supersymmetric Orbifolds, Nucl. Phys. B 634 (2002) 51-70 [arXiv:hep-th/0202057]

and specifically fluxed KK-compactification of D=6 supergravity on the pillowcase orbifold:

On supergravity KK-compactified (and branes wrapped on) spindle orbifolds:

On orbifolds by 2-groups in view of sigma-models inspired from string theory:

In condensed matter theory

In solid state physics, the effective Brillouin torus of quasi-momenta of electrons in a crystalline material is generally an orbifold, namely the quotient orbifold of Euclidean space (momentum space) by the corresponding crystallographic group.

Explicit discussion of such crystallographic orbifolds for the purpose of crystallography:

In the physics literature this orbifold nature of the Brillouin torus is not often made explicit, but see articles in the context of crystalline topological phases of matter:

category: Lie theory

Last revised on January 18, 2026 at 16:27:18. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (127 revisions) Cite Print Source