nLab braid group statistics

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Discuss this page |
Contents

Context

Topological physics

Contents

Anyon braiding

In quantum physics, braid group statistics or anyon statistics (sometimes: plektons) refers to an exotic phenomenon where the braiding of the worldlines of certain effective particles (“anyons”) in an effectively 2+1-dimensional spacetime has the effect of transforming the quantum state of the total quantum system by unitary operators which constitute a linear representation of the braid group – a braid representation.

Often this is motivated as a generalization of the boson- or fermion-“statistics” which enters the spin-statistics theorem, see below.

But the actual mathematical nature of anyons must be different from that of elements of boson/fermion-Fock spaces and often remains somewhat vague in existing discussions. One may recognize two different more concrete conceptualizations of anyons in the literature:

  1. anyonic quanta much like boson/fermion quanta but subject to an additional global interaction by Aharonov-Bohm phases due to a flat fictitious gauge field which is sourced by and coupled to each of the quanta;

    (this goes back to Arovas, Schrieffer, Wilczek & Zee 1985, further developed in Chen, Wilczek, Witten & Halperin 1989, see below)

  2. anyonic defects like vortices or other solitons, whose position is a classical parameter to the quantum system, the adiabatic movement of which acts by Berry phases on the quantum ground state.

    (e.g. Avros, Schrieffer & Wilczek 1984, see further below)

The concept of anyons is particularly well motivated in solid state physics, where effectively 2-dimensional quantum materials are common place (eg. graphene) or where particles may otherwise be constrained to move in a plane, such as in the quantum Hall effect. There is a multitude of models in condensed matter theory (mostly lattice models, such as string-net models) which theoretically realize anyon braid group statistics, and there are some first experimental indications of anyonic phenomena in actual materials (see the references under Experimental Realization) below.

Specifically, in the context of topological phases of matter, the (potential) presence of anyons has come to be known as the case of topological order, see there for more.

Besides general curiosity, much of the interest in anyonic braid group statistics lies in the fact that these braid representations are imagined to potentially serve as quantum gates in topological quantum computers. See there for more.

As generalized boson/fermion statistics?

In quantum field theory, one speaks of the “statistics” of a particle species when referring to the linear representation that nn-particle wavefunctions form under the the symmetric group Sym(n)Sym(n) which permutes the nn particles.

While there is a rich representation theory of the symmetric group, the spin-statistics theorem says, when it applies, that for field quanta only the simplest two possibilities may occur:

Now, the braid group on nn strands covers the symmetric group

Br(n)↠Sym(n) Br(n) \twoheadrightarrow Sym(n)

which allows one to regard any linear representation of the symmetric group also as a particular braid group representation. But by its definition, the braid group may be understood as the group of isotopy-classes of disjoint timelike worldlines in an effectively 2+1 dimensional spacetime, with the group operation being concatenation of worldlines.

In this sense, one may imagine that any braid group representations may generalize the boson/fermion statistics in 2+1 dimensions. Texts typically suggest that this applies to quasiparticles.

The term anyon (due to Wilczek 1982b) is a pun on this state of affairs that any statistics “in between” boson- and fermion-statistics may be allowed.

On the other hand, anyonic braiding is conceptually different from boson/fermion statistics – if it were on the same footing then the spin-statistics theorem would rule out anyonic braiding. This is acknowledged by Chen, Wilczek, Witten & Halperin 1989, p. 352 (cf. also Wilczek 1990, §I.2, pp. 11):

Once the permutation group is replaced by the braid group, the simple construction of passing from the solution to the one-particle problems to the solution of many-particle problems, familiar from the free bosons and free fermions, does not work anymore.


Braiding of anyonic quanta – via “fictitious” AB-phases

A concrete model for anyonic quanta via otherwise free fermions in 2d interacting through a flat “fictitious gauge field” was proposed in Arovas, Schrieffer, Wilczek & Zee 1985 and developed in Chen, Wilczek, Witten & Halperin 1989 and Iengo & Lechner 1992 (the model has been advertized in early reviews, e.g. Wilczek 1990, §I.3 and Wilczek 1991, but seems not to have been developed much since):

This model regards anyons as a priori free fermions, but equipped now with a non-local mutual interaction via a “fictitious gauge field” (CWWH89, §2), in that each of the particles is modeled as the singular source of a flat circle connection (a vector potential with vanishing field strength), which hence exerts no Lorentz force but has the effect that globally each other particle is subject to the same Aharonov-Bohm effect as would be caused by a tuple of infinite solenoids piercing through each of the other particle’s positions.

For emphasis, from CWWH89, p. 359:

Here the particles are to be regarded (in the absence of interactions) as fermions; the interaction then makes them anyons with statistical parameter θ=π(1−1/n)\theta = \pi(1 - 1/n).

It follows (Wu 1984, Imbo, Imbo & Sudarshan 1990) that (quoting from Fröhlich, Gabbiani & Marchetti 1990, p. 20):

If θ∈/12ℤ\theta \in\!\!\!\!\!/ \frac{1}{2}\mathbb{Z} the Hilbert space of anyon wave functions must be chosen to be a space of multi-valued functions with half-monodromies given by the phase factors exp(2πiθ)exp(2 \pi \mathrm{i} \theta). Such wave functions can be viewed as single-valued functions on the universal cover M˜ n\widetilde M_n of M nM_n [[the configuration space of points]].

Further discussion of anyon-wavefunctions as multi-valued functions on a configuration space of points, hence equivariant functions on its universal cover: BCMS93, §1, Mund & Schrader 1995, DFT97, §1 Myrheim 1999, DMV03, Murthy & Shankar 2009

Incidentally, the quasi-particle-excitations of (or in) a gas of such Aharonov-Bohm phased anyons are argued to be vortices (CWWH89, p. 457):

we are led to conclude that in anyon superconductivity, charged quasi-particles and vortices do not constitute two separate sorts of elementary excitations - they are one and the same.

This seamlessly leads over to:


Braiding of anyonic defects – via adiabatic Berry phases

In practice, many (most?) incarnations of the concept of anyons are anyonic defects – non-perturbative solitonic defects (of codimension=2), akin to vortices in fluids:

Anyonic particles are best viewed as a kind of topological defects that reveal non-trivial properties of the ground state. [[Kitaev 2006, p. 4]]

Anyons can arise in two ways: as localised excitations of an interacting quantum Hamiltonian or as defects in an ordered system. [[Das Sarma, Freedman & Nayak 2015, p. 1]]

(Compare also the original discussions in Goldin, Menikoff & Sharp 1981, §III, Wilczek 1982a & Wilczek 1990, p. 5, which offer a quantum particle “bound” to a classical & infinite solenoid – hence a 2d magnetic monopole defect – as a decent model for an anyon.)

But defects are a kind of boundary conditions, hence external parameters or background fields for the actual quantum field.

Concretely, a widely appreciated proposal (Moore & Read 1991, Read & Rezayi 1999) identifies anyonic ground state wavefunctions with conformal blocks of a 2d CFT – hence with Chern-Simons theory states – with prescribed poles at the location of the anyons.

Now the quantum adiabatic theorem says that the sufficiently slow motion of such external parameters transforms the quantum ground state by unitary operators (“Berry phases”, see also at adiabatic quantum computation). This suggests (Avros, Schrieffer & Wilczek 1984, p. 1, Freedman, Kitaev, Larsen & Wang 2003, pp. 6, Nayak, Simon, Stern & Freedman 2008, §II.A.2 (p. 6), Cheng, Galitski & Das Sarma 2011, p. 1) that:

Definition

(adiabatic defect braiding)
Anyon braiding statistics is the braid group representation on a quantum ground state induced by adiabatic braiding of topological codimension=2 defects in their configuration space.

Here

  1. for point-defects the “configuration space” is the configuration space of points in a surface (as briefly touched upon already in Leinaas & Myrheim 1977, pp. 22, Wilczek 1982b, p. 959), such as in the plane, in which case its fundamental group is the braid group;

  2. “topological” is meant as in topological quantum field theory: The induced adiabatic unitary transformation is demanded/assumed to depend only on the isotopy-class of the defect-worldlines, hence only on the underlying braid-pattern.

This notion of anyon “statistics” is at least tacitly implicit in much of the literature on anyons in topological quantum computation, such as in the popular graphics depicting anyon worldlines as the Wilson lines in Chern-Simons theory. (see the graphics below).

Indeed, the effects of adiabatic braiding of defects in quantum materials has been understood and discussed before and in parallel to the term “anyon” becoming established: Mermin 1979, Lo & Preskill 1993.

A concrete realistic example of defect anyons are vortex anyons see below. But the notion of codimension=2 defects subsumes situations that are quite different from the quasiparticle-excitations imagined in traditional texts on anyons, such as:

Vortex anyons

Specifically, vortex anyons are realized in Bose-Einstein condensates (MPSS19, following PFCZ01) and in (other) superfluids (MMN21).

In fact, defect-type vortex anyons generically appear in condensates of non-defect anyons (CDLR19):

From CDLR19

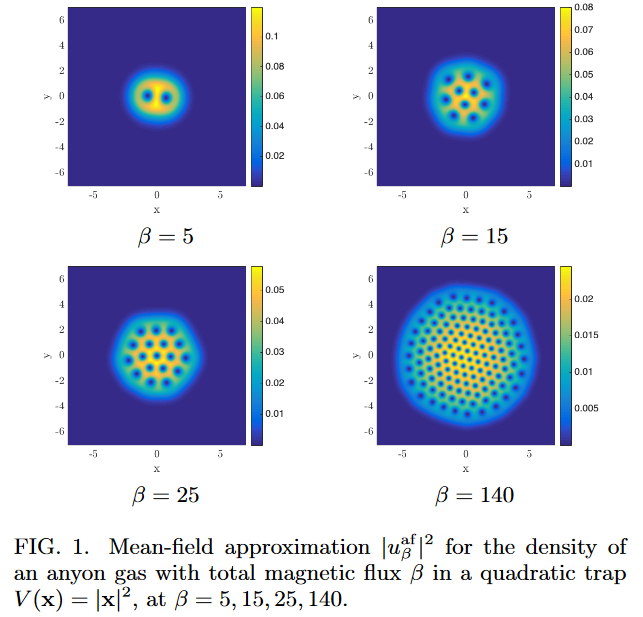
A theoretical model of vortex anyons in a Higgs field coupled to Chern-Simons theory is discussed in Fröhlich & Marchetti 1988. An instructive lattice model of vortex anyons is analyzed in detail in Kitaev 2006.

Much attention in current efforts towards realizing topological quantum computation is being paid to anyons realized as Majorana zero modes bound to vortices (Das Sarma, Freedman & Nayak 2015, cf. MMBDRSC19).

This situation may generalize to parafermion-su(2)-anyons, where

each (anti)soliton carries parafermion zero mode which supplies it with the non-Abelian statistics [[Tsvelik 2014a, p. 2, cf. Borcherding 2018, pp. 3. ]]

Braiding of nodal points in momentum space (graphics from MMBDRSC19, Fig. 1):

Anyonic band nodes?

Around 2020 the view has been emerging that also defects “in momentum/reciprocal space” may behave as anyonic defects under braiding in momentum space. This applies concretely to nodal points (where electron bands touch or cross) in the momentum space Brillouin torus of topological semi-metals:

here are band crossing points, henceforth called vortices [[Ahnm Park & Yang 2019]]

a new type non-Abelian “braiding” of nodal-line rings inside the momentum space [[Tiwari & Bzdušek 2020]]

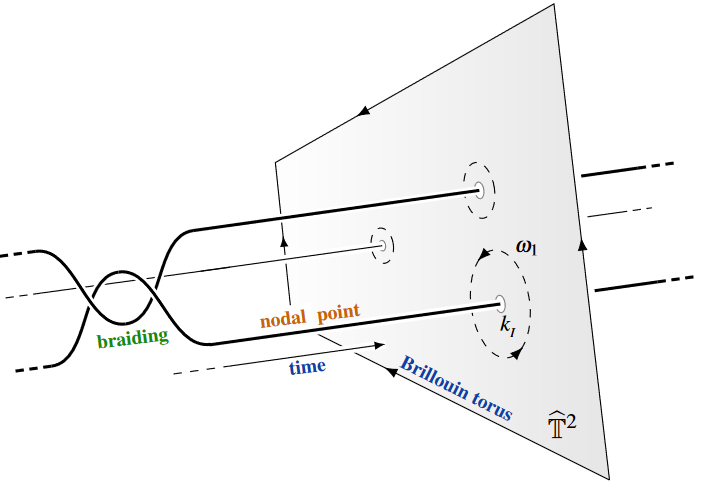
(graphics from SS22)

Curiously, these reciprocal/momemtum space anyons lend themselves to tractable laboratory manipulation in a way that has remained notoriously elusive for “position space” anyons:

Our work opens up routes to readily manipulate Weyl nodes using only slight external parameter changes, paving the way for the practical realization of reciprocal space braiding [[CBSM22]],

specifically

it is possible to controllably braid Kagome band nodes in monolayer Si 2O 3\mathrm{Si}_2 \mathrm{O}_3 using strain and/or an external electric field [[PBMS22]],

leading to:

new opportunities for exploring non-Abelian braiding of band crossing points (nodes) in reciprocal space, providing an alternative to the real space braiding exploited by other strategies.

Real space braiding is practically constrained to boundary states, which has made experimental observation and manipulation difficult; instead, reciprocal space braiding occurs in the bulk states of the band structures and we demonstrate in this work that this provides a straightforward platform for non-Abelian braiding. [[PBSM22]].

Defect branes

In string theory, defect branes are D-branes or M-branes of codimension=2, such as D7-branes in type IIB string theory or M5-brane-intersections on “M3-branes” in M-theory.

It has been suggest in deBoer & Shigemori 2012, p. 65 that these could behave like anyons. This is further substantiated in SS22. See there for more.


References

General

The concept of anyons satisfying braid group statistics originated independently in:

The term anyon was introduced in:

Identification of anyon phases (specifically in the quantum Hall effect) as Berry phases of an adiabatic transport of anyon positions:

The “fictitious gauge field”-method for modelling anyons:

and with specific emphasis on the resulting (abelian!) Chern-Simons theory:

The suggestion that the anyonic ground state-wavefunctions are essentially conformal blocks of 2d CFT (notably for su(2)-anyons):

More comprehensive accounts of anyons:

See also:

On the history of the concept:

Rigorous discussion in terms of superselection sectors in algebraic quantum field theory:

Discussion of anyon-wavefunctions as multi-valued functions on a configuration space of points:

The topic of quantum measurement of non-abelian anyons is crucial to their identification in experiment but has received little attenion, exceptions being:

Anyonic topological order in terms of braided fusion categories

Claim and status

In condensed matter theory it is folklore that species of anyonic topological order correspond to braided unitary fusion categories/modular tensor categories.

The origin of the claim is:

Early accounts re-stating this claim (without attribution):

Further discussion (mostly review and mostly without attribution):

Emphasis that the expected description of anyons by braided fusion categories had remained folklore, together with a list of minimal assumptions that would need to be shown:

An argument that the statement at least for SU(2)-anyons does follow from an enhancement of the K-theory classification of topological phases of matter to interacting topological order:

In string/M-theory

Arguments realizing such anyonic topological order in the worldvolume-field theory on M5-branes:

Via KK-compactification on closed 3-manifolds (Seifert manifolds) analogous to the 3d-3d correspondence (which instead uses hyperbolic 3-manifolds):

Via 3-brane defects:

Further discussion

Relation to ZX-calculus:

On detection of topological order by observing modular transformations on the ground state:

See also:

Anyons in quantum Hall liquids

References on anyon-excitations (satisfying braid group statistics) in the quantum Hall effect (for more on the application to topological quantum computation see the references there):

The prediction of abelian anyon-excitations in the quantum Hall effect (i.e. satisfying braid group statistics in 1-dimensional linear representations of the braid group):

The original discussion of non-abelian anyon-excitations in the quantum Hall effect (i.e. satisfying braid group statistics in higher dimensional linear representations of the braid group, related to modular tensor categories):

Review:

Claims of experimental observation:

Anyons in topological superconductors

On anyon-excitations in topological superconductors.

via Majorana zero modes:

Original proposal:

  • Nicholas Read, Dmitry Green, Paired states of fermions in two dimensions with breaking of parity and time-reversal symmetries, and the fractional quantum Hall effect, Phys. Rev. B61:10267, 2000 (arXiv:cond-mat/9906453)

Review:

  • Sankar Das Sarma, Michael Freedman, Chetan Nayak, Majorana Zero Modes and Topological Quantum Computation, npj Quantum Information 1, 15001 (2015) (nature:npjqi20151)

  • Nur R. Ayukaryana, Mohammad H. Fauzi, Eddwi H. Hasdeo, The quest and hope of Majorana zero modes in topological superconductor for fault-tolerant quantum computing: an introductory overview (arXiv:2009.07764)

  • Yusuke Masaki, Takeshi Mizushima, Muneto Nitta, Non-Abelian Anyons and Non-Abelian Vortices in Topological Superconductors [arXiv:2301.11614]

Further developments:

via Majorana zero modes restricted to edges of topological insulators:

  • Biao Lian, Xiao-Qi Sun, Abolhassan Vaezi, Xiao-Liang Qi, and Shou-Cheng Zhang, Topological quantum computation based on chiral Majorana fermions, PNAS October 23, 2018 115 (43) 10938-10942; first published October 8, 2018 (doi:10.1073/pnas.1810003115)

See also:

  • Yusuke Masaki, Takeshi Mizushima, Muneto Nitta, Non-Abelian Anyons and Non-Abelian Vortices in Topological Superconductors [[arXiv:2301.11614]]

Experimental detection of anyons

While the occurrence of anyon-excitations in the fractional quantum Hall effect is a robust theoretical prediction (see the references above), and while the fractional quantum Hall effect itself has long been established in experiment, the actual observation of anyons in these systems is subtle:

An early claim of the observation of non-abelian anyons seems to remain unconfirmed:

  • Sanghun An, P. Jiang, H. Choi, W. Kang, S. H. Simon, L. N. Pfeiffer, K. W. West, K. W. Baldwin, Braiding of Abelian and Non-Abelian Anyons in the Fractional Quantum Hall Effect (arXiv:1112.3400)

The claimed observation of abelian anyons is more securely established:

Defect anyons

Often the concept of anyons is introduced as if a generalization of perturbative quanta like fundamental bosons and fermions. But many (concepts of) types of anyons are really solitonic\;defects such as vortices.

The general concept of braiding of defects in solid state physics:

and more specifically for vortices:

Explicit discussion in terms of anyons:

  • Alexei Kitaev, Anyons in an exactly solved model and beyond, Annals of Physics 321 1 (2006) 2-111 [[doi:10.1016/j.aop.2005.10.005]]

    Anyonic particles are best viewed as a kind of topological defects that reveal nontrivial properties of the ground state. [[p. 4]]

Concrete vortex\;anyons in Bose-Einstein condensates:

and in (other) superfluids:

  • Yusuke Masaki, Takeshi Mizushima, Muneto Nitta, Non-Abelian Half-Quantum Vortices in 3P2 Topological Superfluids [[arXiv:2107.02448]]

and in condensates of non-defect anyons:

See also Ahn, Park & Yang 19 who refer to the band nodes in the Brillouin torus of a semi-metal as “vortices in momentum space”.

And see at defect brane.

Anyons in momentum-space

On non-trivial braiding of nodal points in the Brillouin torus of topological semi-metals (“braiding in momentum space”):

“a new type non-Abelian ‘braiding’ of nodal-line rings inside the momentum space”

“Here we report that Weyl points in three-dimensional (3D) systems with 𝒞 2𝒯\mathcal{C}_2\mathcal{T} symmetry carry non-Abelian topological charges. These charges are transformed via non-trivial phase factors that arise upon braiding the nodes inside the reciprocal momentum space.”

Braiding of Dirac points in twisted bilayer graphene:

Here, we consider an exotic type of topological phases beyond the above paradigms that, instead, depend on topological charge conversion processes when band nodes are braided with respect to each other in momentum space or recombined over the Brillouin zone. The braiding of band nodes is in some sense the reciprocal space analog of the non-Abelian braiding of particles in real space.

......

we experimentally observe non-Abelian topological semimetals and their evolutions using acoustic Bloch bands in kagome acoustic metamaterials. By tuning the geometry of the metamaterials, we experimentally confirm the creation, annihilation, moving, merging and splitting of the topological band nodes in multiple bandgaps and the associated non-Abelian topological phase transitions

new opportunities for exploring non-Abelian braiding of band crossing points (nodes) in reciprocal space, providing an alternative to the real space braiding exploited by other strategies.

Real space braiding is practically constrained to boundary states, which has made experimental observation and manipulation difficult; instead, reciprocal space braiding occurs in the bulk states of the band structures and we demonstrate in this work that this provides a straightforward platform for non-Abelian braiding.

See also:

  • Robert-Jan Slager, Adrien Bouhon, Fatma Nur Ünal, Floquet multi-gap topology: Non-Abelian braiding and anomalous Dirac string phase, Nature Communications 15 1144 (2024) [arXiv:2208.12824, doi:10.1038/s41467-024-45302-2]

  • Huahui Qiu et al., Minimal non-abelian nodal braiding in ideal metamaterials, Nature Communications 14 1261 (2023) [doi:10.1038/s41467-023-36952-9]

  • Wojciech J. Jankowski, Mohammedreza Noormandipour, Adrien Bouhon, Robert-Jan Slager, Disorder-induced topological quantum phase transitions in Euler semimetals [arXiv:2306.13084]

  • Seung Hun Lee, Yuting Qian, Bohm-Jung Yang, Euler band topology in spin-orbit coupled magnetic systems [arXiv:2404.16383]

    “Based on first-principles calculations, we report that such nodal point braiding in 2D electronic bands can be realized in a MSWI candidate, the bilayer ZrTe 5ZrTe_5 with in-plane ferromagnetism under pressure. […] one can expect that the braiding of nodes can be achieved in 2D bilayer ZrTe 5ZrTe_5 under the influence of an external in-plane Zeeman field.”


Incidentally, references indicating that the required toroidal (or yet higher genus) geometry for anyonic topological order in position space is dubious (as opposed to the evident toroidal geometry of the momentum-space Brillouin torus): Lan 19, p. 1, ….

Knotted nodal lines in 3d semimetals

Beware that various authors consider braids/knots formed by nodal lines in 3d semimetals, i.e. knotted nodal lines in 3 spatial dimensions, as opposed to worldlines (in 2+1 spacetime dimensions) of nodal points in effectively 2d semimetals needed for the anyon-braiding considered above.

An argument that these nodal lines in 3d space, nevertheless, may be controlled by Chern-Simons theory:

Topological quantum computation with anyons

The idea of topological quantum computation via a Chern-Simons theory with anyon braiding defects is due to:

and via a Dijkgraaf-Witten theory (like Chern-Simons theory but with discrete gauge group):

Monographs:

Review:

Focus on abelian anyons:

  • Jiannis K. Pachos, Quantum computation with abelian anyons on the honeycomb lattice, International Journal of Quantum Information 4 6 (2006) 947-954 [doi:10.1142/S0219749906002328, arXiv:quant-ph/0511273]

  • James Robin Wootton, Dissecting Topological Quantum Computation, PhD thesis, Leeds (2010) [ethesis:1163, pdf, pdf]

    “non-Abelian anyons are usually assumed to be better suited to the task. Here we challenge this view, demonstrating that Abelian anyon models have as much potential as some simple non-Abelian models. […] Though universal non-Abelian models are admittedly the holy grail of topological quantum computation, and rightly so, this thesis has shown that Abelian models are just as useful as non-universal non-Abelian models. […] Abelian models are a computationally powerful, fault-tolerant and experimentally realistic prospect for quantum computation.”

  • Seth Lloyd, Quantum computation with abelian anyons, Quantum Information Processing 1 1/2 (2002) [doi:10.1023/A:1019649101654, arXiv:quant-ph/0004010]

  • James R. Wootton, Jiannis K. Pachos: Universal Quantum Computation with Abelian Anyon Models, Electronic Notes in Theoretical Computer Science 270 2 (2011) 209-218 [doi:10.1016/j.entcs.2011.01.032, arXiv:0904.4373]

see also:

Realization in experiment (so far via quantum simulation of anyons on non-topological quantum hardware, cf. FF24, Fig 5, as in “topological codes” for quantum error correction):

on superconducting qbits:

on trapped-ion quantum hardware:

Discussion of anyon braid gates via homotopy type theory:

Braid group representations (as topological quantum gates)

On linear representations of braid groups (see also at braid group statistics and interpretation as quantum gates in topological quantum computation):

Review:

in relation to modular tensor categories:

  • Colleen Delaney, Lecture notes on modular tensor categories and braid group representations, 2019 (pdf, pdf)

Braid representations from the monodromy of the Knizhnik-Zamolodchikov connection on bundles of conformal blocks over configuration spaces of points:

and understood in terms of anyon statistics:

Braid representations seen inside the topological K-theory of the braid group‘s classifying space:

See also:

  • R. B. Zhang, Braid group representations arising from quantum supergroups with arbitrary qq and link polynomials, Journal of Mathematical Physics 33, 3918 (1992) (doi:10.1063/1.529840)

As quantum gates for topological quantum computation with anyons:

Introduction and review:

Realization of Fibonacci anyons on quasicrystal-states:

Realization on supersymmetric spin chains:

  • Indrajit Jana, Filippo Montorsi, Pramod Padmanabhan, Diego Trancanelli, Topological Quantum Computation on Supersymmetric Spin Chains [[arXiv:2209.03822]]

See also:


Compilation to braid gate circuits

On approximating (cf. the Solovay-Kitaev theorem) given quantum gates by (i.e. compiling them to) cicuits of anyon braid gates (generally considered for su(2)-anyons and here mostly for universal Fibonacci anyons, to some extent also for non-universal Majorana anyons):

Approximating all topological quantum gates by just the weaves among all braids:

Last revised on October 28, 2024 at 11:16:57. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (59 revisions) Cite Print Source